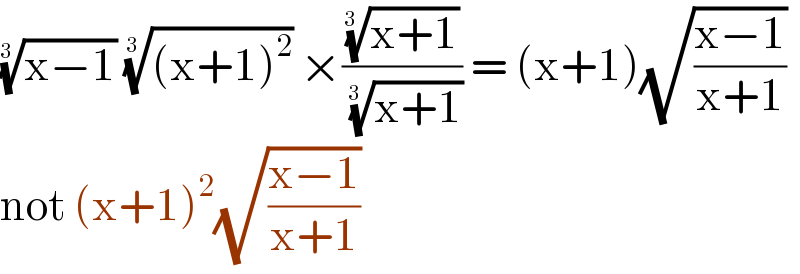bemath-dx-x-1-1-3-x-1-2-1-3-

Question Number 111195 by bemath last updated on 02/Sep/20

\sqrt{bemath}

\int \frac{dx}{\sqrt[3]{x - 1} \sqrt[3]{{(x + 1)}^{2}}} ?

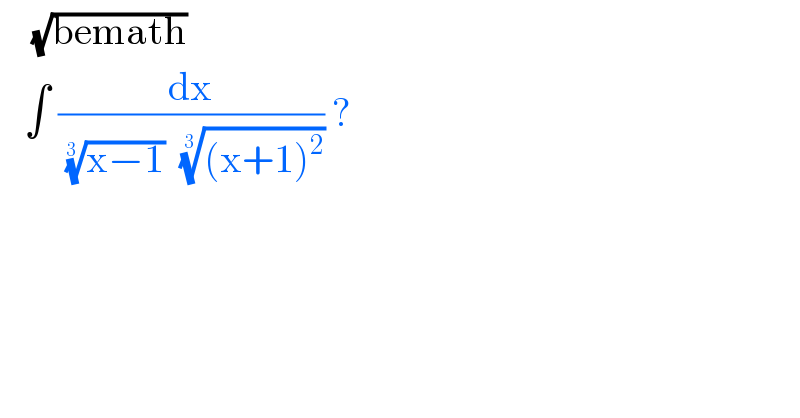
Answered by Sarah85 last updated on 02/Sep/20

substitute t = \sqrt[3]{\frac{x - 1}{x + 1}} \Leftrightarrow x = - \frac{t^{3} + 1}{t^{3} - 1} \Rightarrow

\Rightarrow d x = \frac{3}{2} \sqrt[3]{{(x - 1)}^{2} {(x + 2)}^{4}} d t

\Rightarrow

- 3 \int \frac{t}{t^{3} - 1} d t = - \int \frac{d t}{t - 1} + \int \frac{t - 1}{t^{2} + t + 1} d t =

= - \ln (t - 1) + \frac{1}{2} \ln (t^{2} + t + 1) - \sqrt{3} \arctan \frac{2 t + 1}{\sqrt{3}} =

= \frac{1}{2} \ln \frac{t^{2} + t + 1}{{(t - 1)}^{2}} - \sqrt{3} \arctan \frac{2 t + 1}{\sqrt{3}}

\dots

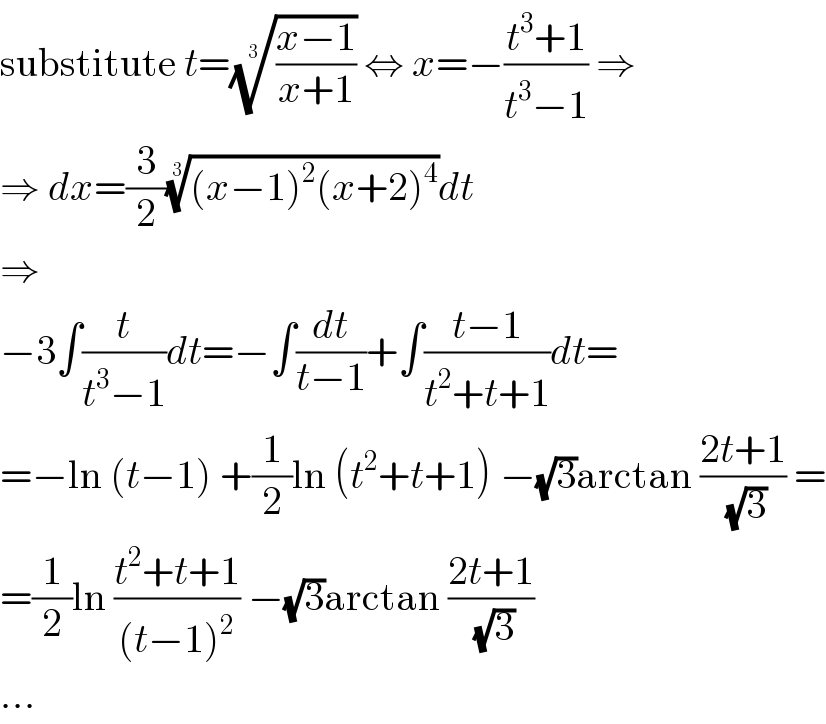
Commented by bemath last updated on 03/Sep/20

how you get t = \sqrt[3]{\frac{x - 1}{x + 1}} ?

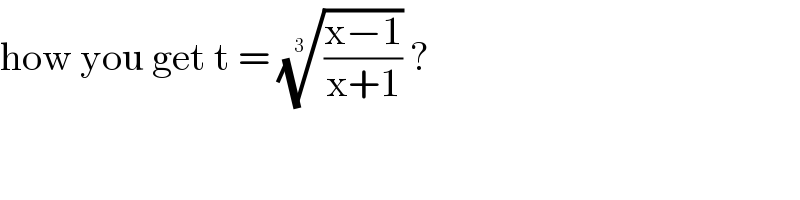
Commented by Sarah85 last updated on 03/Sep/20

try and error

Answered by mindispower last updated on 02/Sep/20

= \int \frac{}{{(x + 1)}^{2}} . \sqrt[3]{\frac{x + 1}{x - 1}} d x

l e t r = \frac{x - 1}{x + 1} \Rightarrow d r = \frac{2}{{(x + 1)}^{2}} d x

\Leftrightarrow= \int \frac{d r}{2 \sqrt[3]{r}} = \frac{1}{2} \int r^{\frac{- 1}{3}} d r = \frac{3}{4} r^{\frac{2}{3}} + c

Commented by Her_Majesty last updated on 02/Sep/20

s o m e t h i n g w e n t w r o n g I t h i n k

Commented by bobhans last updated on 03/Sep/20

\sqrt[3]{x - 1} \sqrt[3]{{(x + 1)}^{2}} \times \frac{\sqrt[3]{x + 1}}{\sqrt[3]{x + 1}} = (x + 1) \sqrt{\frac{x - 1}{x + 1}}

not {(x + 1)}^{2} \sqrt{\frac{x - 1}{x + 1}}

Facebook Tweet Pin