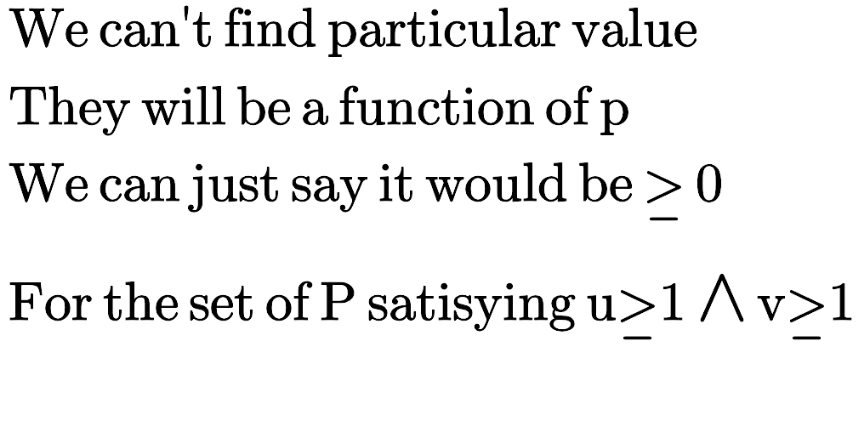Question Number 107385 by bemath last updated on 10/Aug/20

Commented by Her_Majesty last updated on 10/Aug/20

Commented by bemath last updated on 10/Aug/20

Commented by bemath last updated on 10/Aug/20
Commented by bemath last updated on 10/Aug/20
Commented by bemath last updated on 10/Aug/20
Commented by bemath last updated on 10/Aug/20
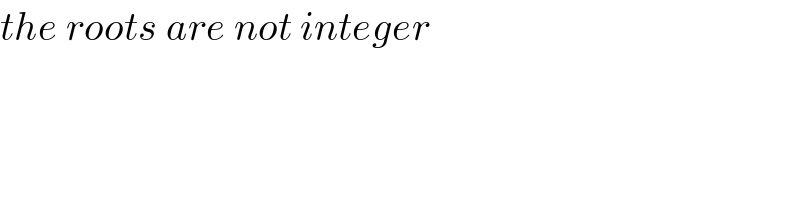
Commented by hgrocks last updated on 10/Aug/20