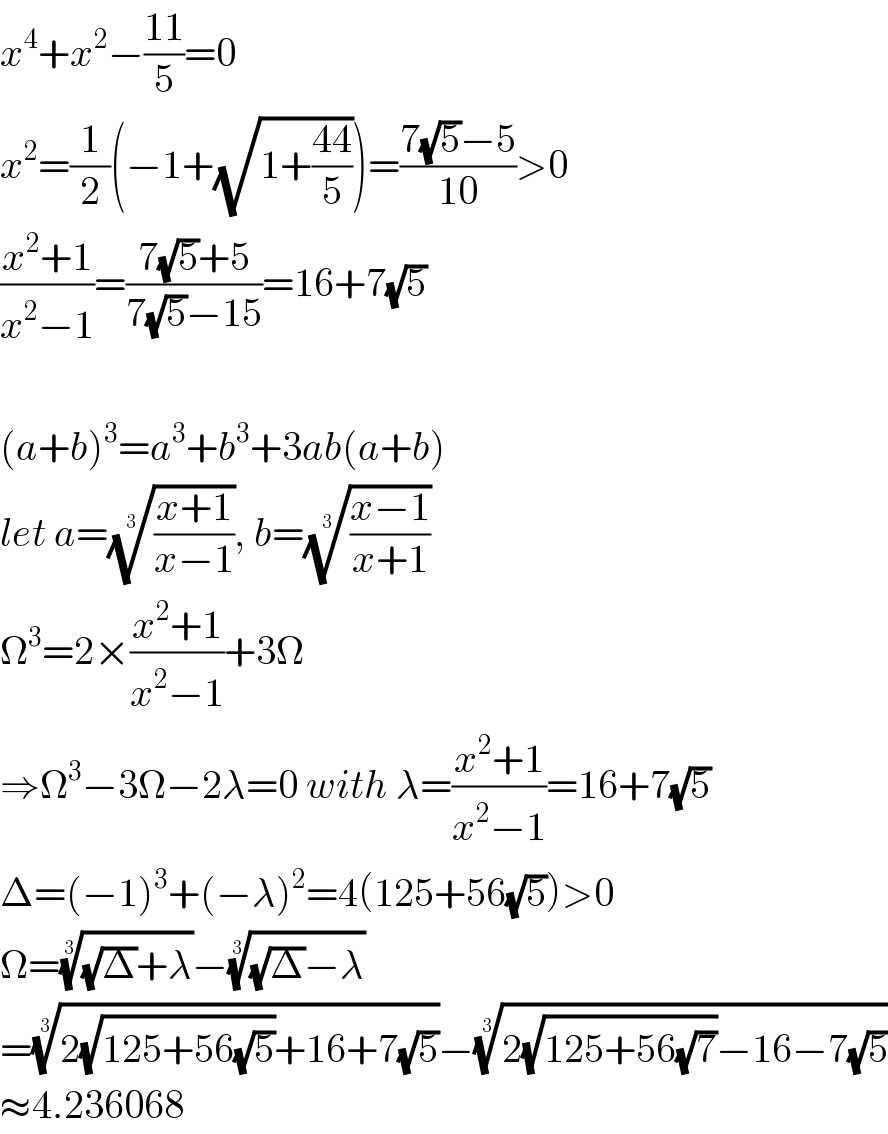BeMath-If-x-4-x-2-11-5-find-the-value-of-x-1-x-1-1-3-x-1-x-1-1-3-

Question Number 108316 by bemath last updated on 16/Aug/20

\frac{▽ B e M a t h ▽}{△}

I f x^{4} + x^{2} = \frac{11}{5}, f i n d t h e v a l u e o f

Ω = \sqrt[3]{\frac{x + 1}{x - 1}} + \sqrt[3]{\frac{x - 1}{x + 1}}

Answered by bobhans last updated on 16/Aug/20

\frac{b o b h a n s}{ψ}

c o n s i d e r \sqrt[3]{x} + \sqrt[3]{y} = \frac{x + y}{\sqrt[3]{x^{2}} - \sqrt[3]{x y} + \sqrt[3]{y^{2}}}

n o w \sqrt[3]{\frac{x + 1}{x - 1}} + \sqrt[3]{\frac{x - 1}{x + 1}} = \frac{\frac{x + 1}{x - 1} + \frac{x - 1}{x + 1}}{\sqrt[3]{{(\frac{x + 1}{x - 1})}^{2}} + \sqrt[3]{{(\frac{x - 1}{x + 1})}^{2}} - 1}

= \frac{2 x^{2} + 2}{(x^{2} - 1) {\sqrt[3]{{(\frac{x + 1}{x - 1})}^{2}} + \sqrt[3]{{(\frac{x - 1}{x + 1})}^{2}} - 1}}

(*) f r o m t h e e q u a t i o n x^{4} + x^{2} = \frac{11}{5}

l e t x^{2} = z \Rightarrow 5 z^{2} + 5 z - 11 = 0

z = \frac{- 5 \pm \sqrt{25 + 220}}{10} = \frac{- 5 \pm 7 \sqrt{5}}{10}

i f x \in R, w e t a k e z = \frac{- 5 + 7 \sqrt{5}}{10} \approx 1.065

a n d x = \sqrt{1.065} \approx 1.032

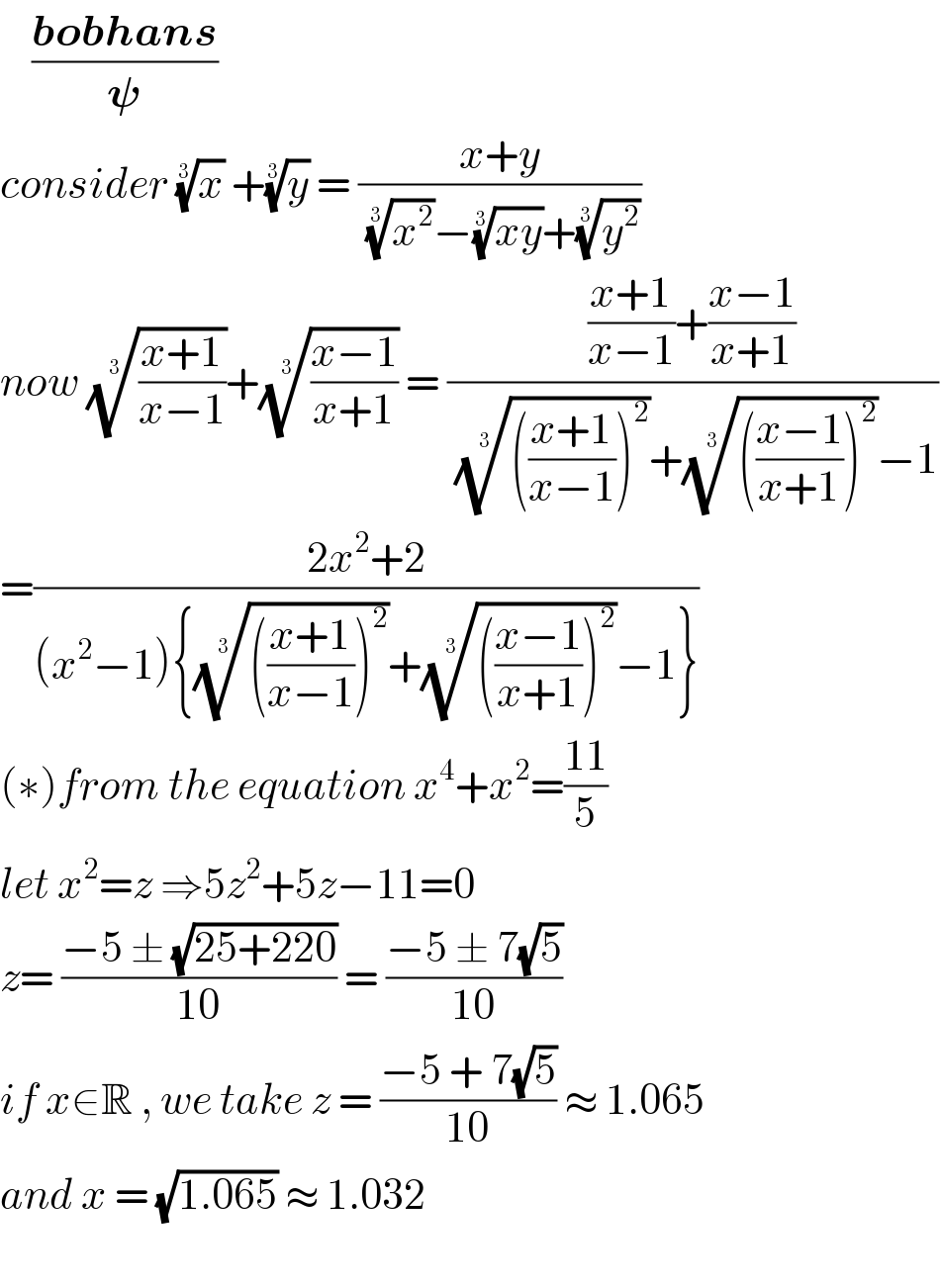
Answered by Rasheed.Sindhi last updated on 16/Aug/20

x^{4} + x^{2} = \frac{11}{5} \Rightarrow x^{2} = \frac{- 5 \pm 7 \sqrt{5}}{10}

Ω = \sqrt[3]{\frac{x + 1}{x - 1}} + \sqrt[3]{\frac{x - 1}{x + 1}}

Ω^{3} = {(\sqrt[3]{\frac{x + 1}{x - 1}} + \sqrt[3]{\frac{x - 1}{x + 1}})}^{3}

= \frac{x + 1}{x - 1} + \frac{x - 1}{x + 1} + 3 (\sqrt[3]{\frac{x + 1}{x - 1}} + \sqrt[3]{\frac{x - 1}{x + 1}})

Ω^{3} - 3 Ω = \frac{2 (x^{2} + 1)}{x^{2} - 1}

= 2 (\frac{\frac{- 5 \pm 7 \sqrt{5}}{10} + 1}{\frac{- 5 \pm 7 \sqrt{5}}{10} - 1}) = 2 (\frac{5 \pm 7 \sqrt{5}}{- 15 \pm 7 \sqrt{5}})

= 2 (\frac{5 \pm 7 \sqrt{5}}{- 15 \pm 7 \sqrt{5}} \times \frac{- 15 \mp 7 \sqrt{5}}{- 15 \mp 7 \sqrt{5}})

= 2 (\frac{- 75 \mp 35 \sqrt{5} \mp 105 \sqrt{5} - 49 (5)}{225 - 49 (5)})

= \frac{320 \pm 140 \sqrt{5}}{10} = 32 \pm 14 \sqrt{5}

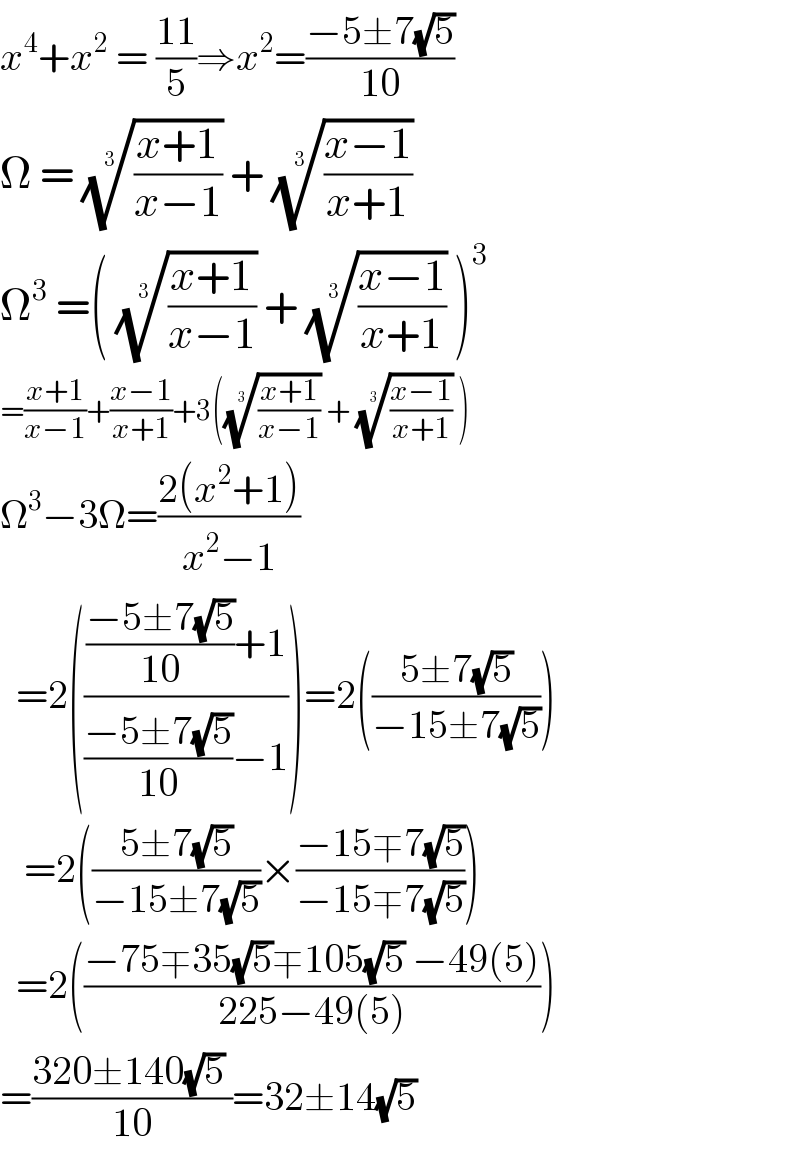
Commented by bemath last updated on 16/Aug/20

c o l l a n d n i c e

Answered by mr W last updated on 16/Aug/20

x^{4} + x^{2} - \frac{11}{5} = 0

x^{2} = \frac{1}{2} (- 1 + \sqrt{1 + \frac{44}{5}}) = \frac{7 \sqrt{5} - 5}{10} > 0

\frac{x^{2} + 1}{x^{2} - 1} = \frac{7 \sqrt{5} + 5}{7 \sqrt{5} - 15} = 16 + 7 \sqrt{5}

{(a + b)}^{3} = a^{3} + b^{3} + 3 a b (a + b)

l e t a = \sqrt[3]{\frac{x + 1}{x - 1}}, b = \sqrt[3]{\frac{x - 1}{x + 1}}

Ω^{3} = 2 \times \frac{x^{2} + 1}{x^{2} - 1} + 3 Ω

\Rightarrow Ω^{3} - 3 Ω - 2 λ = 0 w i t h λ = \frac{x^{2} + 1}{x^{2} - 1} = 16 + 7 \sqrt{5}

Δ = {(- 1)}^{3} + {(- λ)}^{2} = 4 (125 + 56 \sqrt{5}) > 0

Ω = \sqrt[3]{\sqrt{Δ} + λ} - \sqrt[3]{\sqrt{Δ} - λ}

= \sqrt[3]{2 \sqrt{125 + 56 \sqrt{5}} + 16 + 7 \sqrt{5}} - \sqrt[3]{2 \sqrt{125 + 56 \sqrt{7}} - 16 - 7 \sqrt{5}}

\approx 4.236068

Facebook Tweet Pin