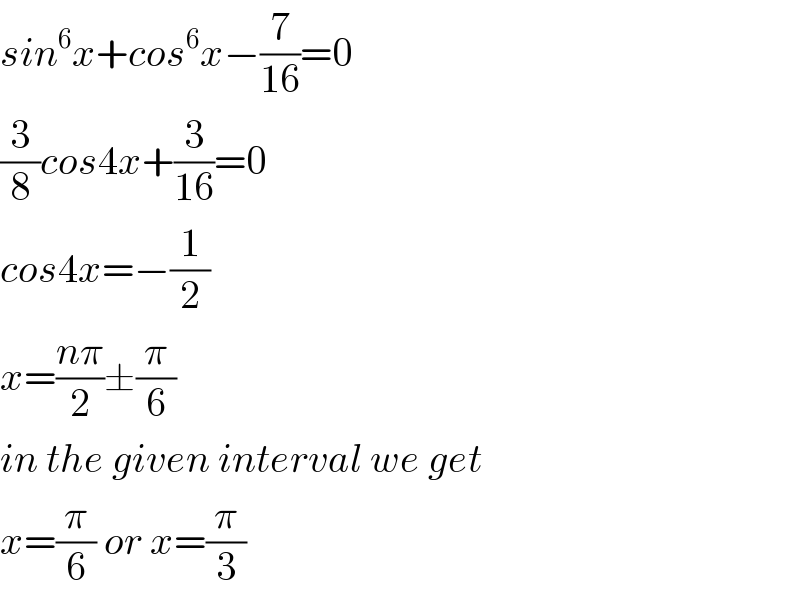BeMath-sin-6-x-cos-6-x-7-16-x-0-pi-2-

Question Number 107534 by bemath last updated on 11/Aug/20

⊨ B e M a t h ⊨

\sin^{6} x + \cos^{6} x = \frac{7}{16}; x \in (0, \frac{π}{2})

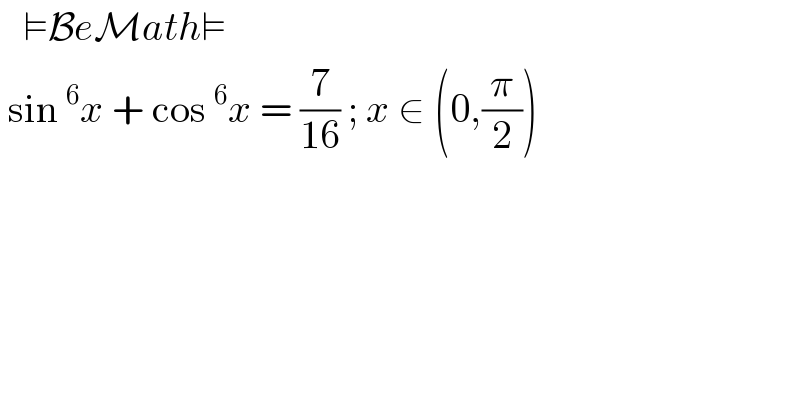
Commented by hgrocks last updated on 11/Aug/20

7 and 16 both my favorite numbers ��

Commented by bemath last updated on 11/Aug/20

t h a n k y o u a l l s i r

Answered by Rio Michael last updated on 11/Aug/20

{(\sin^{2} x + \cos^{2} x)}^{3} = (\sin^{2} x + \cos^{2} x) (\sin^{2} x + \cos^{2} x) (\sin^{2} x + \cos^{2} x)

= (\sin^{4} x + {2 \sin}^{2} x \cos^{2} x + \cos^{4} x) (\sin^{2} x + \cos^{2} x)

= \sin^{6} x + \sin^{4} x \cos^{2} x + {2 \sin}^{4} x \cos^{2} x + {2 \sin}^{2} x \cos^{4} x + \sin^{2} x \cos^{4} x + \cos^{6} x

= \sin^{6} x + \cos^{6} x + {3 \sin}^{4} x \cos^{2} x + 3 \sin^{2} x \cos^{4} x

\Rightarrow 1 - 3 \sin^{4} x \cos^{2} x - 3 \sin^{2} x \cos^{4} x = \sin^{6} x + \cos^{6} x

\Rightarrow

\sin^{6} x + \cos^{6} x = 1 - {3 \sin}^{2} x \cos^{2} x (\sin^{2} x + \cos^{2} x)

= 1 - \frac{3}{4} \sin^{2} 2 x

\Rightarrow 1 - \frac{3}{4} \sin^{2} 2 x = \frac{7}{16}; x \in (0, \frac{π}{2})

- \frac{3}{4} \sin^{2} 2 x = - \frac{9}{16}

or \sin^{2} 2 x = \frac{3}{4}

\Rightarrow \sin 2 x = \frac{\sqrt{3}}{2} or 2 x = π n + {(- 1)}^{n} \frac{π}{3}

x = \frac{π n}{2} + {(- 1)}^{n} \frac{π}{6}

n = 0, x = \frac{π}{6}

n = 1, x = \frac{π}{3}

n = 2, x = out of range given \Rightarrow solution set S = {\frac{π}{6}, \frac{π}{3}}

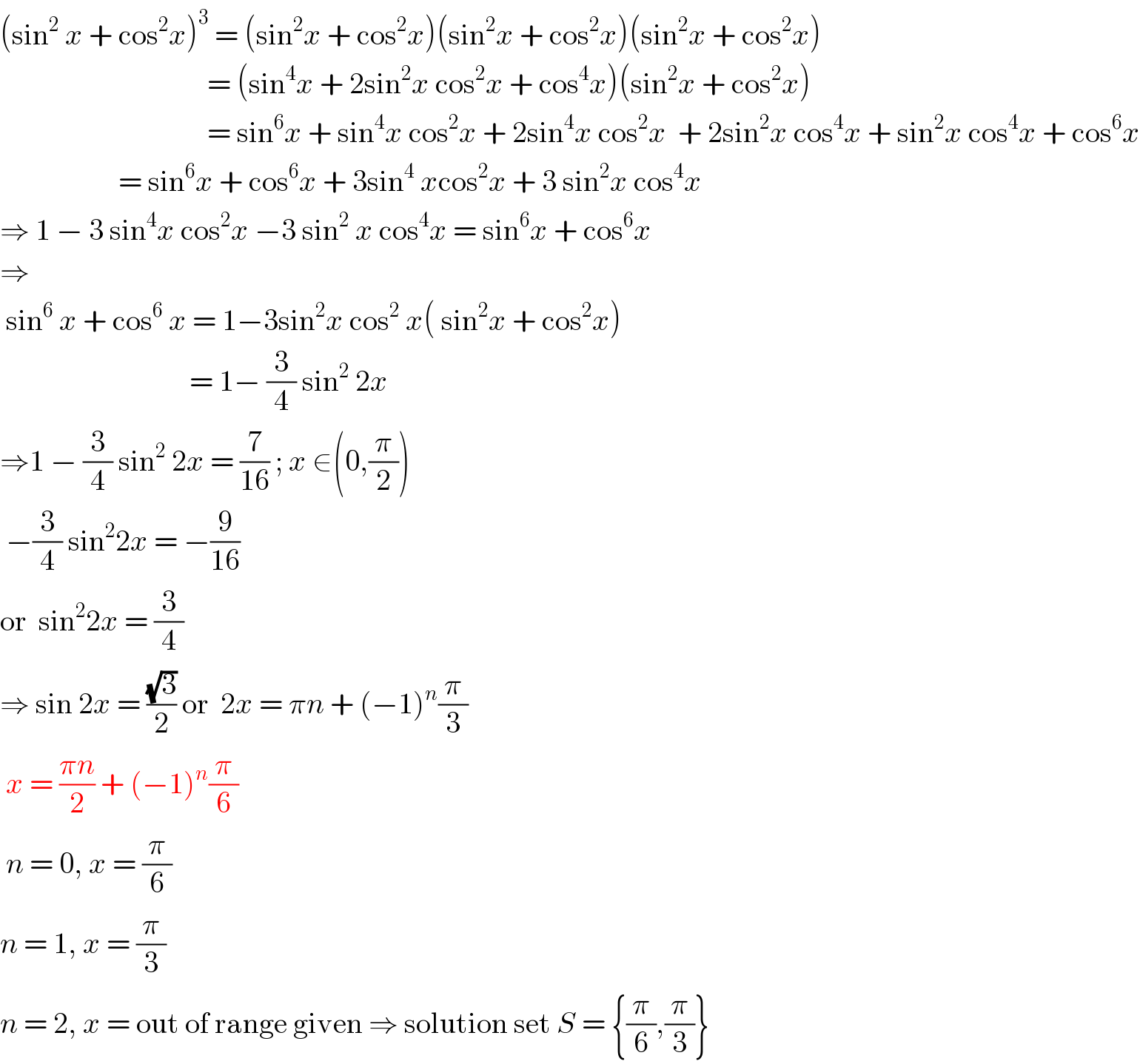
Answered by Dwaipayan Shikari last updated on 11/Aug/20

{({s i n}^{2} x + {c o s}^{2} x)}^{3} - 3 {s i n}^{2} {x c o s}^{2} x ({s i n}^{2} x + {c o s}^{2} x) = \frac{7}{16}

1 - 3 {c o s}^{2} {x s i n}^{2} x = \frac{7}{16}

{c o s}^{2} {x s i n}^{2} x = \frac{3}{16}

4 {c o s}^{2} {x s i n}^{2} x = \frac{3}{4}

{s i n}^{2} 2 x = \frac{3}{4}

s i n 2 x = \pm \frac{\sqrt{3}}{2}

x = π k \pm \frac{π}{3}

x = \frac{π k}{2} \pm \frac{π}{6} (k \in Z)

{\begin{cases} x = \frac{π}{6} \\ x = \frac{π}{3} \end{cases}

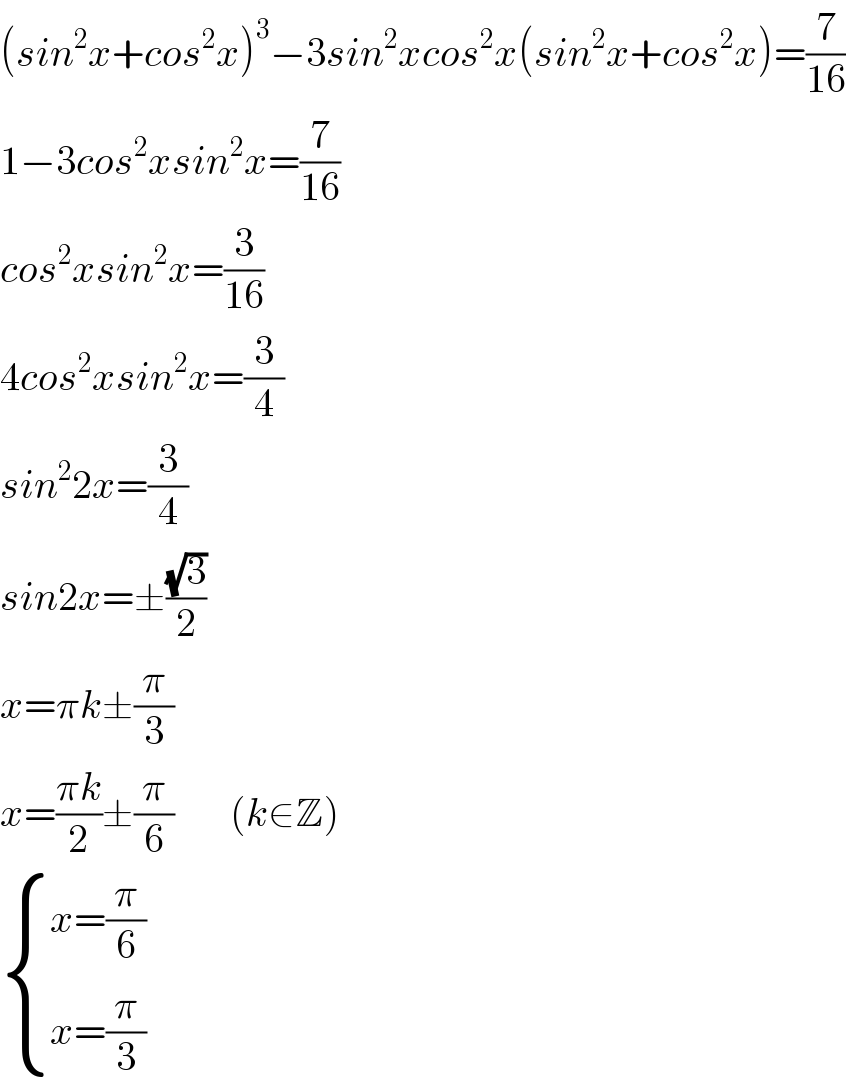
Answered by Sarah85 last updated on 11/Aug/20

s^{6} + {(1 - s^{2})}^{6 / 2} = 7 / 16

s^{4} - s^{2} + 3 / 16 = 0

\sin x = \pm \sqrt{3} / 2 \lor \pm 1 / 2

x = π / 6 \lor x = π / 3

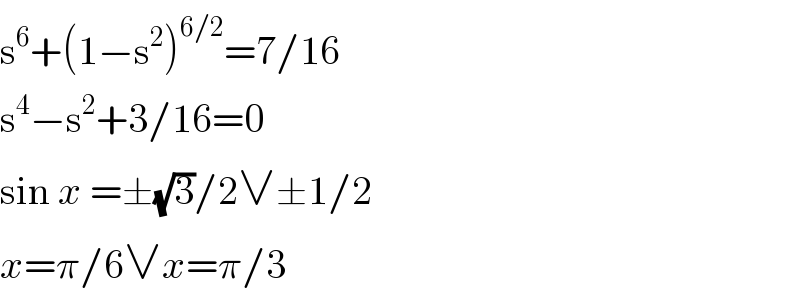
Answered by john santu last updated on 11/Aug/20

⋇ JS ⋇

\sin^{6} x + \cos^{6} x = {(\sin^{2} x)}^{3} + {(\cos^{2} x)}^{3}

= {(\sin^{2} x + \cos^{2} x)}^{3} - 3 \sin^{2} x \cos^{2} x (\sin^{2} x + \cos^{2} x)

= 1 - \frac{3}{4} \sin^{2} 2 x

(⊸) \frac{7}{16} = 1 - \frac{3}{4} \sin^{2} 2 x

7 = 16 - 12 \sin^{2} 2 x \Rightarrow 12 \sin^{2} 2 x - 9 = 0

\Rightarrow \sin 2 x = \pm \frac{3}{2 \sqrt{3}} = \pm \frac{\sqrt{3}}{2}

c a s e (1) \Rightarrow \sin 2 x = \sin 60 °; x = 30 °

2 x = 180 ° - 60 ° + k . 360 °

x = 60 ° + k . 180 °; x = 60 °

c a s e (2) \sin 2 x = \sin (- 60 °)

x = - 30 ° + k . 180 °; x = 150 °

(i g n o r i n g)

T h e r e f o r e t h e s o l u t i o n

x = 30 °; 60 °

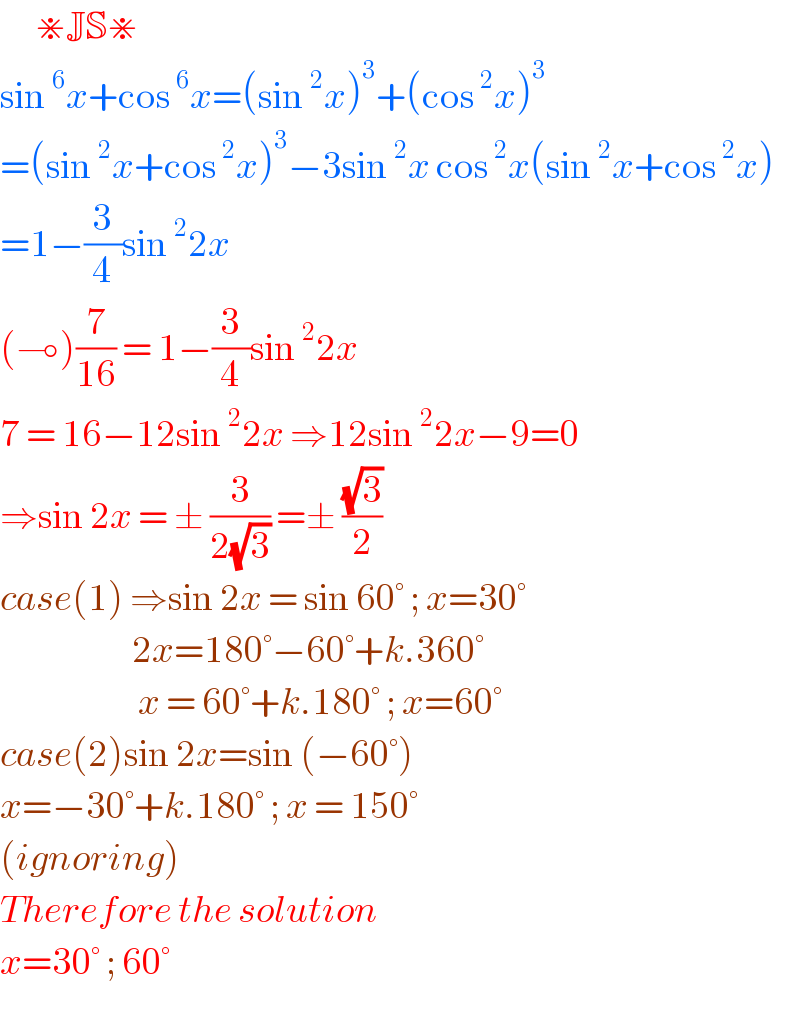
Answered by Her_Majesty last updated on 11/Aug/20

{s i n}^{6} x + {c o s}^{6} x - \frac{7}{16} = 0

\frac{3}{8} c o s 4 x + \frac{3}{16} = 0

c o s 4 x = - \frac{1}{2}

x = \frac{n π}{2} \pm \frac{π}{6}

i n t h e g i v e n i n t e r v a l w e g e t

x = \frac{π}{6} o r x = \frac{π}{3}