Question Number 33331 by prof Abdo imad last updated on 14/Apr/18
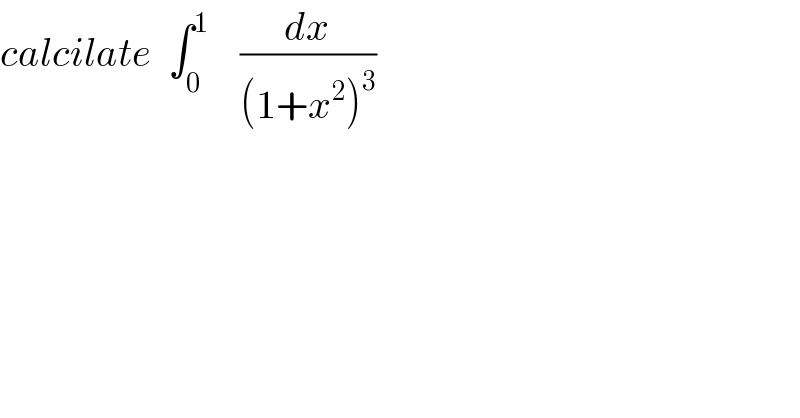
$${calcilate}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{{dx}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{3}} } \\ $$
Commented by math khazana by abdo last updated on 19/Apr/18
![let put I = ∫_0 ^1 (dx/((1+x^2 )^3 )) changement x=tantgive I = ∫_0 ^(π/4) (1/((1+tan^2 t)^3 )) (1+tan^2 t)dt = ∫_0 ^( (π/4)) (dt/((1+tan^2 t)^2 )) =∫_0 ^(π/4) cos^4 t dt = ∫_0 ^(π/4) (1/4)(1+cos(2t))^2 dt = (1/4) ∫_0 ^(π/4) (1+2cos(2t) +cos^2 (2t))dt = (π/(16)) +(1/2) ∫_0 ^(π/4) cos(2t) +(1/8) ∫_0 ^(π/4) ( 1+cos(4t))dt = (π/(16)) +(1/4)[sin(2t)]_0 ^(π/4) + (π/(32)) +(1/(32))[ sin(4t)]_0 ^(π/4) = ((3π)/(32)) +(1/4) +0 ⇒ I = (1/4) +((3π)/(32)) .](https://www.tinkutara.com/question/Q33565.png)
$${let}\:{put}\:\:{I}\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{{dx}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{3}} }\:\:{changement}\:{x}={tantgive} \\ $$$${I}\:\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\:\:\:\frac{\mathrm{1}}{\left(\mathrm{1}+{tan}^{\mathrm{2}} {t}\right)^{\mathrm{3}} }\:\left(\mathrm{1}+{tan}^{\mathrm{2}} {t}\right){dt} \\ $$$$=\:\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{4}}} \:\:\:\:\frac{{dt}}{\left(\mathrm{1}+{tan}^{\mathrm{2}} {t}\right)^{\mathrm{2}} }\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:{cos}^{\mathrm{4}} {t}\:{dt} \\ $$$$=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{1}}{\mathrm{4}}\left(\mathrm{1}+{cos}\left(\mathrm{2}{t}\right)\right)^{\mathrm{2}} {dt} \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{4}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\left(\mathrm{1}+\mathrm{2}{cos}\left(\mathrm{2}{t}\right)\:+{cos}^{\mathrm{2}} \left(\mathrm{2}{t}\right)\right){dt} \\ $$$$=\:\frac{\pi}{\mathrm{16}}\:+\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:{cos}\left(\mathrm{2}{t}\right)\:\:+\frac{\mathrm{1}}{\mathrm{8}}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \left(\:\mathrm{1}+{cos}\left(\mathrm{4}{t}\right)\right){dt} \\ $$$$=\:\frac{\pi}{\mathrm{16}}\:\:+\frac{\mathrm{1}}{\mathrm{4}}\left[{sin}\left(\mathrm{2}{t}\right)\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:+\:\frac{\pi}{\mathrm{32}}\:\:+\frac{\mathrm{1}}{\mathrm{32}}\left[\:{sin}\left(\mathrm{4}{t}\right)\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \\ $$$$=\:\frac{\mathrm{3}\pi}{\mathrm{32}}\:\:\:+\frac{\mathrm{1}}{\mathrm{4}}\:+\mathrm{0}\:\Rightarrow\:\:{I}\:=\:\frac{\mathrm{1}}{\mathrm{4}}\:+\frac{\mathrm{3}\pi}{\mathrm{32}}\:. \\ $$
