Question Number 63552 by minh2001 last updated on 05/Jul/19
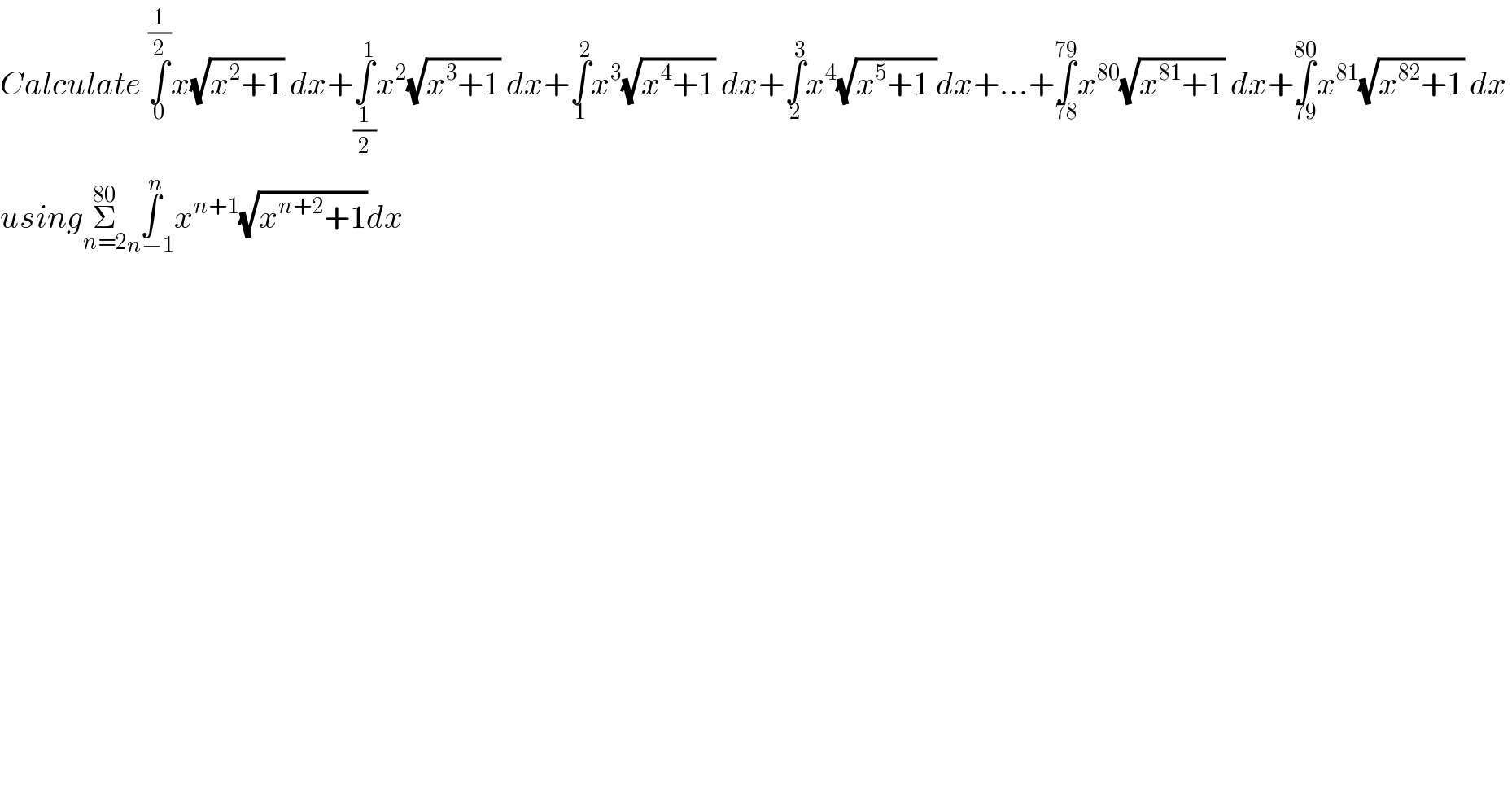
$${Calculate}\:\underset{\mathrm{0}} {\overset{\frac{\mathrm{1}}{\mathrm{2}}} {\int}}{x}\sqrt{{x}^{\mathrm{2}} +\mathrm{1}}\:{dx}+\underset{\frac{\mathrm{1}}{\mathrm{2}}} {\overset{\mathrm{1}} {\int}}{x}^{\mathrm{2}} \sqrt{{x}^{\mathrm{3}} +\mathrm{1}}\:{dx}+\underset{\mathrm{1}} {\overset{\mathrm{2}} {\int}}{x}^{\mathrm{3}} \sqrt{{x}^{\mathrm{4}} +\mathrm{1}}\:{dx}+\underset{\mathrm{2}} {\overset{\mathrm{3}} {\int}}{x}^{\mathrm{4}} \sqrt{{x}^{\mathrm{5}} +\mathrm{1}\:}{dx}+…+\underset{\mathrm{78}} {\overset{\mathrm{79}} {\int}}{x}^{\mathrm{80}} \sqrt{{x}^{\mathrm{81}} +\mathrm{1}}\:{dx}+\underset{\mathrm{79}} {\overset{\mathrm{80}} {\int}}{x}^{\mathrm{81}} \sqrt{{x}^{\mathrm{82}} +\mathrm{1}}\:{dx} \\ $$$${using}\underset{{n}=\mathrm{2}} {\overset{\mathrm{80}} {\sum}}\underset{{n}−\mathrm{1}} {\overset{{n}} {\int}}{x}^{{n}+\mathrm{1}} \sqrt{{x}^{{n}+\mathrm{2}} +\mathrm{1}}{dx} \\ $$
Commented by Prithwish sen last updated on 05/Jul/19
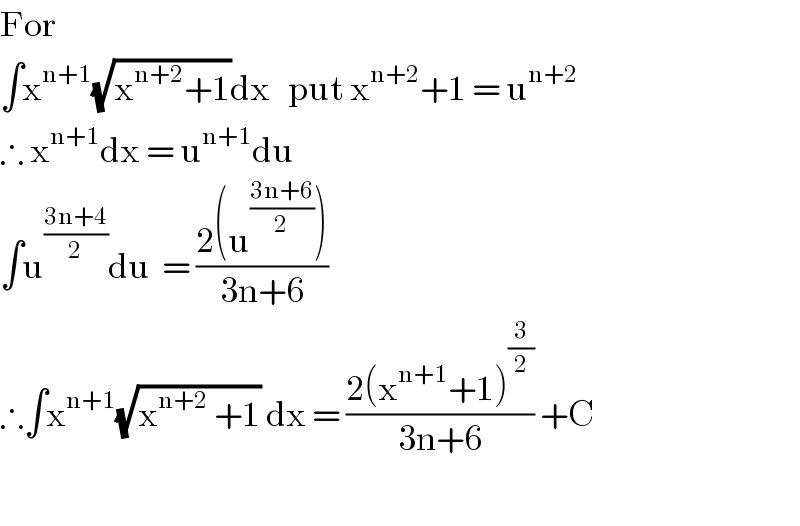
$$\mathrm{For} \\ $$$$\int\mathrm{x}^{\mathrm{n}+\mathrm{1}} \sqrt{\mathrm{x}^{\mathrm{n}+\mathrm{2}} +\mathrm{1}}\mathrm{dx}\:\:\:\mathrm{put}\:\mathrm{x}^{\mathrm{n}+\mathrm{2}} +\mathrm{1}\:=\:\mathrm{u}^{\mathrm{n}+\mathrm{2}} \\ $$$$\therefore\:\mathrm{x}^{\mathrm{n}+\mathrm{1}} \mathrm{dx}\:=\:\mathrm{u}^{\mathrm{n}+\mathrm{1}} \mathrm{du} \\ $$$$\int\mathrm{u}^{\frac{\mathrm{3n}+\mathrm{4}}{\mathrm{2}}} \mathrm{du}\:\:=\:\frac{\mathrm{2}\left(\mathrm{u}^{\frac{\mathrm{3n}+\mathrm{6}}{\mathrm{2}}} \right)}{\mathrm{3n}+\mathrm{6}} \\ $$$$\therefore\int\mathrm{x}^{\mathrm{n}+\mathrm{1}} \sqrt{\mathrm{x}^{\mathrm{n}+\mathrm{2}} \:+\mathrm{1}}\:\mathrm{dx}\:=\:\frac{\mathrm{2}\left(\mathrm{x}^{\mathrm{n}+\mathrm{1}} +\mathrm{1}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} }{\mathrm{3n}+\mathrm{6}}\:+\mathrm{C} \\ $$$$ \\ $$
Answered by MJS last updated on 05/Jul/19
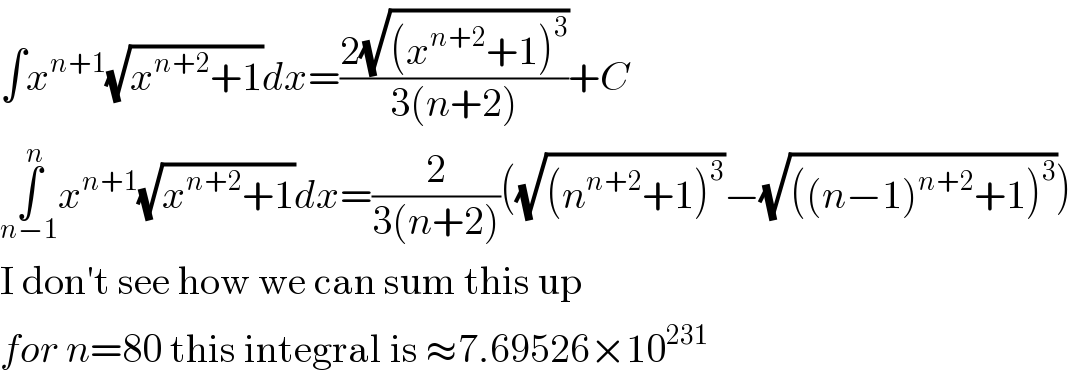
$$\int{x}^{{n}+\mathrm{1}} \sqrt{{x}^{{n}+\mathrm{2}} +\mathrm{1}}{dx}=\frac{\mathrm{2}\sqrt{\left({x}^{{n}+\mathrm{2}} +\mathrm{1}\right)^{\mathrm{3}} }}{\mathrm{3}\left({n}+\mathrm{2}\right)}+{C} \\ $$$$\underset{{n}−\mathrm{1}} {\overset{{n}} {\int}}{x}^{{n}+\mathrm{1}} \sqrt{{x}^{{n}+\mathrm{2}} +\mathrm{1}}{dx}=\frac{\mathrm{2}}{\mathrm{3}\left({n}+\mathrm{2}\right)}\left(\sqrt{\left({n}^{{n}+\mathrm{2}} +\mathrm{1}\right)^{\mathrm{3}} }−\sqrt{\left(\left({n}−\mathrm{1}\right)^{{n}+\mathrm{2}} +\mathrm{1}\right)^{\mathrm{3}} }\right) \\ $$$$\mathrm{I}\:\mathrm{don}'\mathrm{t}\:\mathrm{see}\:\mathrm{how}\:\mathrm{we}\:\mathrm{can}\:\mathrm{sum}\:\mathrm{this}\:\mathrm{up} \\ $$$${for}\:{n}=\mathrm{80}\:\mathrm{this}\:\mathrm{integral}\:\mathrm{is}\:\approx\mathrm{7}.\mathrm{69526}×\mathrm{10}^{\mathrm{231}} \\ $$
