Question Number 41516 by maxmathsup by imad last updated on 08/Aug/18
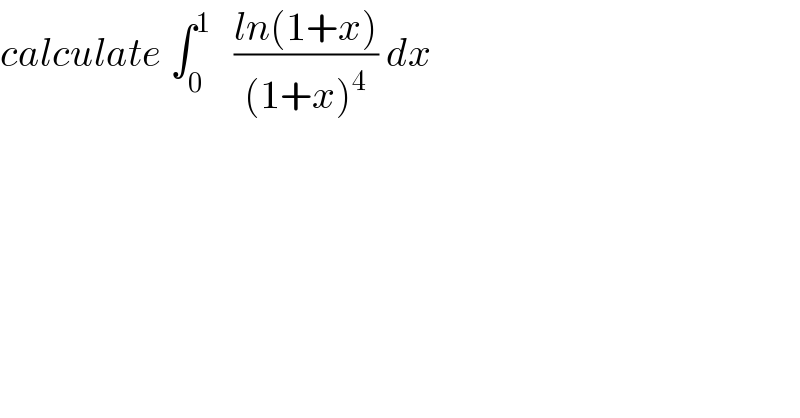
$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{ln}\left(\mathrm{1}+{x}\right)}{\left(\mathrm{1}+{x}\right)^{\mathrm{4}} }\:{dx} \\ $$
Commented by turbo msup by abdo last updated on 12/Aug/18
![let A =∫_0 ^1 ((ln(1+x))/((1+x)^4 ))dx changement 1+x =t give A = ∫_1 ^2 ((ln(t))/t^4 )dt and by parts u^′ =t^(−4) and v=ln(t) ⇒ A =[−(1/3)t^(−3) ln(t)]_1 ^2 +∫_1 ^2 (1/3) t^(−3) (dt/t) =−(1/3)((ln(2))/8) +(1/3) ∫_1 ^2 t^(−4) dt =−((ln(2))/(24)) +(1/3)[−(1/3)t^(−3) ]_1 ^2 =−((ln(2))/(24)) −(1/9)( (1/8) −1) =−((ln(2))/(24)) −(1/9)(((−7)/8)) =(7/(72)) −((ln(2))/(24))](https://www.tinkutara.com/question/Q41774.png)
$${let}\:{A}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{ln}\left(\mathrm{1}+{x}\right)}{\left(\mathrm{1}+{x}\right)^{\mathrm{4}} }{dx}\:{changement} \\ $$$$\mathrm{1}+{x}\:={t}\:{give} \\ $$$${A}\:=\:\int_{\mathrm{1}} ^{\mathrm{2}} \:\:\frac{{ln}\left({t}\right)}{{t}^{\mathrm{4}} }{dt}\:\:{and}\:{by}\:{parts} \\ $$$${u}^{'} \:={t}^{−\mathrm{4}} \:\:{and}\:{v}={ln}\left({t}\right)\:\Rightarrow \\ $$$${A}\:=\left[−\frac{\mathrm{1}}{\mathrm{3}}{t}^{−\mathrm{3}} {ln}\left({t}\right)\right]_{\mathrm{1}} ^{\mathrm{2}} +\int_{\mathrm{1}} ^{\mathrm{2}} \frac{\mathrm{1}}{\mathrm{3}}\:{t}^{−\mathrm{3}} \:\frac{{dt}}{{t}} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{3}}\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{8}}\:\:+\frac{\mathrm{1}}{\mathrm{3}}\:\int_{\mathrm{1}} ^{\mathrm{2}} \:\:{t}^{−\mathrm{4}} \:{dt} \\ $$$$=−\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{24}}\:+\frac{\mathrm{1}}{\mathrm{3}}\left[−\frac{\mathrm{1}}{\mathrm{3}}{t}^{−\mathrm{3}} \right]_{\mathrm{1}} ^{\mathrm{2}} \\ $$$$=−\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{24}}\:−\frac{\mathrm{1}}{\mathrm{9}}\left(\:\frac{\mathrm{1}}{\mathrm{8}}\:−\mathrm{1}\right) \\ $$$$=−\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{24}}\:−\frac{\mathrm{1}}{\mathrm{9}}\left(\frac{−\mathrm{7}}{\mathrm{8}}\right) \\ $$$$=\frac{\mathrm{7}}{\mathrm{72}}\:−\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{24}} \\ $$
Answered by alex041103 last updated on 09/Aug/18
![∫_0 ^1 ((ln(1+x))/((1+x)^4 )) dx=∫_0 ^1 ((ln(1+x))/((1+x)^4 )) d(x+1)= =∫_1 ^2 ((ln(x))/x^4 ) dx=I let x=e^u ⇒dx=e^u du ⇒I=∫_0 ^( ln(2)) (u/e^(4u) )e^u du=∫_0 ^(ln(2)) ue^(−3u) du= =−(1/3)∫_0 ^(ln(2)) ud(e^(−3u) ) We integrate by parts: −3I=ue^(−3u) ]_0 ^(ln(2)) −∫_0 ^(ln(2)) e^(−3u) du= =((ln(2))/8)−(7/(24)) ⇒I=(7/(72))−((ln(2))/(24))≈0.0683411](https://www.tinkutara.com/question/Q41548.png)
$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{ln}\left(\mathrm{1}+{x}\right)}{\left(\mathrm{1}+{x}\right)^{\mathrm{4}} }\:{dx}=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{ln}\left(\mathrm{1}+{x}\right)}{\left(\mathrm{1}+{x}\right)^{\mathrm{4}} }\:{d}\left({x}+\mathrm{1}\right)= \\ $$$$=\int_{\mathrm{1}} ^{\mathrm{2}} \:\:\:\frac{{ln}\left({x}\right)}{{x}^{\mathrm{4}} }\:{dx}={I} \\ $$$${let}\:{x}={e}^{{u}} \Rightarrow{dx}={e}^{{u}} {du} \\ $$$$\Rightarrow{I}=\int_{\mathrm{0}} ^{\:{ln}\left(\mathrm{2}\right)} \frac{{u}}{{e}^{\mathrm{4}{u}} }{e}^{{u}} {du}=\int_{\mathrm{0}} ^{{ln}\left(\mathrm{2}\right)} {ue}^{−\mathrm{3}{u}} {du}= \\ $$$$=−\frac{\mathrm{1}}{\mathrm{3}}\int_{\mathrm{0}} ^{{ln}\left(\mathrm{2}\right)} {ud}\left({e}^{−\mathrm{3}{u}} \right) \\ $$$${We}\:{integrate}\:{by}\:{parts}: \\ $$$$\left.−\mathrm{3}{I}={ue}^{−\mathrm{3}{u}} \right]_{\mathrm{0}} ^{{ln}\left(\mathrm{2}\right)} −\int_{\mathrm{0}} ^{{ln}\left(\mathrm{2}\right)} {e}^{−\mathrm{3}{u}} {du}= \\ $$$$=\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{8}}−\frac{\mathrm{7}}{\mathrm{24}} \\ $$$$\Rightarrow{I}=\frac{\mathrm{7}}{\mathrm{72}}−\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{24}}\approx\mathrm{0}.\mathrm{0683411} \\ $$
