Question Number 130835 by mathmax by abdo last updated on 29/Jan/21
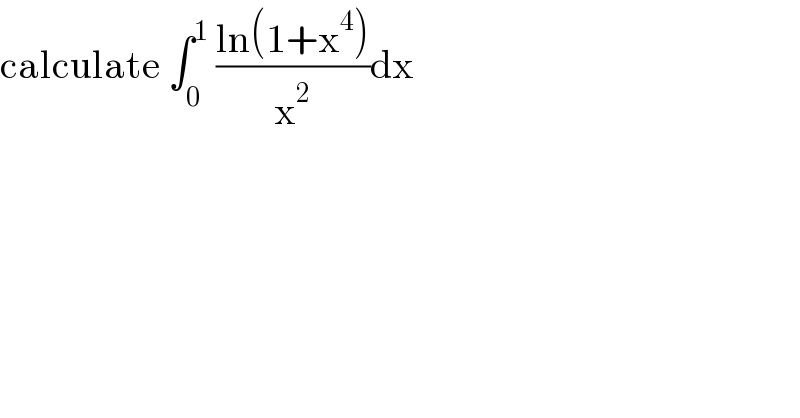
$$\mathrm{calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{ln}\left(\mathrm{1}+\mathrm{x}^{\mathrm{4}} \right)}{\mathrm{x}^{\mathrm{2}} }\mathrm{dx} \\ $$
Answered by Dwaipayan Shikari last updated on 29/Jan/21
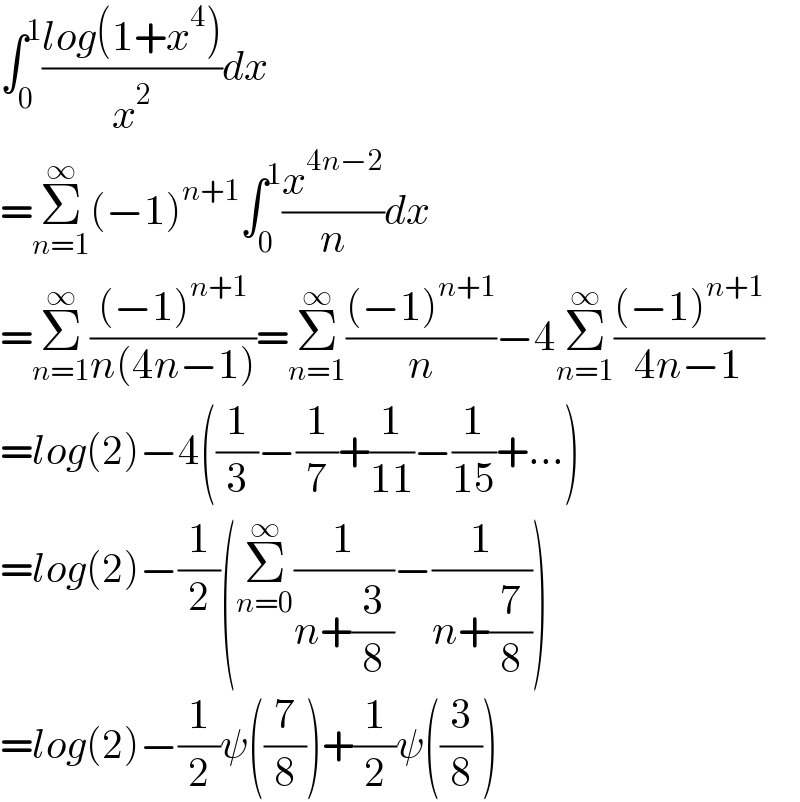
$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{log}\left(\mathrm{1}+{x}^{\mathrm{4}} \right)}{{x}^{\mathrm{2}} }{dx} \\ $$$$=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} \int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{4}{n}−\mathrm{2}} }{{n}}{dx} \\ $$$$=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{{n}\left(\mathrm{4}{n}−\mathrm{1}\right)}=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{{n}}−\mathrm{4}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\mathrm{4}{n}−\mathrm{1}} \\ $$$$={log}\left(\mathrm{2}\right)−\mathrm{4}\left(\frac{\mathrm{1}}{\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{7}}+\frac{\mathrm{1}}{\mathrm{11}}−\frac{\mathrm{1}}{\mathrm{15}}+…\right) \\ $$$$={log}\left(\mathrm{2}\right)−\frac{\mathrm{1}}{\mathrm{2}}\left(\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}+\frac{\mathrm{3}}{\mathrm{8}}}−\frac{\mathrm{1}}{{n}+\frac{\mathrm{7}}{\mathrm{8}}}\right) \\ $$$$={log}\left(\mathrm{2}\right)−\frac{\mathrm{1}}{\mathrm{2}}\psi\left(\frac{\mathrm{7}}{\mathrm{8}}\right)+\frac{\mathrm{1}}{\mathrm{2}}\psi\left(\frac{\mathrm{3}}{\mathrm{8}}\right) \\ $$
