calculate-0-2-dx-2-cos2x-

Question Number 93249 by 1549442205 last updated on 12/May/20

c a l c u l a t e \int_{0}^{\frac{Π}{2}} \frac{d x}{2 + c o s 2 x}

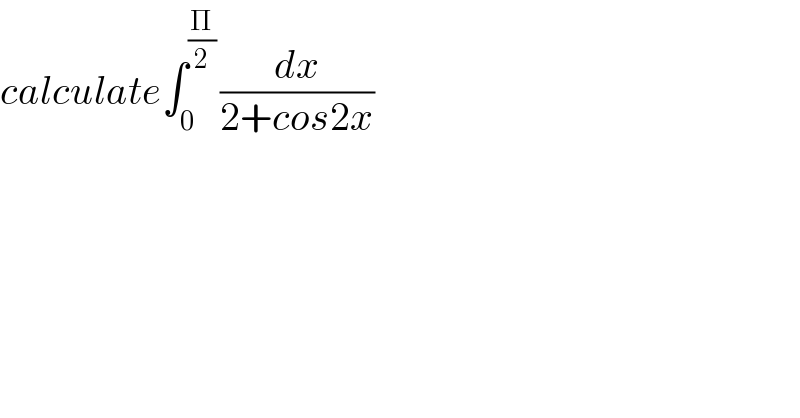
Commented by 1549442205 last updated on 12/May/20

C a l c u l a t e i t s v a l u e ?

Commented by abdomathmax last updated on 12/May/20

I = \int_{0}^{\frac{π}{2}} \frac{d x}{2 + c o s (2 x)} \Rightarrow I =_{2 x = t} \frac{1}{2} \int_{0}^{π} \frac{d t}{2 + c o s t}

=_{t a n (\frac{t}{2}) = u} \frac{1}{2} \int_{0}^{\infty} \frac{2 d u}{(1 + u^{2}) (2 + \frac{1 - u^{2}}{1 + u^{2}})}

= \int_{0}^{\infty} \frac{d u}{2 + 2 u^{2} + 1 - u^{2}} = \int_{0}^{\infty} \frac{d u}{3 + u^{2}} =_{u = \sqrt{3} z}

= \int_{0}^{\infty} \frac{\sqrt{3} d z}{3 (1 + z^{2})} = \frac{1}{\sqrt{3}} {[a r c t a n z]}_{0}^{+ \infty} = \frac{1}{\sqrt{3}} \times \frac{π}{2} \Rightarrow

I = \frac{π}{2 \sqrt{3}}

Commented by 1549442205 last updated on 12/May/20

T h a n k y o u v e r y m u c h!

Commented by mathmax by abdo last updated on 12/May/20

y o u a r e w e l c o m e .