calculate-0-dx-1-x-2-x-4-

Question Number 51324 by Abdo msup. last updated on 25/Dec/18

c a l c u l a t e \int_{0}^{+ \infty} \frac{d x}{1 + x^{2} + x^{4}}

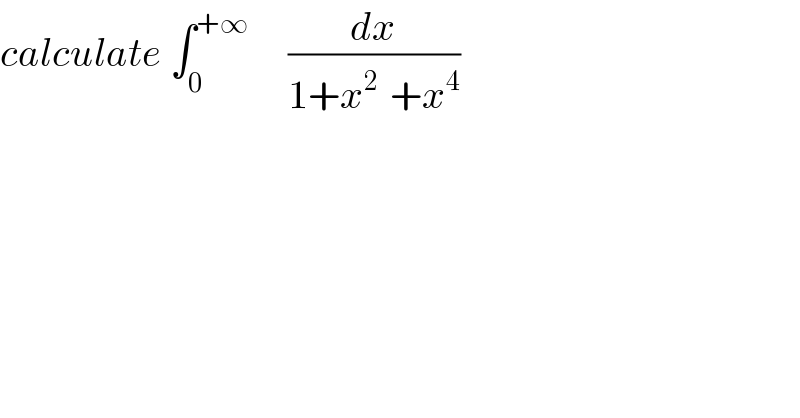
Answered by tanmay.chaudhury50@gmail.com last updated on 26/Dec/18

\int \frac{d x}{x^{4} + x^{2} + 1}

\frac{1}{2} \int \frac{\frac{2}{x^{2}}}{x^{2} + 1 + \frac{1}{x^{2}}}

\frac{1}{2} \int \frac{(1 + \frac{1}{x^{2}}) - (1 - \frac{1}{x^{2}})}{(x^{2} + \frac{1}{x^{2}} + 1)} d x

\frac{1}{2} \int \frac{d (x - \frac{1}{x})}{{(x - \frac{1}{x})}^{2} + 3} - \frac{1}{2} \int \frac{d (x + \frac{1}{x})}{{(x + \frac{1}{x})}^{2} - 1}

\frac{1}{2} \times \frac{1}{\sqrt{3}} {t a n}^{- 1} (\frac{x - \frac{1}{x}}{\sqrt{3}}) - \frac{1}{2} \times \frac{1}{2} l n (\frac{x + \frac{1}{x} - 1}{x + \frac{1}{x} + 1}) + c_{1}

= \frac{1}{2 \sqrt{3}} \times {t a n}^{- 1} (\frac{x^{2} - 1}{x \sqrt{3}}) - \frac{1}{4} l n (\frac{x^{2} - x + 1}{x^{2} + x + 1}) + c_{1}

r e q u i r e d a n s w e r

=∣ \frac{1}{2 \sqrt{3}} {t a n}^{- 1} (\frac{x^{2} - 1}{x \sqrt{3}}) - \frac{1}{4} l n (\frac{1 - \frac{1}{x} + \frac{1}{x^{2}}}{1 + \frac{1}{x} + \frac{1}{x^{2}}}) ∣_{0}^{\infty}

=∣ \frac{1}{2 \sqrt{3}} {t a n}^{- 1} (\frac{1 - \frac{1}{x^{2}}}{\frac{\sqrt{3}}{x}}) - d o ∣_{0}^{\infty}

= \frac{1}{2 \sqrt{3}} [{t a n}^{- 1} (\frac{1 - 0}{0}) - {t a n}^{- 1} (\frac{0^{2} - 1}{0 \times \sqrt{3}})] - \frac{1}{4} [l n (\frac{1 - 0 + 0}{1 + 0 + 0}) - l n (\frac{0^{2} - 0 + 1}{0^{2} + 0 + 1})]

= \frac{1}{2 \sqrt{3}} [t a n (\infty) - {t a n}^{- 1} (- \infty)] - d o

= \frac{1}{2 \sqrt{3}} [\frac{π}{2} - (\frac{- π}{2})]

= \frac{π}{2 \sqrt{3}}

Commented by peter frank last updated on 26/Dec/18

n i c e w o r k s i r

Answered by Smail last updated on 26/Dec/18

\frac{1}{(x^{2} + x + 1) (x^{2} - x + 1)} = \frac{a x + b}{x^{2} + x + 1} + \frac{c x + d}{x^{2} - x + 1}

a = - c, d = 1 - b

b = 1 - a

a = b = - c = d = \frac{1}{2}

A = \int_{0}^{\infty} \frac{d x}{1 + x^{2} + x^{4}} = \frac{1}{2} \int_{0}^{\infty} \frac{x + 1}{x^{2} + x + 1} d x - \frac{1}{2} \int_{0}^{\infty} \frac{x - 1}{x^{2} - x + 1} d x

= \frac{1}{4} \int_{0}^{\infty} \frac{2 x + 1 + 1}{x^{2} + x + 1} d x - \frac{1}{4} \int_{0}^{\infty} \frac{2 x - 1 - 1}{x^{2} - x + 1} d x

= \frac{1}{4} {[l n ∣ \frac{x^{2} + x + 1}{x^{2} - x + 1} ∣]}_{0}^{\infty} + \frac{1}{4} \int_{0}^{\infty} \frac{d x}{{(x + \frac{1}{2})}^{2} + \frac{3}{4}} + \frac{1}{4} \int_{0}^{\infty} \frac{d x}{{(x - \frac{1}{2})}^{2} + \frac{3}{4}}

= \frac{1}{3} \int_{0}^{\infty} \frac{d x}{{(\frac{2 x + 1}{\sqrt{3}})}^{2} + 1} + \frac{1}{3} \int_{0}^{\infty} \frac{d x}{{(\frac{2 x - 1}{\sqrt{3}})}^{2} + 1}

t = \frac{2 x \underline{+} 1}{\sqrt{3}} \Rightarrow d x = \frac{\sqrt{3}}{2} d t

A = \frac{1}{2 \sqrt{3}} \int_{1 / \sqrt{3}}^{\infty} \frac{d t}{t^{2} + 1} + \frac{1}{2 \sqrt{3}} \int_{- 1 / \sqrt{3}}^{\infty} \frac{d t}{t^{2} + 1}

= \frac{1}{2 \sqrt{3}} {[{t a n}^{- 1} (t)]}_{1 / \sqrt{3}}^{\infty} + \frac{1}{2 \sqrt{3}} {[{t a n}^{- 1} (t)]}_{- 1 / \sqrt{3}}^{\infty}

= \frac{1}{2 \sqrt{3}} (\frac{π}{2} - {t a n}^{- 1} (\frac{\sqrt{3}}{3})) + \frac{1}{2 \sqrt{3}} (\frac{π}{2} + {t a n}^{- 1} (\frac{\sqrt{3}}{3}))

= \frac{π}{2 \sqrt{3}}

Commented by peter frank last updated on 26/Dec/18

n i c e w o r k s i r