Question Number 40868 by math khazana by abdo last updated on 28/Jul/18
$${calculate}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{{x}}{{sinx}}{dx}\:\:. \\ $$
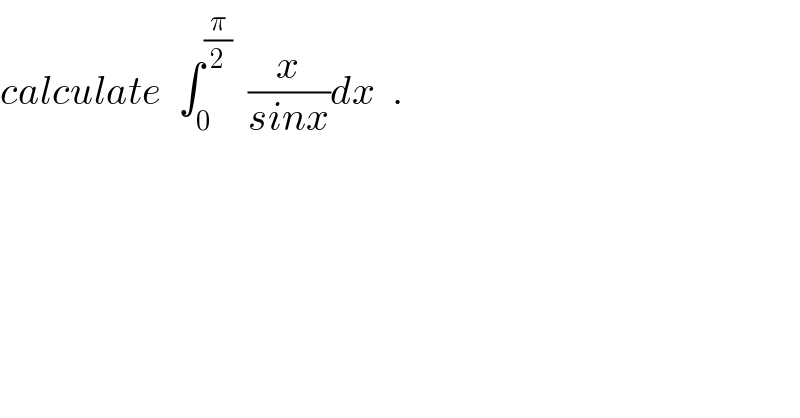
Commented by tanmay.chaudhury50@gmail.com last updated on 29/Jul/18
Commented by tanmay.chaudhury50@gmail.com last updated on 29/Jul/18
$$\frac{\Pi}{\mathrm{2}}=\mathrm{1}.\mathrm{57}\:\:{so}\:\mathrm{0}<\int_{\mathrm{0}} ^{\frac{\Pi}{\mathrm{2}}} \frac{{x}}{{sinx}}\leqslant\mathrm{18}\:\:\:{pls}\:{check} \\ $$
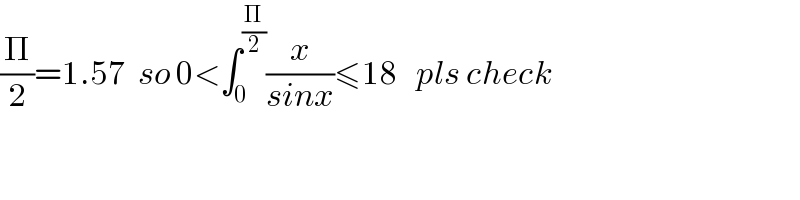
Commented by maxmathsup by imad last updated on 29/Jul/18
$${the}\:{Q}\:{is}\:{calculate}\:\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{{x}}{{sinx}}{dx}\:. \\ $$
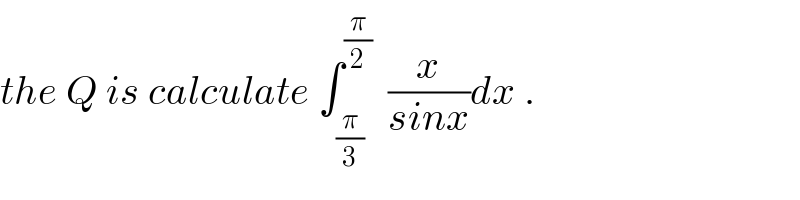
Commented by math khazana by abdo last updated on 30/Jul/18
![let I = ∫_(π/3) ^(π/2) (x/(sinx))dx changement tan((x/2))=t give I = ∫_(1/( (√3))) ^1 ((2arctant)/((2t)/(1+t^2 ))) ((2dt)/(1+t^2 )) =2 ∫_(1/( (√3))) ^1 ((arctant)/t) dt let introduce the parametric function f(x) = ∫_(1/( (√3))) ^1 ((arctan(xt))/t)dt we have f^′ (x)= ∫_(1/( (√3))) ^1 (t/(t(1+x^2 t^2 )))dt =∫_(1/( (√3))) ^1 (dt/(1+x^2 t^2 )) =_(xt=u) ∫_(x/( (√3))) ^x (1/(1+u^2 )) (du/x) =(1/x) ∫_(x/( (√3))) ^x (du/(1+u^2 )) =(1/x)[arctan(u)]_(x/( (√3))) ^x =(1/x){arctan(x)−arctan((x/( (√3))))}⇒ f(x)= ∫_0 ^x ((arctan(t))/t)dt −∫_0 ^x ((arctan((t/( (√3)))))/t)dt +λ λ=f(0)=0 but ∫_0 ^x ((arctan((t/( (√3)))))/t)dt =_((t/( (√3)))=u) ∫_0 ^(x/( (√3))) ((arctan(u))/(u(√3))) (√3)du =∫_0 ^(x/( (√3))) ((arctan(t))/t)dt⇒ f(x)= ∫_0 ^x ((arctan(t))/t)dt−∫_0 ^(x/( (√3))) ((arctan(t))/t)dt I =2f(1) =2{ ∫_0 ^1 ((arctant)/t)dt −∫_0 ^(1/( (√3))) ((arctant)/t)dt} we have arctan^′ (t)=(1/(1+t^2 )) =Σ_(n=0) ^∞ (−1)^n t^(2n) ⇒ arctant = Σ_(n=0) ^∞ (((−1)^n )/(2n+1))t^(2n+1) ⇒ ((arctant)/t) =Σ_(n=0) ^∞ (((−1)^n )/(2n+1)) t^(2n) ⇒ ∫_0 ^1 ((arctant)/t)dt =Σ_(n=0) ^∞ (((−1)^n )/(2n+1))∫_0 ^1 t^(2n) dt =Σ_(n=0) ^∞ (((−1)^n )/((2n+1)^2 )) (sum knownby fourier serie) ∫_0 ^(1/( (√3))) ((arctant)/t)dt =Σ_(n=0) ^∞ (((−1)^n )/((n+1+))[(1/(2n+1))t^(2n+1) ]_0 ^(1/( (√3))) =Σ_(n=0) ^∞ (((−1)^n )/((2n+1)^2 )) ((1/( (√3))))^(2n+1) ...](https://www.tinkutara.com/question/Q40976.png)
$${let}\:{I}\:=\:\int_{\frac{\pi}{\mathrm{3}}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{x}}{{sinx}}{dx}\:{changement}\:{tan}\left(\frac{{x}}{\mathrm{2}}\right)={t}\:{give} \\ $$$${I}\:=\:\int_{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\:\frac{\mathrm{2}{arctant}}{\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{2}} }}\:\frac{\mathrm{2}{dt}}{\mathrm{1}+{t}^{\mathrm{2}} }\:=\mathrm{2}\:\int_{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\:\frac{{arctant}}{{t}}\:{dt} \\ $$$${let}\:{introduce}\:{the}\:{parametric}\:{function} \\ $$$${f}\left({x}\right)\:=\:\int_{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\frac{{arctan}\left({xt}\right)}{{t}}{dt}\:{we}\:{have} \\ $$$${f}^{'} \left({x}\right)=\:\int_{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\frac{{t}}{{t}\left(\mathrm{1}+{x}^{\mathrm{2}} {t}^{\mathrm{2}} \right)}{dt}\:=\int_{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} ^{\mathrm{1}} \:\:\:\frac{{dt}}{\mathrm{1}+{x}^{\mathrm{2}} {t}^{\mathrm{2}} } \\ $$$$=_{{xt}={u}} \:\:\int_{\frac{{x}}{\:\sqrt{\mathrm{3}}}} ^{{x}} \:\:\:\:\frac{\mathrm{1}}{\mathrm{1}+{u}^{\mathrm{2}} }\:\frac{{du}}{{x}}\:=\frac{\mathrm{1}}{{x}}\:\int_{\frac{{x}}{\:\sqrt{\mathrm{3}}}} ^{{x}} \:\frac{{du}}{\mathrm{1}+{u}^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{{x}}\left[{arctan}\left({u}\right)\right]_{\frac{{x}}{\:\sqrt{\mathrm{3}}}} ^{{x}} =\frac{\mathrm{1}}{{x}}\left\{{arctan}\left({x}\right)−{arctan}\left(\frac{{x}}{\:\sqrt{\mathrm{3}}}\right)\right\}\Rightarrow \\ $$$${f}\left({x}\right)=\:\int_{\mathrm{0}} ^{{x}} \:\:\frac{{arctan}\left({t}\right)}{{t}}{dt}\:−\int_{\mathrm{0}} ^{{x}} \:\:\:\:\frac{{arctan}\left(\frac{{t}}{\:\sqrt{\mathrm{3}}}\right)}{{t}}{dt}\:+\lambda \\ $$$$\lambda={f}\left(\mathrm{0}\right)=\mathrm{0}\:\:{but}\:\int_{\mathrm{0}} ^{{x}} \:\:\frac{{arctan}\left(\frac{{t}}{\:\sqrt{\mathrm{3}}}\right)}{{t}}{dt} \\ $$$$=_{\frac{{t}}{\:\sqrt{\mathrm{3}}}={u}} \:\:\int_{\mathrm{0}} ^{\frac{{x}}{\:\sqrt{\mathrm{3}}}} \:\:\frac{{arctan}\left({u}\right)}{{u}\sqrt{\mathrm{3}}}\:\sqrt{\mathrm{3}}{du}\:=\int_{\mathrm{0}} ^{\frac{{x}}{\:\sqrt{\mathrm{3}}}} \:\:\frac{{arctan}\left({t}\right)}{{t}}{dt}\Rightarrow \\ $$$${f}\left({x}\right)=\:\int_{\mathrm{0}} ^{{x}} \:\:\frac{{arctan}\left({t}\right)}{{t}}{dt}−\int_{\mathrm{0}} ^{\frac{{x}}{\:\sqrt{\mathrm{3}}}} \:\:\frac{{arctan}\left({t}\right)}{{t}}{dt} \\ $$$${I}\:=\mathrm{2}{f}\left(\mathrm{1}\right)\:=\mathrm{2}\left\{\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{arctant}}{{t}}{dt}\:−\int_{\mathrm{0}} ^{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} \:\frac{{arctant}}{{t}}{dt}\right\} \\ $$$${we}\:{have}\:{arctan}^{'} \left({t}\right)=\frac{\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{2}} }\:=\sum_{{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{{n}} {t}^{\mathrm{2}{n}} \Rightarrow \\ $$$${arctant}\:=\:\sum_{{n}=\mathrm{0}} ^{\infty} \frac{\left(−\mathrm{1}\right)^{{n}} }{\mathrm{2}{n}+\mathrm{1}}{t}^{\mathrm{2}{n}+\mathrm{1}} \Rightarrow \\ $$$$\frac{{arctant}}{{t}}\:=\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{\mathrm{2}{n}+\mathrm{1}}\:{t}^{\mathrm{2}{n}} \Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{arctant}}{{t}}{dt}\:=\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{\mathrm{2}{n}+\mathrm{1}}\int_{\mathrm{0}} ^{\mathrm{1}} \:{t}^{\mathrm{2}{n}} {dt} \\ $$$$=\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{\left(\mathrm{2}{n}+\mathrm{1}\right)^{\mathrm{2}} }\:\left({sum}\:{knownby}\:{fourier}\:{serie}\right) \\ $$$$\int_{\mathrm{0}} ^{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} \:\frac{{arctant}}{{t}}{dt}\:=\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{\left({n}+\mathrm{1}+\right.}\left[\frac{\mathrm{1}}{\mathrm{2}{n}+\mathrm{1}}{t}^{\mathrm{2}{n}+\mathrm{1}} \right]_{\mathrm{0}} ^{\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}} \\ $$$$=\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{\left(\mathrm{2}{n}+\mathrm{1}\right)^{\mathrm{2}} }\:\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}\right)^{\mathrm{2}{n}+\mathrm{1}} \:… \\ $$$$ \\ $$$$ \\ $$