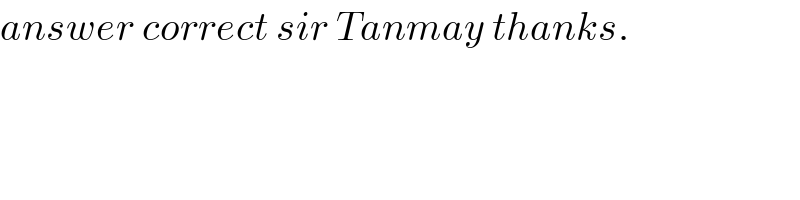calculate-0-pi-3-sinxdx-cosx-2-ln-cosx-

Question Number 39383 by maxmathsup by imad last updated on 05/Jul/18

c a l c u l a t e \int_{0}^{\frac{π}{3}} \frac{s i n x d x}{c o s x (2 + l n (c o s x)} .

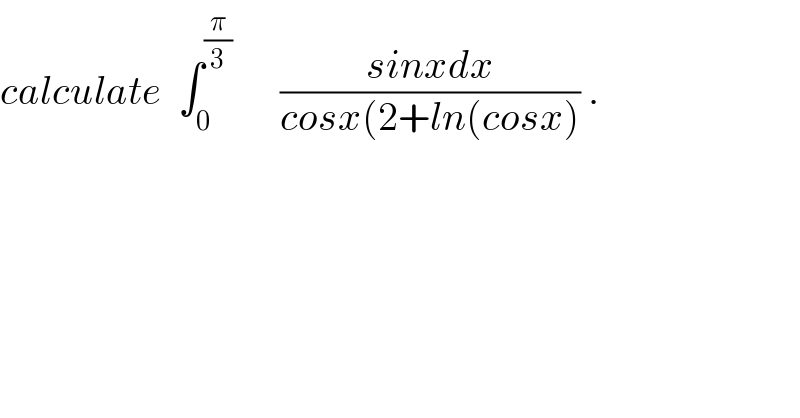
Commented by math khazana by abdo last updated on 06/Jul/18

c h a n v e m e n t c o s x = t g i v e

I = \int_{1}^{\frac{1}{2}} \frac{\sqrt{1 - t^{2}}}{t (2 + l n (t))} \frac{- d t}{\sqrt{1 - t^{2}}}

= \int_{\frac{1}{2}}^{1} \frac{d t}{t (2 + l n (t))} t h e n w e d o t h e c h a n g .

l n (t) = u \Rightarrow I = \int_{- l n (2)}^{0} \frac{e^{u} d u}{e^{u} (2 + u)}

= \int_{- l n (2)}^{0} \frac{d u}{2 + u} = {[l n ∣ 2 + u ∣]}_{- l n (2)}^{0}

= l n (2) - l n (2 - l n (2)) .

Answered by tanmay.chaudhury50@gmail.com last updated on 06/Jul/18

t = 2 + l n c o s x

t = 2 w h e n x = 0

t = 2 + l n (\frac{1}{2}) = 2 - l n 2

d t = \frac{- s i n x}{c o s x} d x

\int_{2}^{2 - l n 2} \frac{- d t}{t}

- ∣ l n t ∣_{2}^{2 - l n 2}

- l n ∣ \frac{2 - l n 2}{2} ∣

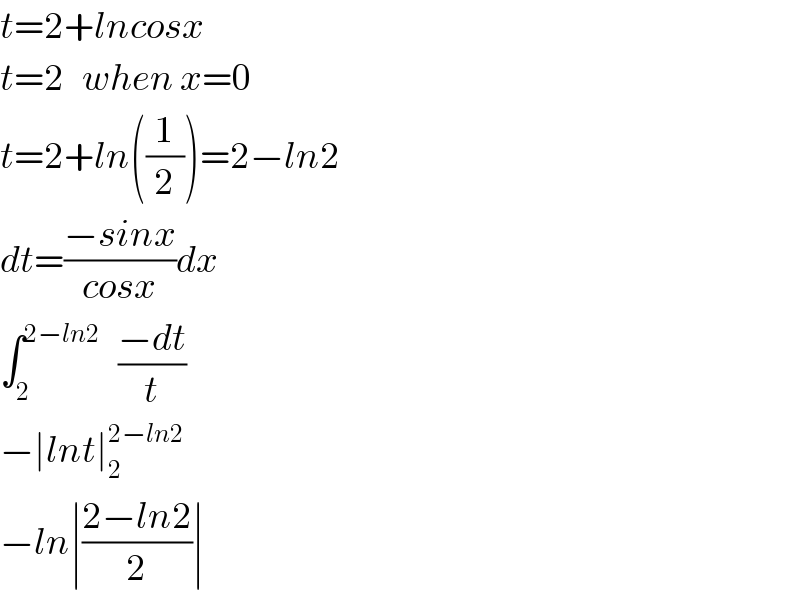
Commented by math khazana by abdo last updated on 06/Jul/18

a n s w e r c o r r e c t s i r T a n m a y t h a n k s .