Menu Close

calculate-0-sin-x-x-dx-

Tinku Tara 0 Comments
Pin




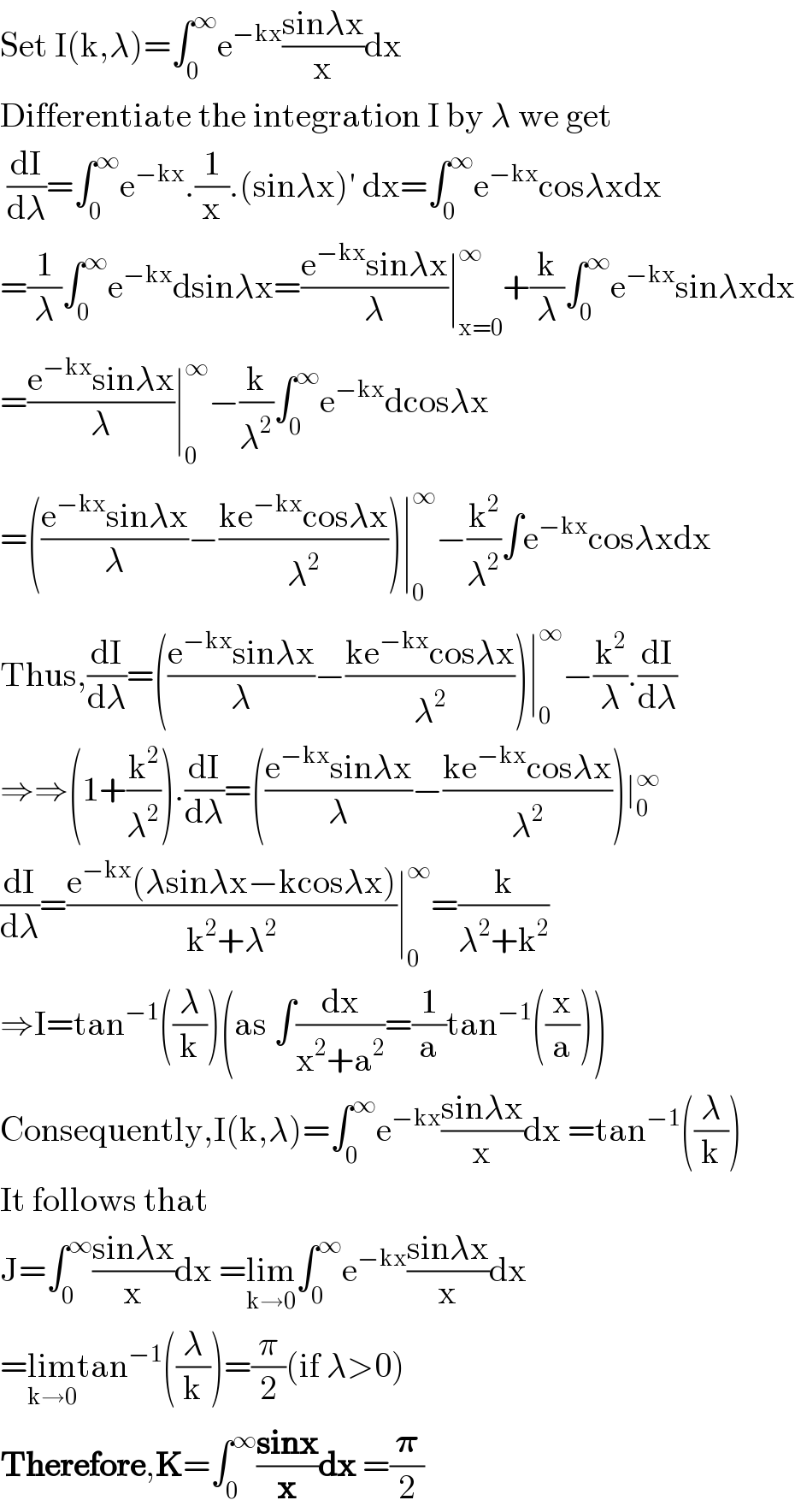
Question Number 105545 by 4635 last updated on 29/Jul/20
calculate ∫_0 ^∞ ((sin x)/x)dx
$${calculate}\:\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sin}\:{x}}{{x}}{dx} \\ $$
Answered by Ar Brandon last updated on 29/Jul/20
Let f(a)=∫_0 ^∞ ((sinx)/x)∙e^(−ax) dx⇒f ′(a)=−∫_0 ^∞ sinx∙e^(−ax) dx Let; p=∫_0 ^∞ cosx∙e^(−ax) dx , q=∫_0 ^∞ sinx∙e^(−ax) dx ⇒p−iq=∫_0 ^∞ (cosx−isinx)e^(−ax) dx=∫_0 ^∞ e^(−ix−ax) dx=∫_0 ^∞ e^(−(a+i)x) dx =−(1/(a+i))∙[e^(−(a+i)x) ]_0 ^∞ =(1/(a+i)) {lim_(x→∞) e^(−(a+i)x) =0} =((a−i)/(a^2 +1))=(a/(a^2 +1))−i((1/(a^2 +1))) ⇒ Im(p−iq)=Im{(a/(a^2 +1))−i((1/(a^2 +1)))}⇒q=(1/(a^2 +1)) ⇒f ′(a)=−q=−(1/(a^2 +1))⇒f(a)=−[Arctan(a)+C] But f(a)=∫_0 ^∞ ((sinx)/x)∙e^(−ax) dx , lim_(a→+∞) f(a)=0 ⇒lim_(a→+∞) [Arctan(a)+C]=0⇒C=−(π/2) f(0)=∫_0 ^∞ ((sinx)/x)dx=−[Arctan(0)+C]=(π/2) Hence ∫_0 ^∞ ((sinx)/x)dx=(π/2)
$$\mathrm{Let}\:\mathrm{f}\left(\mathrm{a}\right)=\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sinx}}{\mathrm{x}}\centerdot\mathrm{e}^{−\mathrm{ax}} \mathrm{dx}\Rightarrow\mathrm{f}\:'\left(\mathrm{a}\right)=−\int_{\mathrm{0}} ^{\infty} \mathrm{sinx}\centerdot\mathrm{e}^{−\mathrm{ax}} \mathrm{dx} \\ $$$$\mathrm{Let};\:\mathrm{p}=\int_{\mathrm{0}} ^{\infty} \mathrm{cosx}\centerdot\mathrm{e}^{−\mathrm{ax}} \mathrm{dx}\:,\:\mathrm{q}=\int_{\mathrm{0}} ^{\infty} \mathrm{sinx}\centerdot\mathrm{e}^{−\mathrm{ax}} \mathrm{dx} \\ $$$$\Rightarrow\mathrm{p}−\mathrm{iq}=\int_{\mathrm{0}} ^{\infty} \left(\mathrm{cosx}−\mathrm{isinx}\right)\mathrm{e}^{−\mathrm{ax}} \mathrm{dx}=\int_{\mathrm{0}} ^{\infty} \mathrm{e}^{−\mathrm{ix}−\mathrm{ax}} \mathrm{dx}=\int_{\mathrm{0}} ^{\infty} \mathrm{e}^{−\left(\mathrm{a}+\mathrm{i}\right)\mathrm{x}} \mathrm{dx} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=−\frac{\mathrm{1}}{\mathrm{a}+\mathrm{i}}\centerdot\left[\mathrm{e}^{−\left(\mathrm{a}+\mathrm{i}\right)\mathrm{x}} \right]_{\mathrm{0}} ^{\infty} =\frac{\mathrm{1}}{\mathrm{a}+\mathrm{i}}\:\:\:\left\{\underset{\mathrm{x}\rightarrow\infty} {\mathrm{lim}e}^{−\left(\mathrm{a}+\mathrm{i}\right)\mathrm{x}} =\mathrm{0}\right\} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{a}−\mathrm{i}}{\mathrm{a}^{\mathrm{2}} +\mathrm{1}}=\frac{\mathrm{a}}{\mathrm{a}^{\mathrm{2}} +\mathrm{1}}−\mathrm{i}\left(\frac{\mathrm{1}}{\mathrm{a}^{\mathrm{2}} +\mathrm{1}}\right) \\ $$$$\Rightarrow\:{Im}\left(\mathrm{p}−\mathrm{iq}\right)={Im}\left\{\frac{\mathrm{a}}{\mathrm{a}^{\mathrm{2}} +\mathrm{1}}−\mathrm{i}\left(\frac{\mathrm{1}}{\mathrm{a}^{\mathrm{2}} +\mathrm{1}}\right)\right\}\Rightarrow\mathrm{q}=\frac{\mathrm{1}}{\mathrm{a}^{\mathrm{2}} +\mathrm{1}} \\ $$$$\Rightarrow\mathrm{f}\:'\left(\mathrm{a}\right)=−\mathrm{q}=−\frac{\mathrm{1}}{\mathrm{a}^{\mathrm{2}} +\mathrm{1}}\Rightarrow\mathrm{f}\left(\mathrm{a}\right)=−\left[\mathrm{Arctan}\left(\mathrm{a}\right)+\mathrm{C}\right] \\ $$$$\mathrm{But}\:\mathrm{f}\left(\mathrm{a}\right)=\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sinx}}{\mathrm{x}}\centerdot\mathrm{e}^{−\mathrm{ax}} \mathrm{dx}\:,\:\underset{\mathrm{a}\rightarrow+\infty} {\mathrm{lim}f}\left(\mathrm{a}\right)=\mathrm{0} \\ $$$$\Rightarrow\underset{\mathrm{a}\rightarrow+\infty} {\mathrm{lim}}\left[\mathrm{Arctan}\left(\mathrm{a}\right)+\mathrm{C}\right]=\mathrm{0}\Rightarrow\mathrm{C}=−\frac{\pi}{\mathrm{2}} \\ $$$$\mathrm{f}\left(\mathrm{0}\right)=\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sinx}}{\mathrm{x}}\mathrm{dx}=−\left[\mathrm{Arctan}\left(\mathrm{0}\right)+\mathrm{C}\right]=\frac{\pi}{\mathrm{2}} \\ $$$$\mathrm{Hence}\:\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sinx}}{\mathrm{x}}\mathrm{dx}=\frac{\pi}{\mathrm{2}} \\ $$
Answered by Aziztisffola last updated on 29/Jul/20
Using Laplace transforms. L{((sinx)/x)}=∫_s ^( ∞) L{sinx}dt=∫_s ^( ∞) (1/(1+t^2 ))dt =[arctan(t)]_s ^(t→∞) =(π/2)−arctan(s) then ∫_0 ^( ∞) e^(−sx) ((sin x)/x) dx=(π/2)−arctan(s) let s=0 ⇒∫_0 ^∞ ((sin x)/x)dx=(π/2)−arctan(0)=(π/2) Hence ∫_0 ^∞ ((sin x)/x)dx=(π/2)
$$\:\mathrm{Using}\:\mathrm{Laplace}\:\mathrm{transforms}. \\ $$$$\:\mathrm{L}\left\{\frac{\mathrm{sin}{x}}{{x}}\right\}=\int_{\mathrm{s}} ^{\:\infty} \mathrm{L}\left\{\mathrm{sin}{x}\right\}\mathrm{dt}=\int_{\mathrm{s}} ^{\:\infty} \frac{\mathrm{1}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\mathrm{dt} \\ $$$$=\left[\mathrm{arctan}\left(\mathrm{t}\right)\right]_{\mathrm{s}} ^{\mathrm{t}\rightarrow\infty} =\frac{\pi}{\mathrm{2}}−\mathrm{arctan}\left(\mathrm{s}\right) \\ $$$$\mathrm{then}\:\int_{\mathrm{0}} ^{\:\infty} \mathrm{e}^{−\mathrm{s}{x}} \:\frac{\mathrm{sin}\:{x}}{{x}}\:{dx}=\frac{\pi}{\mathrm{2}}−\mathrm{arctan}\left(\mathrm{s}\right) \\ $$$$\mathrm{let}\:\mathrm{s}=\mathrm{0}\:\Rightarrow\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sin}\:{x}}{{x}}{dx}=\frac{\pi}{\mathrm{2}}−\mathrm{arctan}\left(\mathrm{0}\right)=\frac{\pi}{\mathrm{2}} \\ $$$$\:\mathrm{Hence}\:\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sin}\:{x}}{{x}}{dx}=\frac{\pi}{\mathrm{2}} \\ $$
Commented by 4635 last updated on 29/Jul/20
thank you very much
$${thank}\:{you}\:{very}\:{much} \\ $$
Commented by Aziztisffola last updated on 29/Jul/20
you′re welcome
$$\mathrm{you}'\mathrm{re}\:\mathrm{welcome} \\ $$
Answered by 1549442205PVT last updated on 30/Jul/20
Set I(k,λ)=∫_0 ^∞ e^(−kx) ((sinλx)/x)dx Differentiate the integration I by λ we get (dI/dλ)=∫_0 ^∞ e^(−kx) .(1/x).(sinλx)′ dx=∫_0 ^∞ e^(−kx) cosλxdx =(1/λ)∫_0 ^∞ e^(−kx) dsinλx=((e^(−kx) sinλx)/λ)∣_(x=0) ^∞ +(k/λ)∫_0 ^∞ e^(−kx) sinλxdx =((e^(−kx) sinλx)/λ)∣_0 ^∞ −(k/λ^2 )∫_0 ^∞ e^(−kx) dcosλx =(((e^(−kx) sinλx)/λ)−((ke^(−kx) cosλx)/λ^2 ))∣_0 ^∞ −(k^2 /λ^2 )∫e^(−kx) cosλxdx Thus,(dI/dλ)=(((e^(−kx) sinλx)/λ)−((ke^(−kx) cosλx)/λ^2 ))∣_0 ^∞ −(k^2 /λ).(dI/dλ) ⇒⇒(1+(k^2 /λ^2 )).(dI/dλ)=(((e^(−kx) sinλx)/λ)−((ke^(−kx) cosλx)/λ^2 ))∣_0 ^∞ (dI/dλ)=((e^(−kx) (λsinλx−kcosλx))/(k^2 +λ^2 ))∣_0 ^∞ =(k/(λ^2 +k^2 )) ⇒I=tan^(−1) ((λ/k))(as ∫(dx/(x^2 +a^2 ))=(1/a)tan^(−1) ((x/a))) Consequently,I(k,λ)=∫_0 ^∞ e^(−kx) ((sinλx)/x)dx =tan^(−1) ((λ/k)) It follows that J=∫_0 ^∞ ((sinλx)/x)dx =lim_(k→0) ∫_0 ^∞ e^(−kx) ((sinλx)/x)dx =lim_(k→0) tan^(−1) ((λ/k))=(π/2)(if λ>0) Therefore,K=∫_0 ^∞ ((sinx)/x)dx =(𝛑/2)
$$\mathrm{Set}\:\mathrm{I}\left(\mathrm{k},\lambda\right)=\int_{\mathrm{0}} ^{\infty} \mathrm{e}^{−\mathrm{kx}} \frac{\mathrm{sin}\lambda\mathrm{x}}{\mathrm{x}}\mathrm{dx}\: \\ $$$$\mathrm{Differentiate}\:\mathrm{the}\:\mathrm{integration}\:\mathrm{I}\:\mathrm{by}\:\lambda\:\mathrm{we}\:\mathrm{get} \\ $$$$\:\frac{\mathrm{dI}}{\mathrm{d}\lambda}=\int_{\mathrm{0}} ^{\infty} \mathrm{e}^{−\mathrm{kx}} .\frac{\mathrm{1}}{\mathrm{x}}.\left(\mathrm{sin}\lambda\mathrm{x}\right)'\:\mathrm{dx}=\int_{\mathrm{0}} ^{\infty} \mathrm{e}^{−\mathrm{kx}} \mathrm{cos}\lambda\mathrm{xdx} \\ $$$$=\frac{\mathrm{1}}{\lambda}\int_{\mathrm{0}} ^{\infty} \mathrm{e}^{−\mathrm{kx}} \mathrm{dsin}\lambda\mathrm{x}=\frac{\mathrm{e}^{−\mathrm{kx}} \mathrm{sin}\lambda\mathrm{x}}{\lambda}\mid_{\mathrm{x}=\mathrm{0}} ^{\infty} +\frac{\mathrm{k}}{\lambda}\int_{\mathrm{0}} ^{\infty} \mathrm{e}^{−\mathrm{kx}} \mathrm{sin}\lambda\mathrm{xdx} \\ $$$$=\frac{\mathrm{e}^{−\mathrm{kx}} \mathrm{sin}\lambda\mathrm{x}}{\lambda}\mid_{\mathrm{0}} ^{\infty} −\frac{\mathrm{k}}{\lambda^{\mathrm{2}} }\int_{\mathrm{0}} ^{\infty} \mathrm{e}^{−\mathrm{kx}} \mathrm{dcos}\lambda\mathrm{x} \\ $$$$=\left(\frac{\mathrm{e}^{−\mathrm{kx}} \mathrm{sin}\lambda\mathrm{x}}{\lambda}−\frac{\mathrm{ke}^{−\mathrm{kx}} \mathrm{cos}\lambda\mathrm{x}}{\lambda^{\mathrm{2}} }\right)\mid_{\mathrm{0}} ^{\infty} −\frac{\mathrm{k}^{\mathrm{2}} }{\lambda^{\mathrm{2}} }\int\mathrm{e}^{−\mathrm{kx}} \mathrm{cos}\lambda\mathrm{xdx} \\ $$$$\mathrm{Thus},\frac{\mathrm{dI}}{\mathrm{d}\lambda}=\left(\frac{\mathrm{e}^{−\mathrm{kx}} \mathrm{sin}\lambda\mathrm{x}}{\lambda}−\frac{\mathrm{ke}^{−\mathrm{kx}} \mathrm{cos}\lambda\mathrm{x}}{\lambda^{\mathrm{2}} }\right)\mid_{\mathrm{0}} ^{\infty} −\frac{\mathrm{k}^{\mathrm{2}} }{\lambda}.\frac{\mathrm{dI}}{\mathrm{d}\lambda} \\ $$$$\Rightarrow\Rightarrow\left(\mathrm{1}+\frac{\mathrm{k}^{\mathrm{2}} }{\lambda^{\mathrm{2}} }\right).\frac{\mathrm{dI}}{\mathrm{d}\lambda}=\left(\frac{\mathrm{e}^{−\mathrm{kx}} \mathrm{sin}\lambda\mathrm{x}}{\lambda}−\frac{\mathrm{ke}^{−\mathrm{kx}} \mathrm{cos}\lambda\mathrm{x}}{\lambda^{\mathrm{2}} }\right)\mid_{\mathrm{0}} ^{\infty} \\ $$$$\frac{\mathrm{dI}}{\mathrm{d}\lambda}=\frac{\mathrm{e}^{−\mathrm{kx}} \left(\lambda\mathrm{sin}\lambda\mathrm{x}−\mathrm{kcos}\lambda\mathrm{x}\right)}{\mathrm{k}^{\mathrm{2}} +\lambda^{\mathrm{2}} }\mid_{\mathrm{0}} ^{\infty} =\frac{\mathrm{k}}{\lambda^{\mathrm{2}} +\mathrm{k}^{\mathrm{2}} } \\ $$$$\Rightarrow\mathrm{I}=\mathrm{tan}^{−\mathrm{1}} \left(\frac{\lambda}{\mathrm{k}}\right)\left(\mathrm{as}\:\int\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{2}} +\mathrm{a}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{a}}\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{x}}{\mathrm{a}}\right)\right) \\ $$$$\mathrm{Consequently},\mathrm{I}\left(\mathrm{k},\lambda\right)=\int_{\mathrm{0}} ^{\infty} \mathrm{e}^{−\mathrm{kx}} \frac{\mathrm{sin}\lambda\mathrm{x}}{\mathrm{x}}\mathrm{dx}\:=\mathrm{tan}^{−\mathrm{1}} \left(\frac{\lambda}{\mathrm{k}}\right) \\ $$$$\mathrm{It}\:\mathrm{follows}\:\mathrm{that}\: \\ $$$$\mathrm{J}=\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sin}\lambda\mathrm{x}}{\mathrm{x}}\mathrm{dx}\:=\underset{\mathrm{k}\rightarrow\mathrm{0}} {\mathrm{lim}}\int_{\mathrm{0}} ^{\infty} \mathrm{e}^{−\mathrm{kx}} \frac{\mathrm{sin}\lambda\mathrm{x}}{\mathrm{x}}\mathrm{dx}\: \\ $$$$=\underset{\mathrm{k}\rightarrow\mathrm{0}} {\mathrm{lim}tan}^{−\mathrm{1}} \left(\frac{\lambda}{\mathrm{k}}\right)=\frac{\pi}{\mathrm{2}}\left(\mathrm{if}\:\lambda>\mathrm{0}\right) \\ $$$$\boldsymbol{\mathrm{Therefore}},\boldsymbol{\mathrm{K}}=\int_{\mathrm{0}} ^{\infty} \frac{\boldsymbol{\mathrm{sinx}}}{\boldsymbol{\mathrm{x}}}\boldsymbol{\mathrm{dx}}\:=\frac{\boldsymbol{\pi}}{\mathrm{2}} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *