Menu Close

calculate-0-sinx-x-x-4-1-dx-

Tinku Tara 0 Comments
Pin




Question Number 126494 by mathmax by abdo last updated on 21/Dec/20
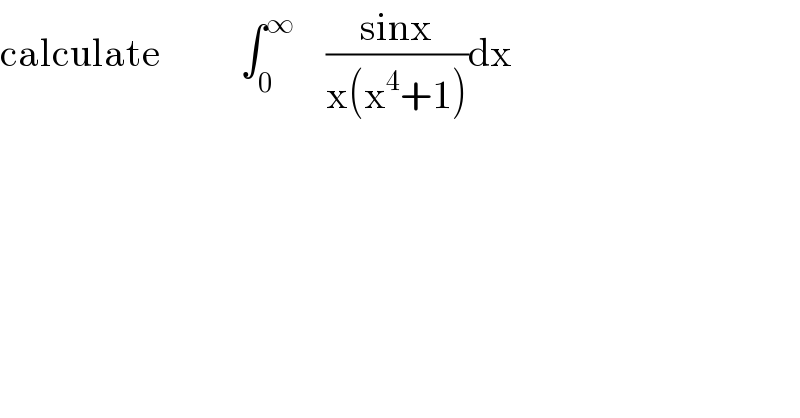
calculate ∫_0 ^∞ ((sinx)/(x(x^4 +1)))dx
$$\mathrm{calculate}\:\:\:\:\:\:\:\:\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{\mathrm{sinx}}{\mathrm{x}\left(\mathrm{x}^{\mathrm{4}} +\mathrm{1}\right)}\mathrm{dx} \\ $$
Answered by mathmax by abdo last updated on 22/Dec/20
I=∫_0 ^∞ ((sinx)/(x(x^4 +1)))dx ⇒2I=∫_(−∞) ^(+∞) ((sinx)/(x(x^4 +1)))dx =Im(∫_(−∞) ^(+∞) (e^(ix) /(x(x^4 +1)))dx) let consider the complex function ϕ(z)=(e^(iz) /(z(z^4 +1))) poles of ϕ? ϕ(z) =(e^(iz) /(z(z^2 −i)(z^2 +i))) =(e^(iz) /(z(z−e^((iπ)/4) )(z+e^((iπ)/4) )(z−e^(−((iπ)/4)) )(z+e^(−((iπ)/4)) ))) so the poles are 0,+^− e^((iπ)/(4 )) and +^− e^(−((iπ)/4)) residus theorem give ∫_(−∞) ^∞ ϕ(z)dz =2iπ{ Res(ϕ,0)+Res(ϕ,e^((iπ)/4) ) +Res(ϕ,−e^(−((iπ)/4)) )} Res(ϕ,0)=1 Res(ϕ,e^((iπ)/4) ) =(e^(ie^((iπ)/4) ) /(e^((iπ)/4) .2e^((iπ)/4) (2i))) =(e^(i((1/( (√2)))+(i/( (√2))))) /((2i)(2i)))=−(1/4) e^(−(1/( (√2)))) (cos((1/( (√2))))+isin((1/( (√2))))) Res(ϕ,−e^(−((iπ)/4)) ) =(e^(−ie^(−((iπ)/4)) ) /((−e^(−((iπ)/4)) )(−2e^(−((iπ)/4)) )(−2i))) =(e^(−ie^(−((iπ)/4)) ) /(−4i(−i))) =−(1/4) e^(−i((1/( (√2)))−(i/( (√2))))) =−(1/4)e^(−(1/( (√2)))) e^(−(i/( (√2)))) ⇒ ∫_(−∞) ^(+∞) ϕ(z)dz =2iπ{1−(1/4)e^(−(1/( (√2)))) e^(i/( (√2))) −(1/4)e^(−(1/( (√2)))) e^(−(i/( (√2)))) } =2iπ{1−(1/4)e^(−(1/( (√2)))) (e^(i/( (√2))) +e^(−(i/( (√2)))) )} =2iπ{1−(1/2) e^(−(1/( (√2)))) cos((1/( (√2))))} ⇒ 2I =2π{1−(1/2)e^(−(1/( (√2)))) cos((1/( (√2))))} ⇒I =π−(π/2)e^(−(1/( (√2)))) cos((1/( (√2))))
$$\mathrm{I}=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{sinx}}{\mathrm{x}\left(\mathrm{x}^{\mathrm{4}} +\mathrm{1}\right)}\mathrm{dx}\:\Rightarrow\mathrm{2I}=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{sinx}}{\mathrm{x}\left(\mathrm{x}^{\mathrm{4}} +\mathrm{1}\right)}\mathrm{dx}\:=\mathrm{Im}\left(\int_{−\infty} ^{+\infty} \:\frac{\mathrm{e}^{\mathrm{ix}} }{\mathrm{x}\left(\mathrm{x}^{\mathrm{4}} +\mathrm{1}\right)}\mathrm{dx}\right) \\ $$$$\mathrm{let}\:\mathrm{consider}\:\mathrm{the}\:\mathrm{complex}\:\mathrm{function}\:\varphi\left(\mathrm{z}\right)=\frac{\mathrm{e}^{\mathrm{iz}} }{\mathrm{z}\left(\mathrm{z}^{\mathrm{4}} \:+\mathrm{1}\right)}\:\mathrm{poles}\:\mathrm{of}\:\varphi? \\ $$$$\varphi\left(\mathrm{z}\right)\:=\frac{\mathrm{e}^{\mathrm{iz}} }{\mathrm{z}\left(\mathrm{z}^{\mathrm{2}} −\mathrm{i}\right)\left(\mathrm{z}^{\mathrm{2}} +\mathrm{i}\right)}\:=\frac{\mathrm{e}^{\mathrm{iz}} }{\mathrm{z}\left(\mathrm{z}−\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\left(\mathrm{z}+\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\left(\mathrm{z}−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\left(\mathrm{z}+\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)} \\ $$$$\mathrm{so}\:\mathrm{the}\:\mathrm{poles}\:\mathrm{are}\:\mathrm{0},\overset{−} {+}\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}\:}} \:\mathrm{and}\:\overset{−} {+}\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \:\:\mathrm{residus}\:\mathrm{theorem}\:\mathrm{give} \\ $$$$\int_{−\infty} ^{\infty} \:\varphi\left(\mathrm{z}\right)\mathrm{dz}\:=\mathrm{2i}\pi\left\{\:\mathrm{Res}\left(\varphi,\mathrm{0}\right)+\mathrm{Res}\left(\varphi,\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\:+\mathrm{Res}\left(\varphi,−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\right\} \\ $$$$\mathrm{Res}\left(\varphi,\mathrm{0}\right)=\mathrm{1} \\ $$$$\mathrm{Res}\left(\varphi,\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\:=\frac{\mathrm{e}^{\mathrm{ie}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} } }{\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} .\mathrm{2e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} \left(\mathrm{2i}\right)}\:=\frac{\mathrm{e}^{\mathrm{i}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}+\frac{\mathrm{i}}{\:\sqrt{\mathrm{2}}}\right)} }{\left(\mathrm{2i}\right)\left(\mathrm{2i}\right)}=−\frac{\mathrm{1}}{\mathrm{4}}\:\mathrm{e}^{−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}} \:\left(\mathrm{cos}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\right)+\mathrm{isin}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\right)\right) \\ $$$$\mathrm{Res}\left(\varphi,−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\:=\frac{\mathrm{e}^{−\mathrm{ie}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} } }{\left(−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\left(−\mathrm{2e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \right)\left(−\mathrm{2i}\right)}\:=\frac{\mathrm{e}^{−\mathrm{ie}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} } }{−\mathrm{4i}\left(−\mathrm{i}\right)} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}\:\mathrm{e}^{−\mathrm{i}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}−\frac{\mathrm{i}}{\:\sqrt{\mathrm{2}}}\right)} \:=−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{e}^{−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}} \:\:\mathrm{e}^{−\frac{\mathrm{i}}{\:\sqrt{\mathrm{2}}}} \:\:\Rightarrow\:\: \\ $$$$\int_{−\infty} ^{+\infty} \:\varphi\left(\mathrm{z}\right)\mathrm{dz}\:=\mathrm{2i}\pi\left\{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{e}^{−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}} \:\:\:\mathrm{e}^{\frac{\mathrm{i}}{\:\sqrt{\mathrm{2}}}} \:\:−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{e}^{−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}} \:\:\mathrm{e}^{−\frac{\mathrm{i}}{\:\sqrt{\mathrm{2}}}} \:\:\:\right\} \\ $$$$=\mathrm{2i}\pi\left\{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{e}^{−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}} \:\left(\mathrm{e}^{\frac{\mathrm{i}}{\:\sqrt{\mathrm{2}}}} \:\:+\mathrm{e}^{−\frac{\mathrm{i}}{\:\sqrt{\mathrm{2}}}} \:\right)\right\} \\ $$$$=\mathrm{2i}\pi\left\{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}\:\mathrm{e}^{−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}} \:\:\mathrm{cos}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\right)\right\}\:\Rightarrow \\ $$$$\mathrm{2I}\:=\mathrm{2}\pi\left\{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{e}^{−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}} \:\:\mathrm{cos}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\right)\right\}\:\Rightarrow\mathrm{I}\:=\pi−\frac{\pi}{\mathrm{2}}\mathrm{e}^{−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}} \:\:\mathrm{cos}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\right) \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *