Menu Close

calculate-arctan-cosx-sinx-x-2-4-dx-

Tinku Tara 0 Comments
Pin




Question Number 100511 by mathmax by abdo last updated on 27/Jun/20
calculate ∫_(−∞) ^∞ ((arctan(cosx +sinx))/(x^2 +4)) dx
$$\mathrm{calculate}\:\:\int_{−\infty} ^{\infty} \:\:\frac{\mathrm{arctan}\left(\mathrm{cosx}\:+\mathrm{sinx}\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{4}}\:\mathrm{dx} \\ $$
Answered by abdomathmax last updated on 28/Jun/20
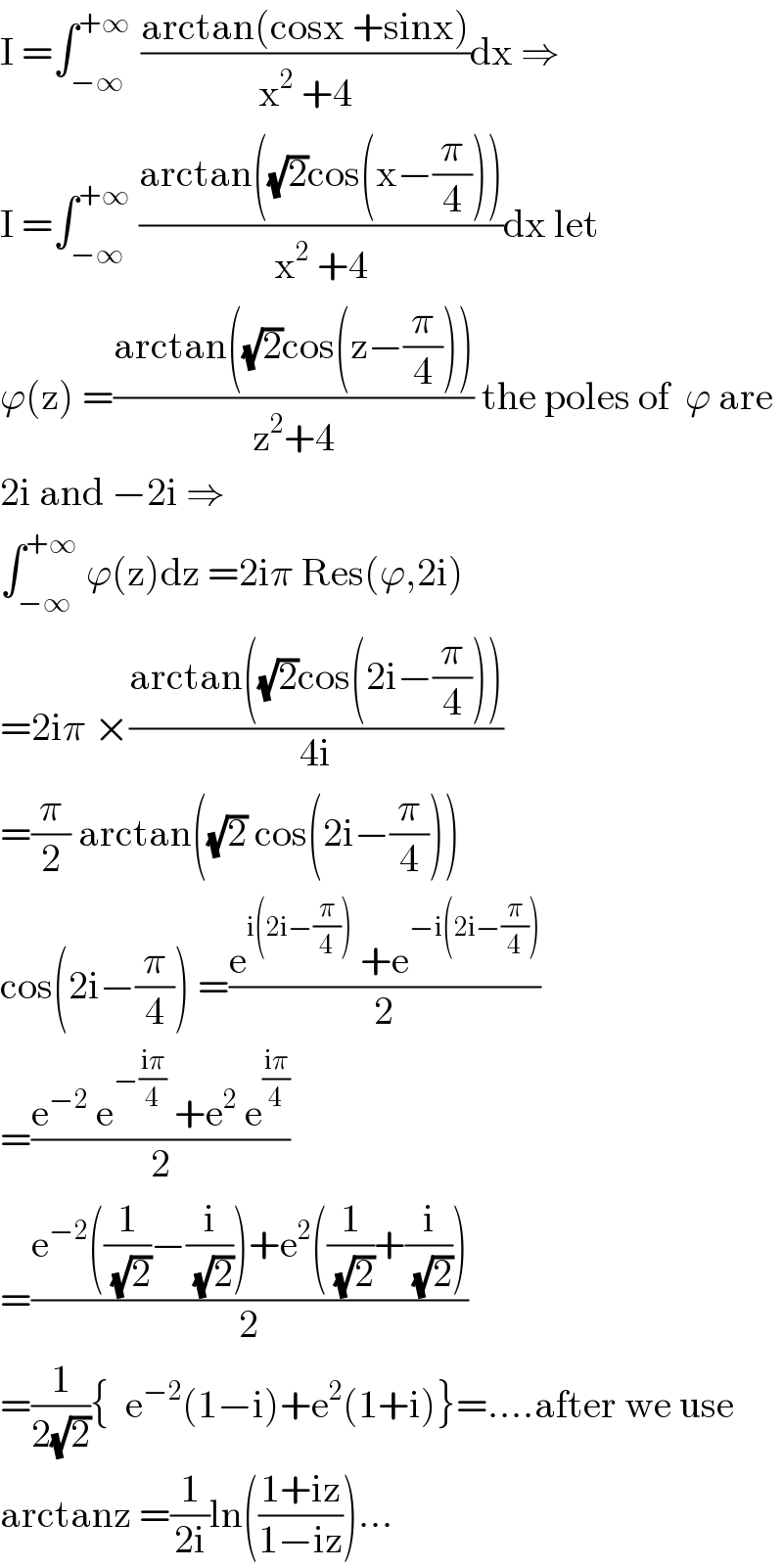
I =∫_(−∞) ^(+∞ ) ((arctan(cosx +sinx))/(x^2 +4))dx ⇒ I =∫_(−∞) ^(+∞) ((arctan((√2)cos(x−(π/4))))/(x^2 +4))dx let ϕ(z) =((arctan((√2)cos(z−(π/4))))/(z^2 +4)) the poles of ϕ are 2i and −2i ⇒ ∫_(−∞) ^(+∞) ϕ(z)dz =2iπ Res(ϕ,2i) =2iπ ×((arctan((√2)cos(2i−(π/4))))/(4i)) =(π/2) arctan((√2) cos(2i−(π/4))) cos(2i−(π/4)) =((e^(i(2i−(π/4))) +e^(−i(2i−(π/4))) )/2) =((e^(−2) e^(−((iπ)/4)) +e^2 e^((iπ)/4) )/2) =((e^(−2) ((1/( (√2)))−(i/( (√2))))+e^2 ((1/( (√2)))+(i/( (√2)))))/2) =(1/(2(√2))){ e^(−2) (1−i)+e^2 (1+i)}=....after we use arctanz =(1/(2i))ln(((1+iz)/(1−iz)))...
$$\mathrm{I}\:=\int_{−\infty} ^{+\infty\:} \:\frac{\mathrm{arctan}\left(\mathrm{cosx}\:+\mathrm{sinx}\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{4}}\mathrm{dx}\:\Rightarrow \\ $$$$\mathrm{I}\:=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{arctan}\left(\sqrt{\mathrm{2}}\mathrm{cos}\left(\mathrm{x}−\frac{\pi}{\mathrm{4}}\right)\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{4}}\mathrm{dx}\:\mathrm{let} \\ $$$$\varphi\left(\mathrm{z}\right)\:=\frac{\mathrm{arctan}\left(\sqrt{\mathrm{2}}\mathrm{cos}\left(\mathrm{z}−\frac{\pi}{\mathrm{4}}\right)\right)}{\mathrm{z}^{\mathrm{2}} +\mathrm{4}}\:\mathrm{the}\:\mathrm{poles}\:\mathrm{of}\:\:\varphi\:\mathrm{are} \\ $$$$\mathrm{2i}\:\mathrm{and}\:−\mathrm{2i}\:\Rightarrow \\ $$$$\int_{−\infty} ^{+\infty} \:\varphi\left(\mathrm{z}\right)\mathrm{dz}\:=\mathrm{2i}\pi\:\mathrm{Res}\left(\varphi,\mathrm{2i}\right) \\ $$$$=\mathrm{2i}\pi\:×\frac{\mathrm{arctan}\left(\sqrt{\mathrm{2}}\mathrm{cos}\left(\mathrm{2i}−\frac{\pi}{\mathrm{4}}\right)\right)}{\mathrm{4i}} \\ $$$$=\frac{\pi}{\mathrm{2}}\:\mathrm{arctan}\left(\sqrt{\mathrm{2}}\:\mathrm{cos}\left(\mathrm{2i}−\frac{\pi}{\mathrm{4}}\right)\right)\: \\ $$$$\mathrm{cos}\left(\mathrm{2i}−\frac{\pi}{\mathrm{4}}\right)\:=\frac{\mathrm{e}^{\mathrm{i}\left(\mathrm{2i}−\frac{\pi}{\mathrm{4}}\right)} \:+\mathrm{e}^{−\mathrm{i}\left(\mathrm{2i}−\frac{\pi}{\mathrm{4}}\right)} }{\mathrm{2}} \\ $$$$=\frac{\mathrm{e}^{−\mathrm{2}} \:\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \:+\mathrm{e}^{\mathrm{2}} \:\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{4}}} }{\mathrm{2}} \\ $$$$=\frac{\mathrm{e}^{−\mathrm{2}} \left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}−\frac{\mathrm{i}}{\:\sqrt{\mathrm{2}}}\right)+\mathrm{e}^{\mathrm{2}} \left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}+\frac{\mathrm{i}}{\:\sqrt{\mathrm{2}}}\right)}{\mathrm{2}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\left\{\:\:\mathrm{e}^{−\mathrm{2}} \left(\mathrm{1}−\mathrm{i}\right)+\mathrm{e}^{\mathrm{2}} \left(\mathrm{1}+\mathrm{i}\right)\right\}=….\mathrm{after}\:\mathrm{we}\:\mathrm{use} \\ $$$$\mathrm{arctanz}\:=\frac{\mathrm{1}}{\mathrm{2i}}\mathrm{ln}\left(\frac{\mathrm{1}+\mathrm{iz}}{\mathrm{1}−\mathrm{iz}}\right)… \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *