calculate-dx-x-1-3-x-2-3x-2-2-find-the-value-of-2-dx-x-1-3-x-2-3x-2-

Question Number 56939 by maxmathsup by imad last updated on 26/Mar/19

c a l c u l a t e \int \frac{d x}{{(x + 1)}^{3} (x^{2} - 3 x + 2)}

2) f i n d t h e v a l u e o f \int_{2}^{+ \infty} \frac{d x}{{(x + 1)}^{3} (x^{2} - 3 x + 2)}

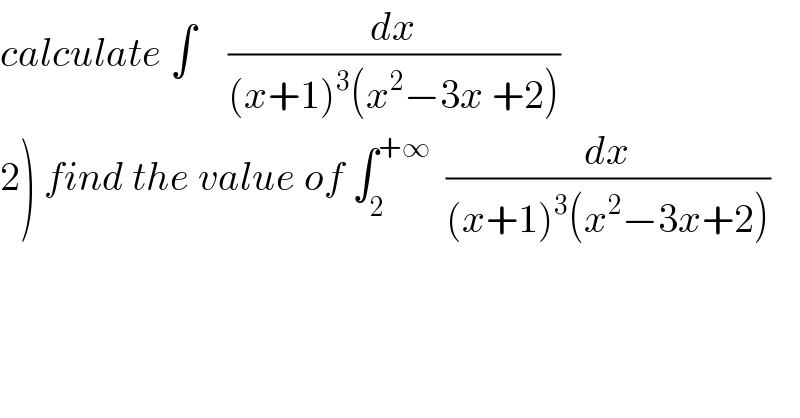
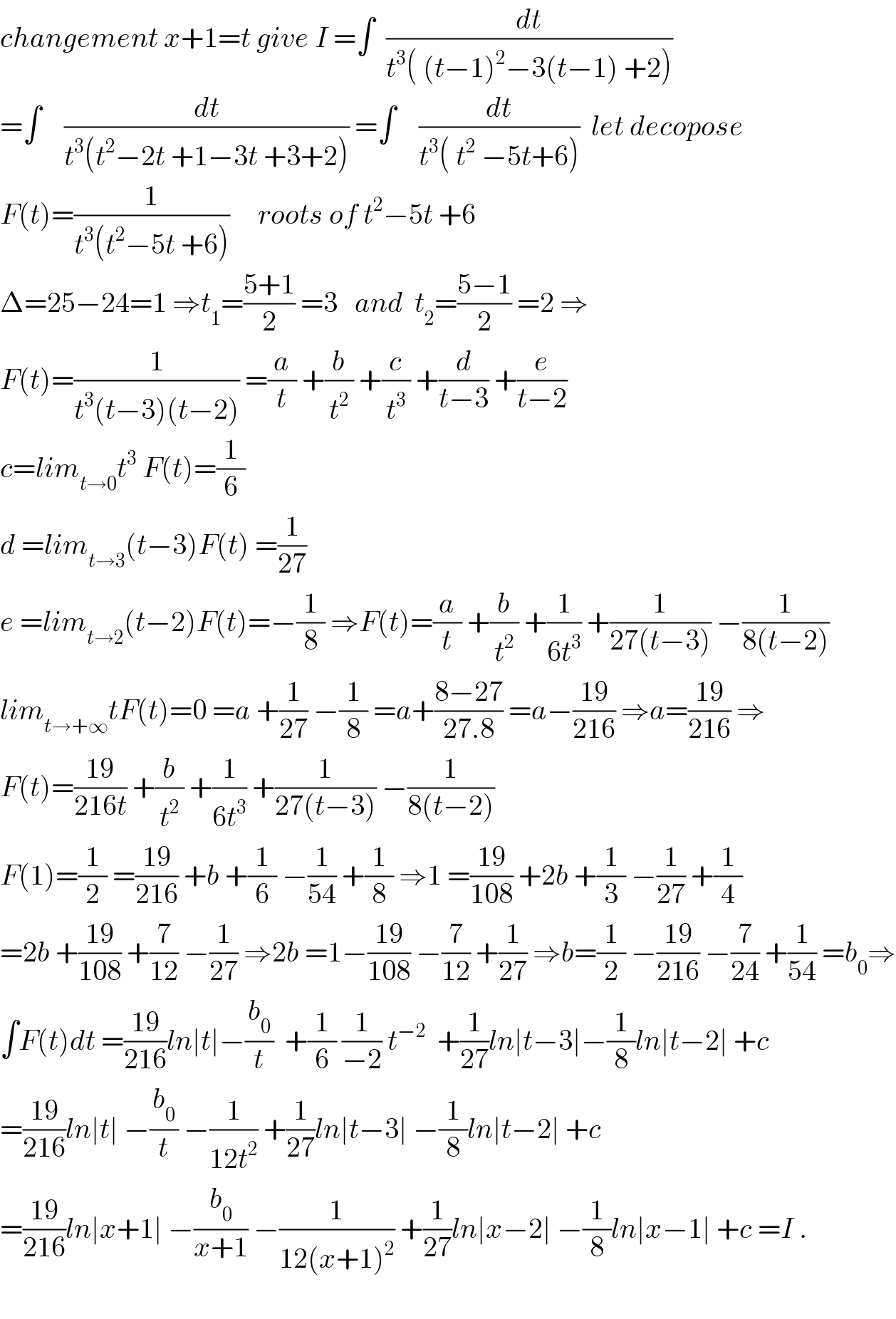
Commented by turbo msup by abdo last updated on 27/Mar/19

2) t h e q u e s t i o n i s f i n d \int_{3}^{+ \infty} \frac{d x}{{(x + 1)}^{2} (x^{2} - 3 x + 2)}

Commented by maxmathsup by imad last updated on 27/Mar/19

c h a n g e m e n t x + 1 = t g i v e I = \int \frac{d t}{t^{3} ({(t - 1)}^{2} - 3 (t - 1) + 2)}

= \int \frac{d t}{t^{3} (t^{2} - 2 t + 1 - 3 t + 3 + 2)} = \int \frac{d t}{t^{3} (t^{2} - 5 t + 6)} l e t d e c o p o s e

F (t) = \frac{1}{t^{3} (t^{2} - 5 t + 6)} r o o t s o f t^{2} - 5 t + 6

Δ = 25 - 24 = 1 \Rightarrow t_{1} = \frac{5 + 1}{2} = 3 a n d t_{2} = \frac{5 - 1}{2} = 2 \Rightarrow

F (t) = \frac{1}{t^{3} (t - 3) (t - 2)} = \frac{a}{t} + \frac{b}{t^{2}} + \frac{c}{t^{3}} + \frac{d}{t - 3} + \frac{e}{t - 2}

c = {l i m}_{t \to 0} t^{3} F (t) = \frac{1}{6}

d = {l i m}_{t \to 3} (t - 3) F (t) = \frac{1}{27}

e = {l i m}_{t \to 2} (t - 2) F (t) = - \frac{1}{8} \Rightarrow F (t) = \frac{a}{t} + \frac{b}{t^{2}} + \frac{1}{6 t^{3}} + \frac{1}{27 (t - 3)} - \frac{1}{8 (t - 2)}

{l i m}_{t \to + \infty} t F (t) = 0 = a + \frac{1}{27} - \frac{1}{8} = a + \frac{8 - 27}{27.8} = a - \frac{19}{216} \Rightarrow a = \frac{19}{216} \Rightarrow

F (t) = \frac{19}{216 t} + \frac{b}{t^{2}} + \frac{1}{6 t^{3}} + \frac{1}{27 (t - 3)} - \frac{1}{8 (t - 2)}

F (1) = \frac{1}{2} = \frac{19}{216} + b + \frac{1}{6} - \frac{1}{54} + \frac{1}{8} \Rightarrow 1 = \frac{19}{108} + 2 b + \frac{1}{3} - \frac{1}{27} + \frac{1}{4}

= 2 b + \frac{19}{108} + \frac{7}{12} - \frac{1}{27} \Rightarrow 2 b = 1 - \frac{19}{108} - \frac{7}{12} + \frac{1}{27} \Rightarrow b = \frac{1}{2} - \frac{19}{216} - \frac{7}{24} + \frac{1}{54} = b_{0} \Rightarrow

\int F (t) d t = \frac{19}{216} l n ∣ t ∣ - \frac{b_{0}}{t} + \frac{1}{6} \frac{1}{- 2} t^{- 2} + \frac{1}{27} l n ∣ t - 3 ∣ - \frac{1}{8} l n ∣ t - 2 ∣ + c

= \frac{19}{216} l n ∣ t ∣ - \frac{b_{0}}{t} - \frac{1}{12 t^{2}} + \frac{1}{27} l n ∣ t - 3 ∣ - \frac{1}{8} l n ∣ t - 2 ∣ + c

= \frac{19}{216} l n ∣ x + 1 ∣ - \frac{b_{0}}{x + 1} - \frac{1}{12 {(x + 1)}^{2}} + \frac{1}{27} l n ∣ x - 2 ∣ - \frac{1}{8} l n ∣ x - 1 ∣ + c = I .

Answered by MJS last updated on 27/Mar/19

\int \frac{d x}{{(x + 1)}^{3} (x^{2} - 3 x + 2)} = \int \frac{d x}{(x - 2) (x - 1) {(x + 1)}^{3}} =

= \int (\frac{1}{27 (x - 2)} - \frac{1}{8 (x - 1)} + \frac{19}{216 (x + 1)} + \frac{5}{36 {(x + 1)}^{2}} + \frac{1}{6 {(x + 1)}^{3}}) d x =

= \frac{1}{27} \ln ∣ x - 2 ∣ - \frac{1}{8} \ln ∣ x - 1 ∣ + \frac{19}{216} \ln ∣ x + 1 ∣ - \frac{5}{36 (x + 1)} - \frac{1}{12 {(x + 1)}^{2}} + C =

= \frac{1}{27} \ln ∣ x - 2 ∣ - \frac{1}{8} \ln ∣ x - 1 ∣ + \frac{19}{216} \ln ∣ x + 1 ∣ - \frac{5 x + 8}{36 {(x + 1)}^{2}} + C

I think the integral in [2; + \infty [{doesn}^{'} t exist

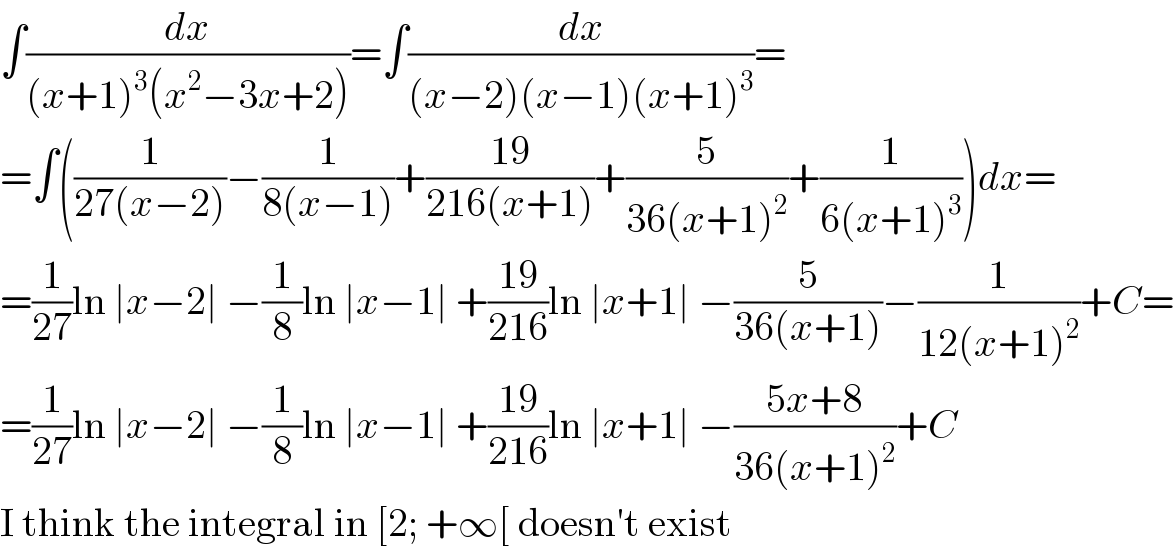
Commented by turbo msup by abdo last updated on 27/Mar/19

t h a n k y o u s i r .