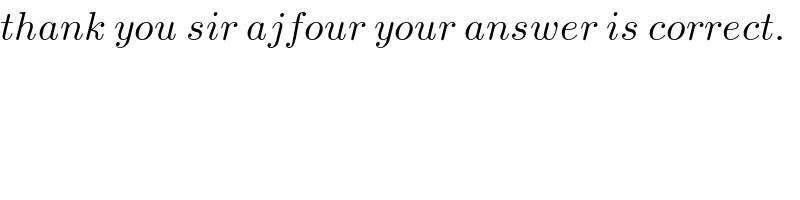Question Number 64150 by mathmax by abdo last updated on 14/Jul/19
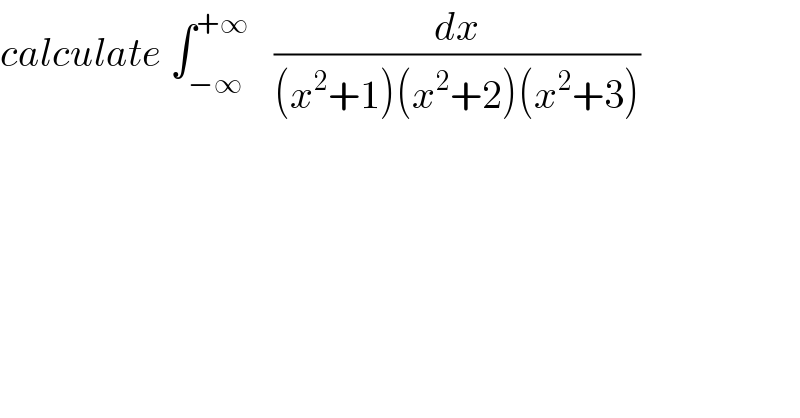
Commented by mathmax by abdo last updated on 14/Jul/19

Commented by mathmax by abdo last updated on 14/Jul/19
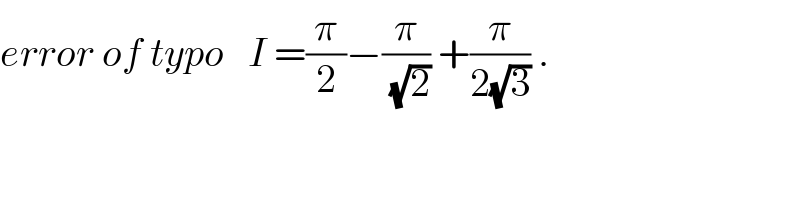
Answered by ajfour last updated on 14/Jul/19
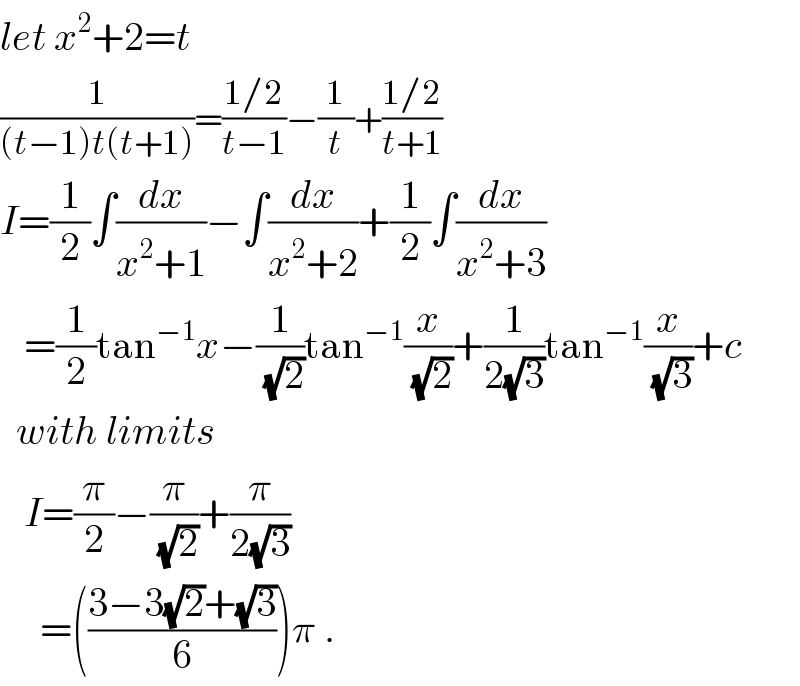
Commented by mathmax by abdo last updated on 14/Jul/19