Question Number 167882 by LEKOUMA last updated on 28/Mar/22
$${Calculate} \\ $$$${I}=\int\frac{\mathrm{1}}{{x}}\left(\sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}}\right){dx} \\ $$$${Indication}\:{poser}\:{t}=\sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}} \\ $$
Answered by ArielVyny last updated on 29/Mar/22
![t=(√((1−x)/(1+x)))→dt=((−(2/((1+x)^2 )))/(2t))dx=−(1/((1+x)^2 ))(1/t)dx t^2 =((1−x)/(1+x))→1−t^2 =x(t^2 +1)→x=((1−t^2 )/(1+t^2 )) dt=−(1/((((1+t^2 )/(1+t^2 ))+((1−t^2 )/(1+t^2 )))t))dx=−.((1+t^2 )/t)dx I=−∫((1+t^2 )/(1−t^2 )).t.(t/(1+t^2 ))dt=∫((−1−t^2 +1)/(1−t^2 ))dt I=t−∫(1/(1−t^2 ))dt t=sinu→dt=cosudu I=t−∫(1/(cos^2 u))cosudu=(√((1−x)/(1+x)))+∫(1/(cosu))du I=(√((1−x)/(1+x)))+∫((cosu)/((1−sin^2 u)))du =.....−(1/2)(−∫−((cosu)/(1−sinu))+∫((cosu)/(1+sinu))du) =.....−(1/2)[−ln(1−sinu)+ln(1+sinu)] =.....−(1/2)ln(((1+sinu)/(1−sinu))) =.....−(1/2)ln(((1+t)/(1−t)))=(√(((1−x)/(1+x))−))(1/2)ln(((1+(√((1−x)/(1+x))))/(1−(√((1−x)/(1+x)))))) I=(√(((1−x)/(1+x))−))(1/2)ln(((((√(1+x))+(√(1−x)))/( (√(1+x))))/(((√(1+x))−(√(1−x)))/( (√(1+x)))))) =(√((1−x)/(1+x)))−(1/2)ln((((√(1+x))+(√(1−x)))/( (√(1+x))−(√(1−x))))) =(√((1−x)/(1+x)))−(1/2)ln[((((√(1+x))+(√(1−x)))^2 )/(((√(1+x)))^2 −((√(1−x)))^2 ))] =(√((1−x)/(1+x)))−(1/2)ln[((1+(√(1−x^2 )))/x)]](https://www.tinkutara.com/question/Q167886.png)
$${t}=\sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}}\rightarrow{dt}=\frac{−\frac{\mathrm{2}}{\left(\mathrm{1}+{x}\right)^{\mathrm{2}} }}{\mathrm{2}{t}}{dx}=−\frac{\mathrm{1}}{\left(\mathrm{1}+{x}\right)^{\mathrm{2}} }\frac{\mathrm{1}}{{t}}{dx} \\ $$$${t}^{\mathrm{2}} =\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}\rightarrow\mathrm{1}−{t}^{\mathrm{2}} ={x}\left({t}^{\mathrm{2}} +\mathrm{1}\right)\rightarrow{x}=\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$${dt}=−\frac{\mathrm{1}}{\left(\frac{\mathrm{1}+{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }+\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }\right){t}}{dx}=−.\frac{\mathrm{1}+{t}^{\mathrm{2}} }{{t}}{dx} \\ $$$${I}=−\int\frac{\mathrm{1}+{t}^{\mathrm{2}} }{\mathrm{1}−{t}^{\mathrm{2}} }.{t}.\frac{{t}}{\mathrm{1}+{t}^{\mathrm{2}} }{dt}=\int\frac{−\mathrm{1}−{t}^{\mathrm{2}} +\mathrm{1}}{\mathrm{1}−{t}^{\mathrm{2}} }{dt} \\ $$$${I}={t}−\int\frac{\mathrm{1}}{\mathrm{1}−{t}^{\mathrm{2}} }{dt} \\ $$$${t}={sinu}\rightarrow{dt}={cosudu} \\ $$$${I}={t}−\int\frac{\mathrm{1}}{{cos}^{\mathrm{2}} {u}}{cosudu}=\sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}}+\int\frac{\mathrm{1}}{{cosu}}{du} \\ $$$${I}=\sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}}+\int\frac{{cosu}}{\left(\mathrm{1}−{sin}^{\mathrm{2}} {u}\right)}{du} \\ $$$$\:\:=…..−\frac{\mathrm{1}}{\mathrm{2}}\left(−\int−\frac{{cosu}}{\mathrm{1}−{sinu}}+\int\frac{{cosu}}{\mathrm{1}+{sinu}}{du}\right) \\ $$$$\:\:=…..−\frac{\mathrm{1}}{\mathrm{2}}\left[−{ln}\left(\mathrm{1}−{sinu}\right)+{ln}\left(\mathrm{1}+{sinu}\right)\right] \\ $$$$\:\:=…..−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\frac{\mathrm{1}+{sinu}}{\mathrm{1}−{sinu}}\right) \\ $$$$\:\:=…..−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\frac{\mathrm{1}+{t}}{\mathrm{1}−{t}}\right)=\sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}−}\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\frac{\mathrm{1}+\sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}}}{\mathrm{1}−\sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}}}\right) \\ $$$${I}=\sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}−}\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\frac{\frac{\sqrt{\mathrm{1}+{x}}+\sqrt{\mathrm{1}−{x}}}{\:\sqrt{\mathrm{1}+{x}}}}{\frac{\sqrt{\mathrm{1}+{x}}−\sqrt{\mathrm{1}−{x}}}{\:\sqrt{\mathrm{1}+{x}}}}\right) \\ $$$$\:\:=\sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}}−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\frac{\sqrt{\mathrm{1}+{x}}+\sqrt{\mathrm{1}−{x}}}{\:\sqrt{\mathrm{1}+{x}}−\sqrt{\mathrm{1}−{x}}}\right) \\ $$$$\:\:\:=\sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}}−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left[\frac{\left(\sqrt{\mathrm{1}+{x}}+\sqrt{\mathrm{1}−{x}}\right)^{\mathrm{2}} }{\left(\sqrt{\mathrm{1}+{x}}\right)^{\mathrm{2}} −\left(\sqrt{\mathrm{1}−{x}}\right)^{\mathrm{2}} }\right] \\ $$$$\:\:\:=\sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}}−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left[\frac{\mathrm{1}+\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}{{x}}\right] \\ $$$$ \\ $$
Commented by peter frank last updated on 28/Mar/22
$$\mathrm{thanks} \\ $$
Commented by MJS_new last updated on 29/Mar/22
![this is wrong. what you do in the 1^(st) and 3^(rd) lines makes no sense. t=((√(1−x))/( (√(1+x)))) ⇔ x=((1−t^2 )/(1+t^2 )) dx=(dt/((d/dx)[((√(1−x))/( (√(1+x))))]))=(dt/(−(1/( (√(1−x))(√((1+x)^3 ))))))=−(√(1−x))(√((1+x)^3 ))dt ⇒ ∫((√(1−x))/(x(√(1+x))))dx=∫((√(1−x))/(x(√(1+x))))×(−(√(1−x))(√((1+x)^3 ))dt)= =∫(((x−1)(x+1))/x)dt=∫((((−2t^2 )/(t^2 +1))×(2/(t^2 +1)))/(−((t^2 −1)/(t^2 +1))))dt= =4∫(t^2 /((t^2 −1)(t^2 +1)))dt](https://www.tinkutara.com/question/Q167913.png)
$$\mathrm{this}\:\mathrm{is}\:\mathrm{wrong}.\:\mathrm{what}\:\mathrm{you}\:\mathrm{do}\:\mathrm{in}\:\mathrm{the}\:\mathrm{1}^{\mathrm{st}} \:\mathrm{and}\:\mathrm{3}^{\mathrm{rd}} \\ $$$$\mathrm{lines}\:\mathrm{makes}\:\mathrm{no}\:\mathrm{sense}. \\ $$$${t}=\frac{\sqrt{\mathrm{1}−{x}}}{\:\sqrt{\mathrm{1}+{x}}}\:\Leftrightarrow\:{x}=\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$${dx}=\frac{{dt}}{\frac{{d}}{{dx}}\left[\frac{\sqrt{\mathrm{1}−{x}}}{\:\sqrt{\mathrm{1}+{x}}}\right]}=\frac{{dt}}{−\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}−{x}}\sqrt{\left(\mathrm{1}+{x}\right)^{\mathrm{3}} }}}=−\sqrt{\mathrm{1}−{x}}\sqrt{\left(\mathrm{1}+{x}\right)^{\mathrm{3}} }{dt} \\ $$$$\Rightarrow \\ $$$$\int\frac{\sqrt{\mathrm{1}−{x}}}{{x}\sqrt{\mathrm{1}+{x}}}{dx}=\int\frac{\sqrt{\mathrm{1}−{x}}}{{x}\sqrt{\mathrm{1}+{x}}}×\left(−\sqrt{\mathrm{1}−{x}}\sqrt{\left(\mathrm{1}+{x}\right)^{\mathrm{3}} }{dt}\right)= \\ $$$$=\int\frac{\left({x}−\mathrm{1}\right)\left({x}+\mathrm{1}\right)}{{x}}{dt}=\int\frac{\frac{−\mathrm{2}{t}^{\mathrm{2}} }{{t}^{\mathrm{2}} +\mathrm{1}}×\frac{\mathrm{2}}{{t}^{\mathrm{2}} +\mathrm{1}}}{−\frac{{t}^{\mathrm{2}} −\mathrm{1}}{{t}^{\mathrm{2}} +\mathrm{1}}}{dt}= \\ $$$$=\mathrm{4}\int\frac{{t}^{\mathrm{2}} }{\left({t}^{\mathrm{2}} −\mathrm{1}\right)\left({t}^{\mathrm{2}} +\mathrm{1}\right)}{dt} \\ $$
Commented by ArielVyny last updated on 29/Mar/22
$${yes}\:{you}\:{have}\:{right}.\:{i}\:{have}\:{forgot}\:{tne}\:{square} \\ $$
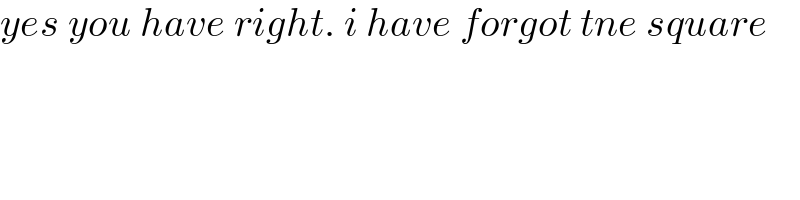
Answered by MJS_new last updated on 28/Mar/22
![∫((√(1−x))/(x(√(1+x))))dx= [t=((√(1−x))/( (√(1+x)))) → dx=−(√(1−x))(√((1+x)^3 ))dt] =4∫(t^2 /(t^4 −1))dt=∫((2/(t^2 +1))+(1/(t−1))−(1/(t+1)))dt= =2arctan t +ln ((t−1)/(t+1)) = =2arctan ((√(1−x))/( (√(1+x)))) +ln ∣((1−(√(1−x^2 )))/x)∣ +C](https://www.tinkutara.com/question/Q167895.png)
$$\int\frac{\sqrt{\mathrm{1}−{x}}}{{x}\sqrt{\mathrm{1}+{x}}}{dx}= \\ $$$$\:\:\:\:\:\left[{t}=\frac{\sqrt{\mathrm{1}−{x}}}{\:\sqrt{\mathrm{1}+{x}}}\:\rightarrow\:{dx}=−\sqrt{\mathrm{1}−{x}}\sqrt{\left(\mathrm{1}+{x}\right)^{\mathrm{3}} }{dt}\right] \\ $$$$=\mathrm{4}\int\frac{{t}^{\mathrm{2}} }{{t}^{\mathrm{4}} −\mathrm{1}}{dt}=\int\left(\frac{\mathrm{2}}{{t}^{\mathrm{2}} +\mathrm{1}}+\frac{\mathrm{1}}{{t}−\mathrm{1}}−\frac{\mathrm{1}}{{t}+\mathrm{1}}\right){dt}= \\ $$$$=\mathrm{2arctan}\:{t}\:+\mathrm{ln}\:\frac{{t}−\mathrm{1}}{{t}+\mathrm{1}}\:= \\ $$$$=\mathrm{2arctan}\:\frac{\sqrt{\mathrm{1}−{x}}}{\:\sqrt{\mathrm{1}+{x}}}\:+\mathrm{ln}\:\mid\frac{\mathrm{1}−\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}{{x}}\mid\:+{C} \\ $$