Calculate-i-cos-arctan-x-ii-cos-arcsin-x-iii-tan-arcsin-x-

Question Number 93239 by Ar Brandon last updated on 11/May/20

Calculate;

i) \cos (\arctan x)

i i) \cos (\arcsin x)

i i i) \tan (\arcsin x)

Commented by mathmax by abdo last updated on 12/May/20

1) v h a n g e m e n t a r c t a n x = t g i v e x = t a n t \Rightarrow

c o s (a r c t a n x) = c o s t = \sqrt{\frac{1}{1 + {t a n}^{2} t}} = \frac{1}{\sqrt{1 + x^{2}}}

2) l e t a r c s i n x = u \Rightarrow x = s i n u \Rightarrow

c o s (a r c s i n x) = c o s u = \sqrt{1 - {s i n}^{2} u} = \sqrt{1 - x^{2}}

3) l e t a r c s i n x = u \Rightarrow x = s i n u \Rightarrow t a n (a r c s i n x) = t a n u

= \frac{s i n u}{c o s u} = \frac{s i n u}{\sqrt{1 - {s i n}^{2} u}} = \frac{x}{\sqrt{1 - x^{2}}}

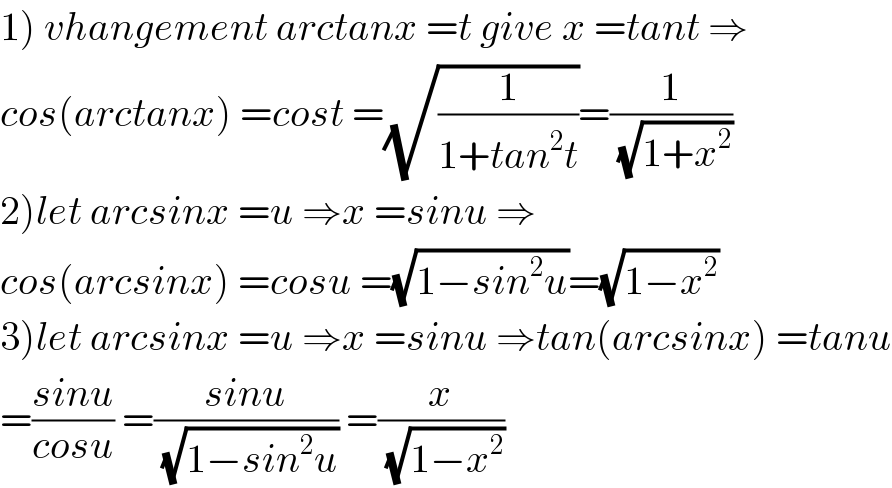
Commented by Ar Brandon last updated on 12/May/20

Thanks Mathmax ��

Commented by mathmax by abdo last updated on 12/May/20

y o u a r e w e l c o m e .

Answered by hknkrc46 last updated on 12/May/20

i) \arctan x = u \Rightarrow \tan u = x \land \cos u = h = ?

\tan u = \frac{\sin u}{\cos u} = \frac{\sqrt{1 - \cos^{2} u}}{\cos u} = \frac{\sqrt{1 - h^{2}}}{h} = x

\Rightarrow \sqrt{1 - h^{2}} = h x \Rightarrow 1 - h^{2} = h^{2} x^{2}

\Rightarrow h^{2} x^{2} + h^{2} = 1 \Rightarrow h^{2} (x^{2} + 1) = 1

\Rightarrow h^{2} = \frac{1}{x^{2} + 1} \Rightarrow h = \frac{1}{\sqrt{x^{2} + 1}}

i i) \arcsin x = u \Rightarrow \sin u = x \land \cos u = ?

\cos u = \sqrt{\cos^{2} u} = \sqrt{1 - \sin^{2} u} = \sqrt{1 - x^{2}}

i i i) \arcsin x = u \Rightarrow \sin u = x \land \tan u = h = ?

h = \tan u = \frac{\sin u}{\cos u} = \frac{\sin u}{\sqrt{1 - \sin^{2} u}} = \frac{x}{\sqrt{1 - x^{2}}}

Commented by Ar Brandon last updated on 12/May/20

hknkrc46 Thank you sir ��

Answered by Rio Michael last updated on 12/May/20

(i) \cos (\arctan x)

my approach

let \arctan x = u \Rightarrow \tan u = x

\Rightarrow \frac{\sin u}{\cos u} = x

\frac{\sin^{2} u}{\cos^{2} u} = x^{2}

\Rightarrow \frac{\sin^{2} u}{\cos^{2} u} + 1 = x^{2} + 1

hence \frac{\sin^{2} u + \cos^{2} u}{\cos^{2} u} = x^{2} + 1

\frac{1}{x^{2} + 1} = \cos^{2} u

\Rightarrow \cos u = \sqrt{\frac{1}{x^{2} + 1}} for - \frac{π}{2} ⩽ x ⩽ \frac{π}{2}

since you were not specific .

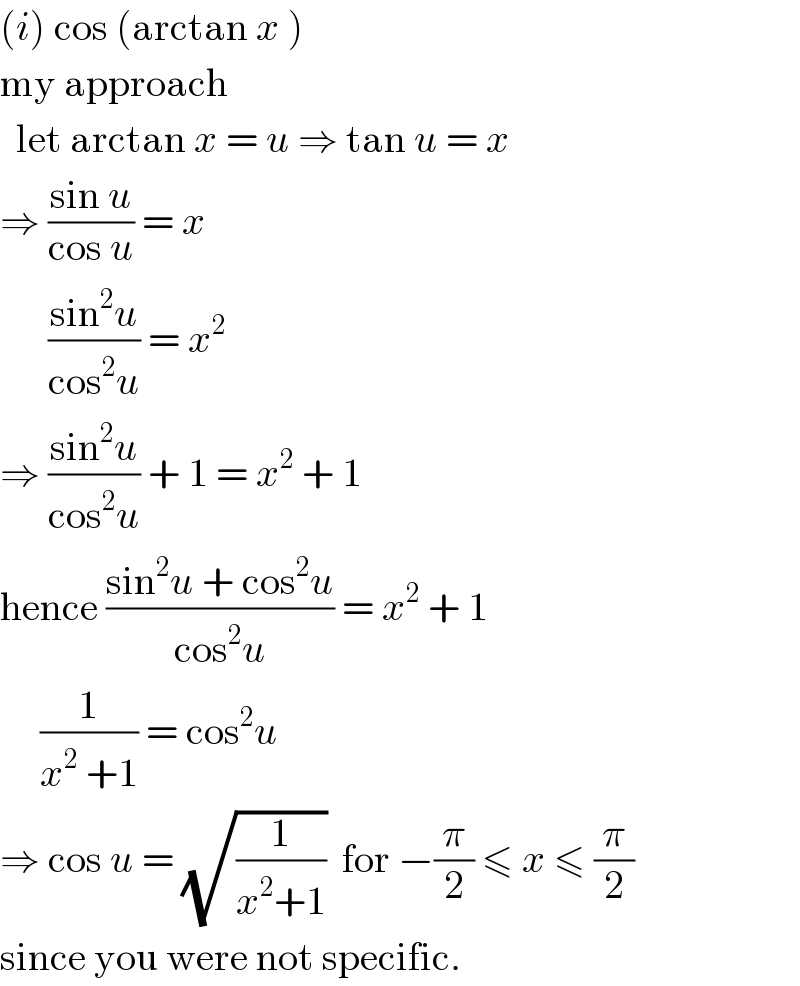
Commented by Ar Brandon last updated on 12/May/20

Thanks Mr Rio Michael. Good Day Sir ��