calculate-lim-x-0-x-x-2-ln-1-t-sin-t-dt-

Question Number 48175 by Abdo msup. last updated on 20/Nov/18

c a l c u l a t e {l i m}_{x \to 0} \int_{x}^{x^{2}} \frac{l n (1 + t)}{s i n (t)} d t

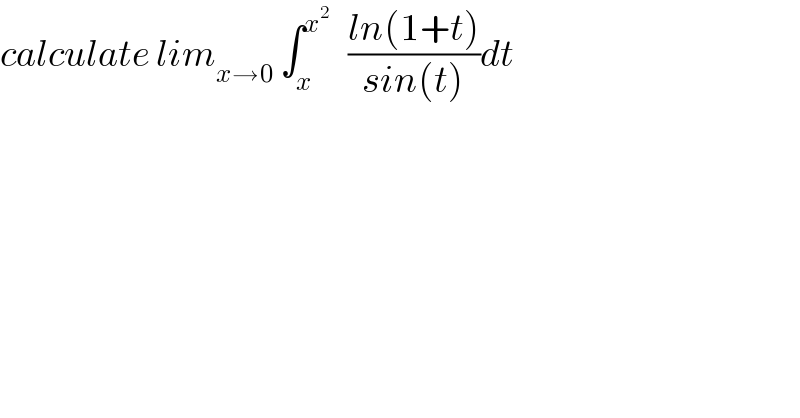
Commented by maxmathsup by imad last updated on 20/Nov/18

\exists c \in] x, x^{2} [/ A (x) = \int_{x}^{x^{2}} \frac{l n (1 + t)}{s i n (t)} d t = \frac{1}{s i n c} \int_{x}^{x^{2}} l n (1 + t) d t b u t

\int_{x}^{x^{2}} l n (1 + t) d t =_{1 + t = u} \int_{1 + x}^{1 + x^{2}} l n (u) d u = {[u l n (u) - u]}_{1 + x}^{1 + x^{2}}

= (1 + x^{2}) l n (1 + x^{2}) - (1 + x^{2}) - (1 + x) l n (1 + x) + 1 + x

\sim (1 + x^{2}) x^{2} + x - x^{2} - x (1 + x) = x^{2} + x^{4} - 2 x^{2} = x^{4} - x^{2}

x < c < x^{2} \Rightarrow \exists α / c = α x + (1 - α) x^{2} a n d s i n c \sim c \Rightarrow

\frac{x^{4} - x^{2}}{α x (1 - α) x^{2}} = \frac{x^{3} - x}{α + (1 - α) x} \to 0 s o {l i m}_{x \to 0} A (x) = 0

Commented by maxmathsup by imad last updated on 20/Nov/18

α \in] 0, 1 [.

Answered by tanmay.chaudhury50@gmail.com last updated on 20/Nov/18

I = \int_{x}^{x^{2}} \frac{l n (1 + t)}{s i n t} d t

\frac{d I}{d x} = \int_{x}^{x^{2}} \frac{\partial}{\partial x} (\frac{l n (1 + t)}{s i n t}) d t + \frac{l n (1 + x^{2})}{{s i n x}^{2}} \times \frac{{d x}^{2}}{d x} - \frac{l n (1 + x)}{s i n x} \times \frac{d x}{d x}

= 2 x \times \frac{l n (1 + x^{2})}{{s i n x}^{2}} - \frac{l n (1 + x)}{s i n x}

= 2 x \times \frac{l n (1 + x^{2})}{x^{2}} \times \frac{1}{\frac{{s i n x}^{2}}{x^{2}}} - \frac{l n (1 + x)}{x} \times \frac{1}{\frac{s i n x}{x}}

w h e n x \to 0

= 2 \times 0 \times \frac{1}{1} - 1 = - \frac{d I}{d x}

\frac{d I}{d x} = - 1 I = - x + c

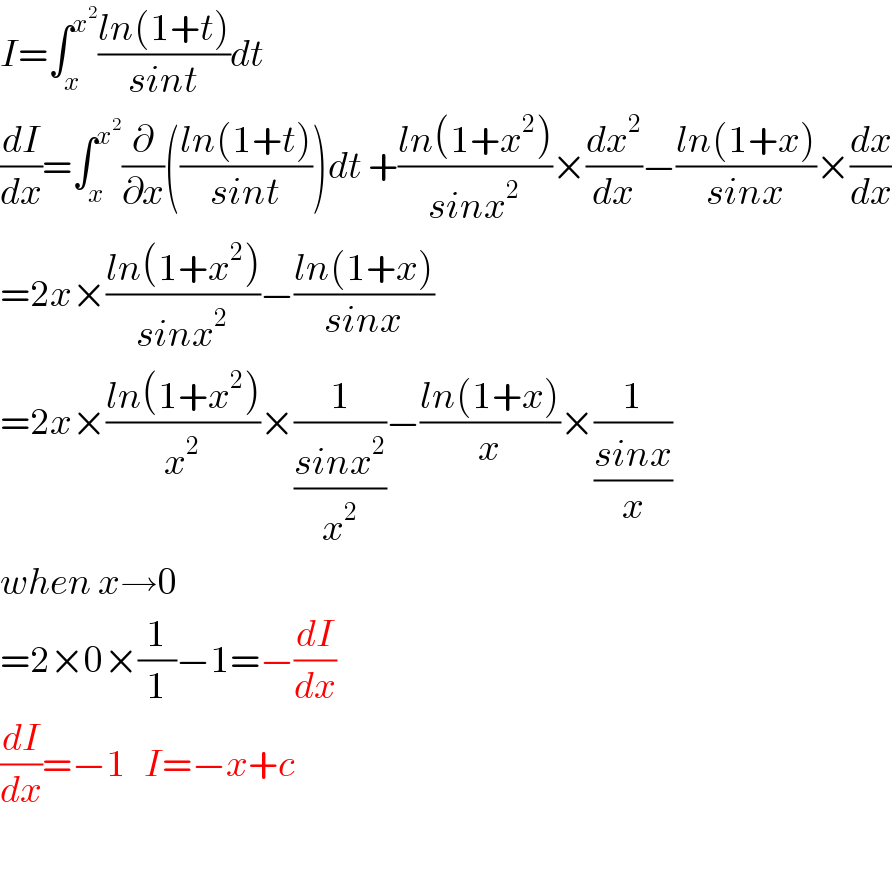
Commented by tanmay.chaudhury50@gmail.com last updated on 20/Nov/18

p l s c h e c k s i r \dots