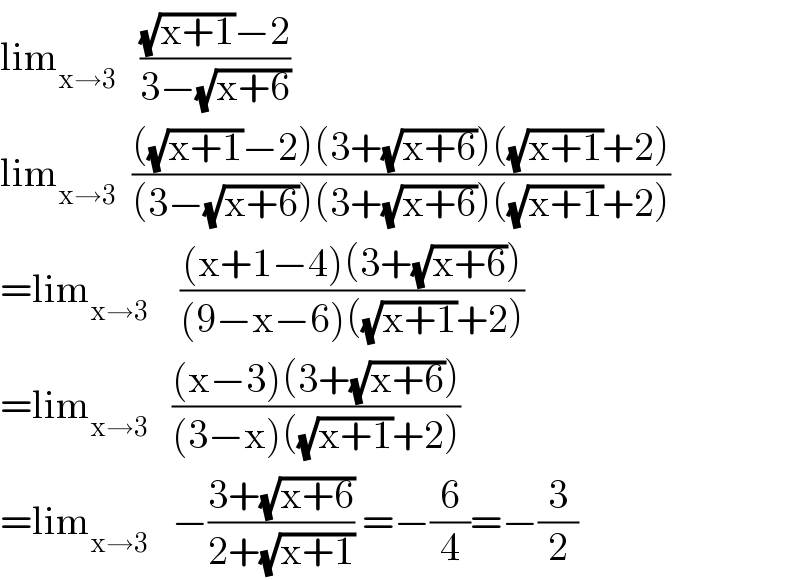calculate-lim-x-3-x-1-2-3-x-6-

Question Number 121010 by mathocean1 last updated on 04/Nov/20

calculate :

\lim_{x \to 3} \frac{\sqrt{x + 1} - 2}{3 - \sqrt{x + 6}}

Answered by 675480065 last updated on 04/Nov/20

= \lim_{x \to 3} \frac{\frac{1}{2 \sqrt{x + 1}}}{- \frac{1}{2 \sqrt{x + 6}}} = - \lim_{x \to 3} \sqrt{\frac{x + 6}{x + 1}} = - 3 / 2

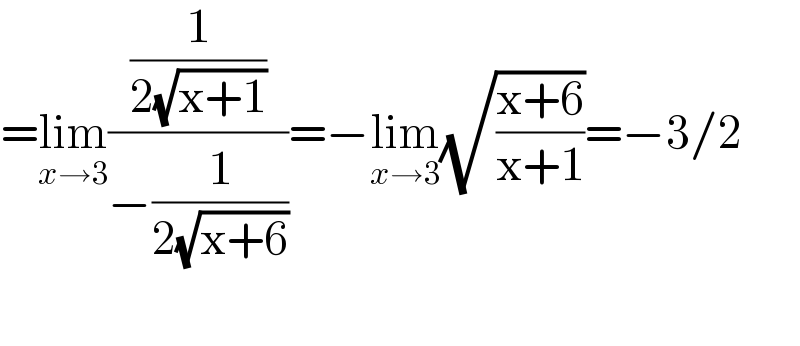
Commented by mathocean1 last updated on 04/Nov/20

please sir can you detail the

first expression

Commented by Ar Brandon last updated on 04/Nov/20

Since on evaluating we get 0/0 we can therefore apply l'hôpital's rule by differentiating the numerator and the denominator.

Answered by Ar Brandon last updated on 04/Nov/20

Υ = \lim_{x \to 3} \frac{\sqrt{x + 1} - 2}{3 - \sqrt{x + 6}}

= \lim_{x \to 3} \frac{1}{2 \sqrt{x + 1}} \cdot \frac{2 \sqrt{x + 6}}{- 1}

= - \frac{2 \sqrt{9}}{2 \sqrt{4}} = - \frac{3}{2}

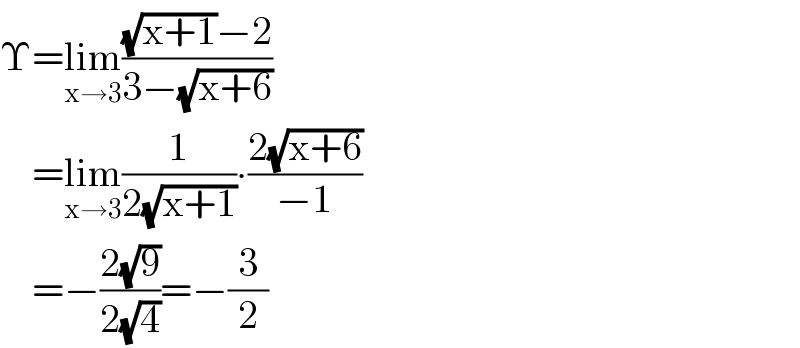
Answered by mathmax by abdo last updated on 04/Nov/20

\lim_{x \to 3} \frac{\sqrt{x + 1} - 2}{3 - \sqrt{x + 6}}

\lim_{x \to 3} \frac{(\sqrt{x + 1} - 2) (3 + \sqrt{x + 6}) (\sqrt{x + 1} + 2)}{(3 - \sqrt{x + 6}) (3 + \sqrt{x + 6}) (\sqrt{x + 1} + 2)}

= \lim_{x \to 3} \frac{(x + 1 - 4) (3 + \sqrt{x + 6})}{(9 - x - 6) (\sqrt{x + 1} + 2)}

= \lim_{x \to 3} \frac{(x - 3) (3 + \sqrt{x + 6})}{(3 - x) (\sqrt{x + 1} + 2)}

= \lim_{x \to 3} - \frac{3 + \sqrt{x + 6}}{2 + \sqrt{x + 1}} = - \frac{6}{4} = - \frac{3}{2}