Menu Close

calculate-x-2-3-x-2-x-1-3-dx-

Tinku Tara 0 Comments
Pin




Question Number 96956 by mathmax by abdo last updated on 05/Jun/20
calculate ∫_(−∞) ^∞ ((x^2 −3)/((x^2 −x+1)^3 ))dx
$$\mathrm{calculate}\:\int_{−\infty} ^{\infty} \:\:\frac{\mathrm{x}^{\mathrm{2}} −\mathrm{3}}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)^{\mathrm{3}} }\mathrm{dx} \\ $$
Answered by MJS last updated on 06/Jun/20
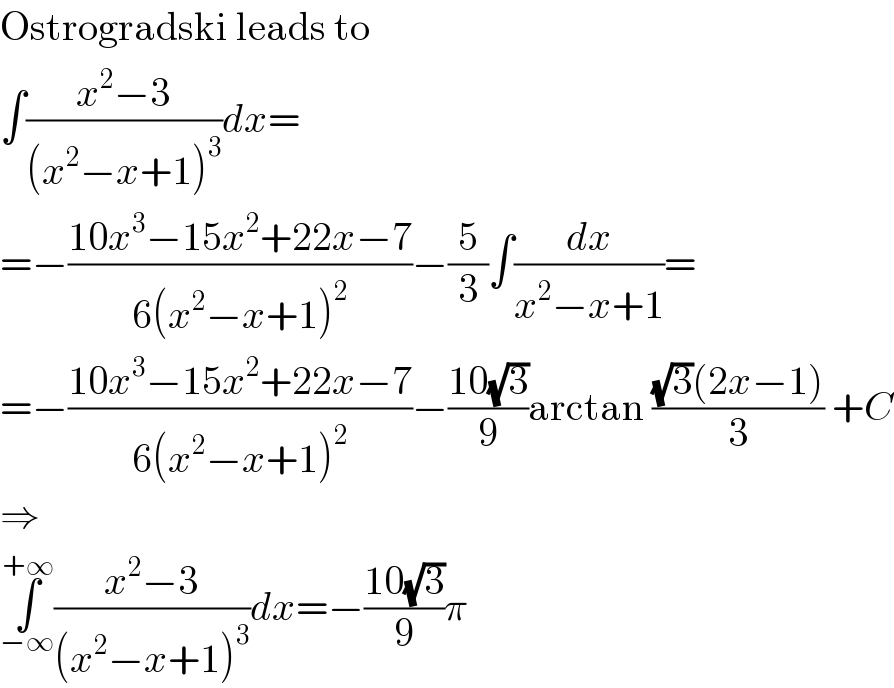
Ostrogradski leads to ∫((x^2 −3)/((x^2 −x+1)^3 ))dx= =−((10x^3 −15x^2 +22x−7)/(6(x^2 −x+1)^2 ))−(5/3)∫(dx/(x^2 −x+1))= =−((10x^3 −15x^2 +22x−7)/(6(x^2 −x+1)^2 ))−((10(√3))/9)arctan (((√3)(2x−1))/3) +C ⇒ ∫_(−∞) ^(+∞) ((x^2 −3)/((x^2 −x+1)^3 ))dx=−((10(√3))/9)π
$$\mathrm{Ostrogradski}\:\mathrm{leads}\:\mathrm{to} \\ $$$$\int\frac{{x}^{\mathrm{2}} −\mathrm{3}}{\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)^{\mathrm{3}} }{dx}= \\ $$$$=−\frac{\mathrm{10}{x}^{\mathrm{3}} −\mathrm{15}{x}^{\mathrm{2}} +\mathrm{22}{x}−\mathrm{7}}{\mathrm{6}\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)^{\mathrm{2}} }−\frac{\mathrm{5}}{\mathrm{3}}\int\frac{{dx}}{{x}^{\mathrm{2}} −{x}+\mathrm{1}}= \\ $$$$=−\frac{\mathrm{10}{x}^{\mathrm{3}} −\mathrm{15}{x}^{\mathrm{2}} +\mathrm{22}{x}−\mathrm{7}}{\mathrm{6}\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)^{\mathrm{2}} }−\frac{\mathrm{10}\sqrt{\mathrm{3}}}{\mathrm{9}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{3}}\left(\mathrm{2}{x}−\mathrm{1}\right)}{\mathrm{3}}\:+{C} \\ $$$$\Rightarrow \\ $$$$\underset{−\infty} {\overset{+\infty} {\int}}\frac{{x}^{\mathrm{2}} −\mathrm{3}}{\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)^{\mathrm{3}} }{dx}=−\frac{\mathrm{10}\sqrt{\mathrm{3}}}{\mathrm{9}}\pi \\ $$
Commented by abdomathmax last updated on 06/Jun/20
thankx sir mjs
$$\mathrm{thankx}\:\mathrm{sir}\:\mathrm{mjs} \\ $$
Answered by abdomathmax last updated on 06/Jun/20
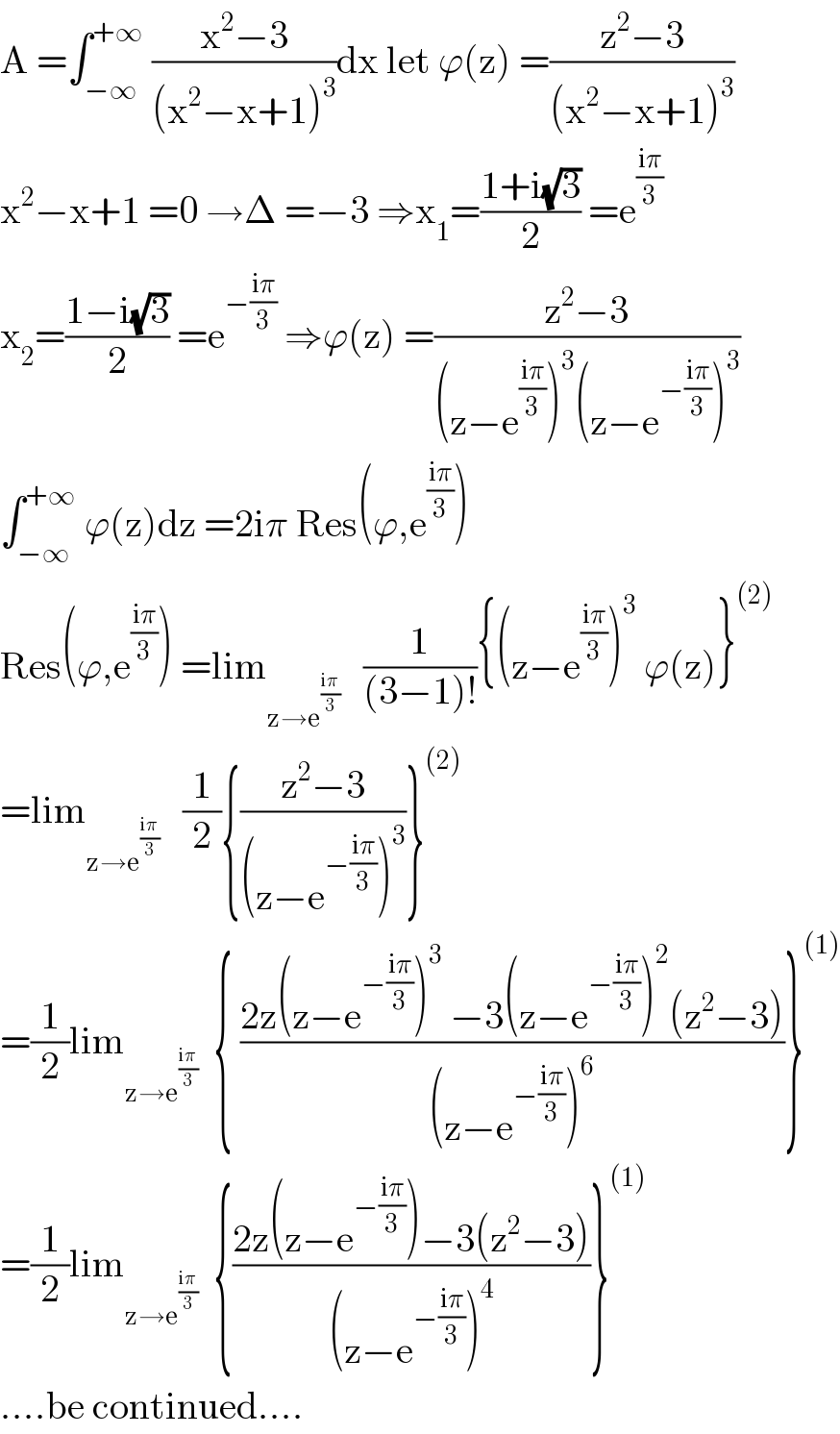
A =∫_(−∞) ^(+∞) ((x^2 −3)/((x^2 −x+1)^3 ))dx let ϕ(z) =((z^2 −3)/((x^2 −x+1)^3 )) x^2 −x+1 =0 →Δ =−3 ⇒x_1 =((1+i(√3))/2) =e^((iπ)/3) x_2 =((1−i(√3))/2) =e^(−((iπ)/3)) ⇒ϕ(z) =((z^2 −3)/((z−e^((iπ)/3) )^3 (z−e^(−((iπ)/3)) )^3 )) ∫_(−∞) ^(+∞) ϕ(z)dz =2iπ Res(ϕ,e^((iπ)/3) ) Res(ϕ,e^((iπ)/3) ) =lim_(z→e^((iπ)/3) ) (1/((3−1)!)){(z−e^((iπ)/3) )^3 ϕ(z)}^((2)) =lim_(z→e^((iπ)/3) ) (1/2){((z^2 −3)/((z−e^(−((iπ)/3)) )^3 ))}^((2)) =(1/2)lim_(z→e^((iπ)/3) ) { ((2z(z−e^(−((iπ)/3)) )^3 −3(z−e^(−((iπ)/3)) )^2 (z^2 −3))/((z−e^(−((iπ)/3)) )^6 ))}^((1)) =(1/2)lim_(z→e^((iπ)/3) ) {((2z(z−e^(−((iπ)/3)) )−3(z^2 −3))/((z−e^(−((iπ)/3)) )^4 ))}^((1)) ....be continued....
$$\mathrm{A}\:=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{x}^{\mathrm{2}} −\mathrm{3}}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)^{\mathrm{3}} }\mathrm{dx}\:\mathrm{let}\:\varphi\left(\mathrm{z}\right)\:=\frac{\mathrm{z}^{\mathrm{2}} −\mathrm{3}}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)^{\mathrm{3}} } \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\:=\mathrm{0}\:\rightarrow\Delta\:=−\mathrm{3}\:\Rightarrow\mathrm{x}_{\mathrm{1}} =\frac{\mathrm{1}+\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\:=\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \\ $$$$\mathrm{x}_{\mathrm{2}} =\frac{\mathrm{1}−\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\:=\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \:\Rightarrow\varphi\left(\mathrm{z}\right)\:=\frac{\mathrm{z}^{\mathrm{2}} −\mathrm{3}}{\left(\mathrm{z}−\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)^{\mathrm{3}} \left(\mathrm{z}−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)^{\mathrm{3}} } \\ $$$$\int_{−\infty} ^{+\infty} \:\varphi\left(\mathrm{z}\right)\mathrm{dz}\:=\mathrm{2i}\pi\:\mathrm{Res}\left(\varphi,\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \right) \\ $$$$\mathrm{Res}\left(\varphi,\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)\:=\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} } \:\:\:\frac{\mathrm{1}}{\left(\mathrm{3}−\mathrm{1}\right)!}\left\{\left(\mathrm{z}−\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)^{\mathrm{3}} \:\varphi\left(\mathrm{z}\right)\right\}^{\left(\mathrm{2}\right)} \\ $$$$=\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} } \:\:\:\frac{\mathrm{1}}{\mathrm{2}}\left\{\frac{\mathrm{z}^{\mathrm{2}} −\mathrm{3}}{\left(\mathrm{z}−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)^{\mathrm{3}} }\right\}^{\left(\mathrm{2}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} } \:\:\left\{\:\frac{\mathrm{2z}\left(\mathrm{z}−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)^{\mathrm{3}} \:−\mathrm{3}\left(\mathrm{z}−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)^{\mathrm{2}} \left(\mathrm{z}^{\mathrm{2}} −\mathrm{3}\right)}{\left(\mathrm{z}−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)^{\mathrm{6}} }\right\}^{\left(\mathrm{1}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{lim}_{\mathrm{z}\rightarrow\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} } \:\:\left\{\frac{\mathrm{2z}\left(\mathrm{z}−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)−\mathrm{3}\left(\mathrm{z}^{\mathrm{2}} −\mathrm{3}\right)}{\left(\mathrm{z}−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)^{\mathrm{4}} }\right\}^{\left(\mathrm{1}\right)} \\ $$$$….\mathrm{be}\:\mathrm{continued}…. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *