Menu Close

calculate-x-2-dx-x-2-x-1-x-2-x-1-

Tinku Tara 0 Comments
Pin




Question Number 148565 by mathmax by abdo last updated on 29/Jul/21
calculate ∫_(−∞) ^(+∞) ((x^2 dx)/((x^2 −x+1)(x^2 +x+1)))
$$\mathrm{calculate}\:\int_{−\infty} ^{+\infty} \:\:\frac{\mathrm{x}^{\mathrm{2}} \mathrm{dx}}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{x}+\mathrm{1}\right)} \\ $$
Answered by Ar Brandon last updated on 29/Jul/21
Υ=∫_(−∞) ^(+∞) ((x^2 dx)/((x^2 −x+1)(x^2 +x+1)))=2∫_0 ^∞ ((x^2 dx)/((x^2 −x+1)(x^2 +x+1))) =2∫_0 ^1 ((x^2 dx)/((x^2 −x+1)(x^2 +x+1)))+2∫_1 ^∞ ((x^2 dx)/((x^2 −x+1)(x^2 +x+1))) =2∫_0 ^1 ((x^2 dx)/((x^2 −x+1)(x^2 +x+1)))+2∫_0 ^1 (dx/((x^2 −x+1)(x^2 +x+1))) =2∫_0 ^1 ((x^2 +1)/((x^2 −x+1)(x^2 +x+1)))dx=2∫_0 ^1 ((x^2 +1)/(x^4 +x^2 +1))dx =2∫_0 ^1 ((1+(1/x^2 ))/(x^2 +1+(1/x^2 )))dx=2∫_0 ^1 ((1+(1/x^2 ))/((x−(1/x))^2 +3))dx =2∫_0 ^1 ((d(x−(1/x)))/((x−(1/x))^2 +3))=(2/( (√3)))[arctan(((x^2 −1)/( (√3)x)))]_0 ^1 =(π/( (√3)))
$$\Upsilon=\int_{−\infty} ^{+\infty} \frac{{x}^{\mathrm{2}} {dx}}{\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)}=\mathrm{2}\int_{\mathrm{0}} ^{\infty} \frac{{x}^{\mathrm{2}} {dx}}{\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)} \\ $$$$\:\:\:\:=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{2}} {dx}}{\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)}+\mathrm{2}\int_{\mathrm{1}} ^{\infty} \frac{{x}^{\mathrm{2}} {dx}}{\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)} \\ $$$$\:\:\:\:=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{2}} {dx}}{\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)}+\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{dx}}{\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)} \\ $$$$\:\:\:\:=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{2}} +\mathrm{1}}{\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)}{dx}=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{2}} +\mathrm{1}}{{x}^{\mathrm{4}} +{x}^{\mathrm{2}} +\mathrm{1}}{dx} \\ $$$$\:\:\:\:=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }}{{x}^{\mathrm{2}} +\mathrm{1}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }}{dx}=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }}{\left({x}−\frac{\mathrm{1}}{{x}}\right)^{\mathrm{2}} +\mathrm{3}}{dx} \\ $$$$\:\:\:\:=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{d}\left({x}−\frac{\mathrm{1}}{{x}}\right)}{\left({x}−\frac{\mathrm{1}}{{x}}\right)^{\mathrm{2}} +\mathrm{3}}=\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}\left[\mathrm{arctan}\left(\frac{{x}^{\mathrm{2}} −\mathrm{1}}{\:\sqrt{\mathrm{3}}{x}}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} =\frac{\pi}{\:\sqrt{\mathrm{3}}} \\ $$
Answered by mathmax by abdo last updated on 29/Jul/21
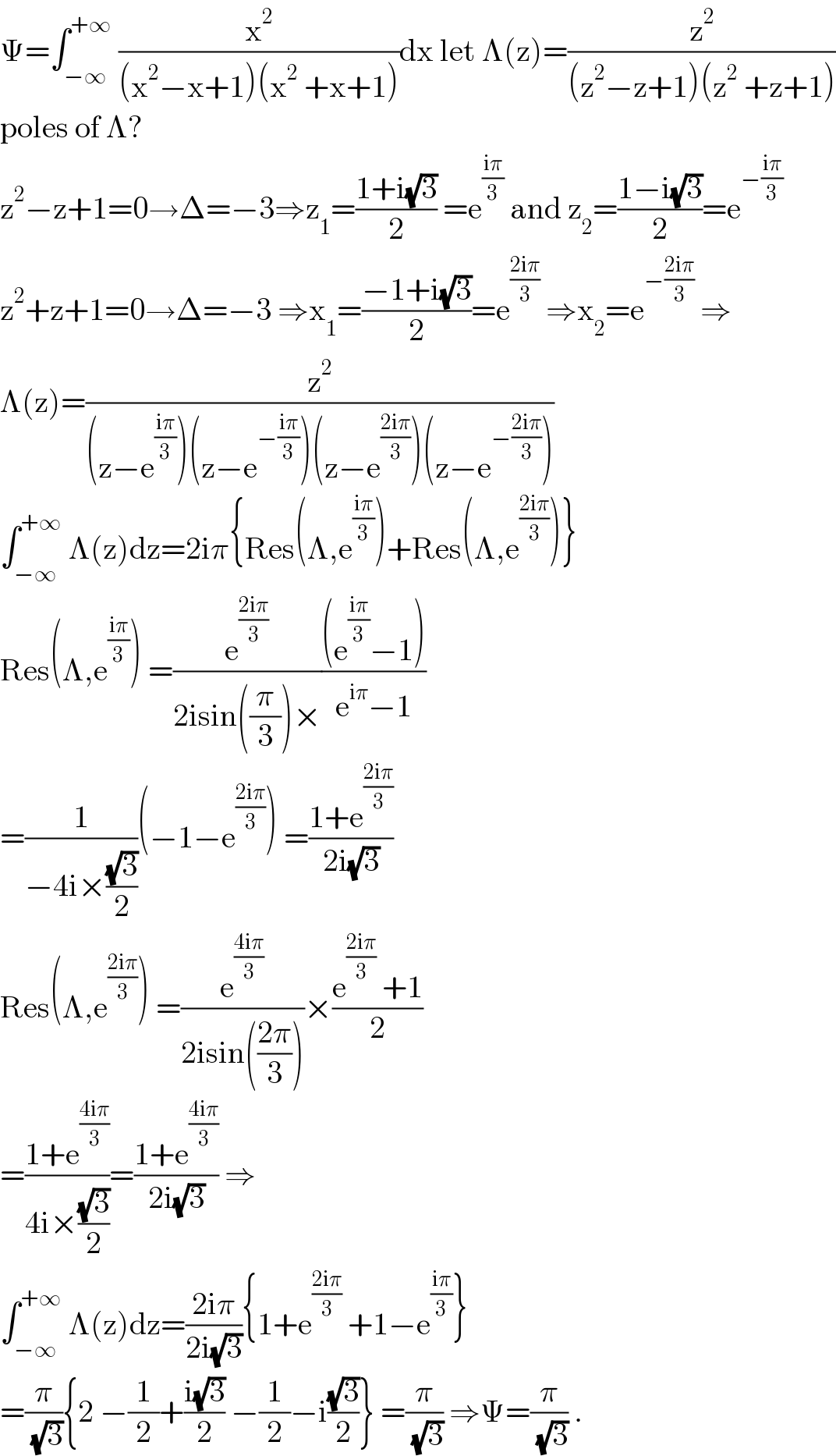
Ψ=∫_(−∞) ^(+∞) (x^2 /((x^2 −x+1)(x^2 +x+1)))dx let Λ(z)=(z^2 /((z^2 −z+1)(z^2 +z+1))) poles of Λ? z^2 −z+1=0→Δ=−3⇒z_1 =((1+i(√3))/2) =e^((iπ)/3) and z_2 =((1−i(√3))/2)=e^(−((iπ)/3)) z^2 +z+1=0→Δ=−3 ⇒x_1 =((−1+i(√3))/2)=e^((2iπ)/3) ⇒x_2 =e^(−((2iπ)/3)) ⇒ Λ(z)=(z^2 /((z−e^((iπ)/3) )(z−e^(−((iπ)/3)) )(z−e^((2iπ)/3) )(z−e^(−((2iπ)/3)) ))) ∫_(−∞) ^(+∞) Λ(z)dz=2iπ{Res(Λ,e^((iπ)/3) )+Res(Λ,e^((2iπ)/3) )} Res(Λ,e^((iπ)/3) ) =(e^((2iπ)/3) /(2isin((π/3))×))(((e^((iπ)/3) −1))/(e^(iπ) −1)) =(1/(−4i×((√3)/2)))(−1−e^((2iπ)/3) ) =((1+e^((2iπ)/3) )/(2i(√3))) Res(Λ,e^((2iπ)/3) ) =(e^((4iπ)/3) /(2isin(((2π)/3))))×((e^((2iπ)/3) +1)/2) =((1+e^((4iπ)/3) )/(4i×((√3)/2)))=((1+e^((4iπ)/3) )/(2i(√3))) ⇒ ∫_(−∞) ^(+∞) Λ(z)dz=((2iπ)/(2i(√3))){1+e^((2iπ)/3) +1−e^((iπ)/3) } =(π/( (√3))){2 −(1/2)+((i(√3))/2) −(1/2)−i((√3)/2)} =(π/( (√3))) ⇒Ψ=(π/( (√3))) .
$$\Psi=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{x}^{\mathrm{2}} }{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{x}+\mathrm{1}\right)}\mathrm{dx}\:\mathrm{let}\:\Lambda\left(\mathrm{z}\right)=\frac{\mathrm{z}^{\mathrm{2}} }{\left(\mathrm{z}^{\mathrm{2}} −\mathrm{z}+\mathrm{1}\right)\left(\mathrm{z}^{\mathrm{2}} \:+\mathrm{z}+\mathrm{1}\right)} \\ $$$$\mathrm{poles}\:\mathrm{of}\:\Lambda? \\ $$$$\mathrm{z}^{\mathrm{2}} −\mathrm{z}+\mathrm{1}=\mathrm{0}\rightarrow\Delta=−\mathrm{3}\Rightarrow\mathrm{z}_{\mathrm{1}} =\frac{\mathrm{1}+\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\:=\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \:\mathrm{and}\:\mathrm{z}_{\mathrm{2}} =\frac{\mathrm{1}−\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}=\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \\ $$$$\mathrm{z}^{\mathrm{2}} +\mathrm{z}+\mathrm{1}=\mathrm{0}\rightarrow\Delta=−\mathrm{3}\:\Rightarrow\mathrm{x}_{\mathrm{1}} =\frac{−\mathrm{1}+\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}=\mathrm{e}^{\frac{\mathrm{2i}\pi}{\mathrm{3}}} \:\Rightarrow\mathrm{x}_{\mathrm{2}} =\mathrm{e}^{−\frac{\mathrm{2i}\pi}{\mathrm{3}}} \:\Rightarrow \\ $$$$\Lambda\left(\mathrm{z}\right)=\frac{\mathrm{z}^{\mathrm{2}} }{\left(\mathrm{z}−\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)\left(\mathrm{z}−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)\left(\mathrm{z}−\mathrm{e}^{\frac{\mathrm{2i}\pi}{\mathrm{3}}} \right)\left(\mathrm{z}−\mathrm{e}^{−\frac{\mathrm{2i}\pi}{\mathrm{3}}} \right)} \\ $$$$\int_{−\infty} ^{+\infty} \:\Lambda\left(\mathrm{z}\right)\mathrm{dz}=\mathrm{2i}\pi\left\{\mathrm{Res}\left(\Lambda,\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)+\mathrm{Res}\left(\Lambda,\mathrm{e}^{\frac{\mathrm{2i}\pi}{\mathrm{3}}} \right)\right\} \\ $$$$\mathrm{Res}\left(\Lambda,\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \right)\:=\frac{\mathrm{e}^{\frac{\mathrm{2i}\pi}{\mathrm{3}}} }{\mathrm{2isin}\left(\frac{\pi}{\mathrm{3}}\right)×}\frac{\left(\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} −\mathrm{1}\right)}{\mathrm{e}^{\mathrm{i}\pi} −\mathrm{1}} \\ $$$$=\frac{\mathrm{1}}{−\mathrm{4i}×\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}}\left(−\mathrm{1}−\mathrm{e}^{\frac{\mathrm{2i}\pi}{\mathrm{3}}} \right)\:=\frac{\mathrm{1}+\mathrm{e}^{\frac{\mathrm{2i}\pi}{\mathrm{3}}} }{\mathrm{2i}\sqrt{\mathrm{3}}} \\ $$$$\mathrm{Res}\left(\Lambda,\mathrm{e}^{\frac{\mathrm{2i}\pi}{\mathrm{3}}} \right)\:=\frac{\mathrm{e}^{\frac{\mathrm{4i}\pi}{\mathrm{3}}} }{\mathrm{2isin}\left(\frac{\mathrm{2}\pi}{\mathrm{3}}\right)}×\frac{\mathrm{e}^{\frac{\mathrm{2i}\pi}{\mathrm{3}}} \:+\mathrm{1}}{\mathrm{2}} \\ $$$$=\frac{\mathrm{1}+\mathrm{e}^{\frac{\mathrm{4i}\pi}{\mathrm{3}}} }{\mathrm{4i}×\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}}=\frac{\mathrm{1}+\mathrm{e}^{\frac{\mathrm{4i}\pi}{\mathrm{3}}} }{\mathrm{2i}\sqrt{\mathrm{3}}}\:\Rightarrow \\ $$$$\int_{−\infty} ^{+\infty} \:\Lambda\left(\mathrm{z}\right)\mathrm{dz}=\frac{\mathrm{2i}\pi}{\mathrm{2i}\sqrt{\mathrm{3}}}\left\{\mathrm{1}+\mathrm{e}^{\frac{\mathrm{2i}\pi}{\mathrm{3}}} \:+\mathrm{1}−\mathrm{e}^{\frac{\mathrm{i}\pi}{\mathrm{3}}} \right\} \\ $$$$=\frac{\pi}{\:\sqrt{\mathrm{3}}}\left\{\mathrm{2}\:−\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\:−\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right\}\:=\frac{\pi}{\:\sqrt{\mathrm{3}}}\:\Rightarrow\Psi=\frac{\pi}{\:\sqrt{\mathrm{3}}}\:. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *