calculus-please-solve-with-explanation-x-2-y-3-x-y-2-5-

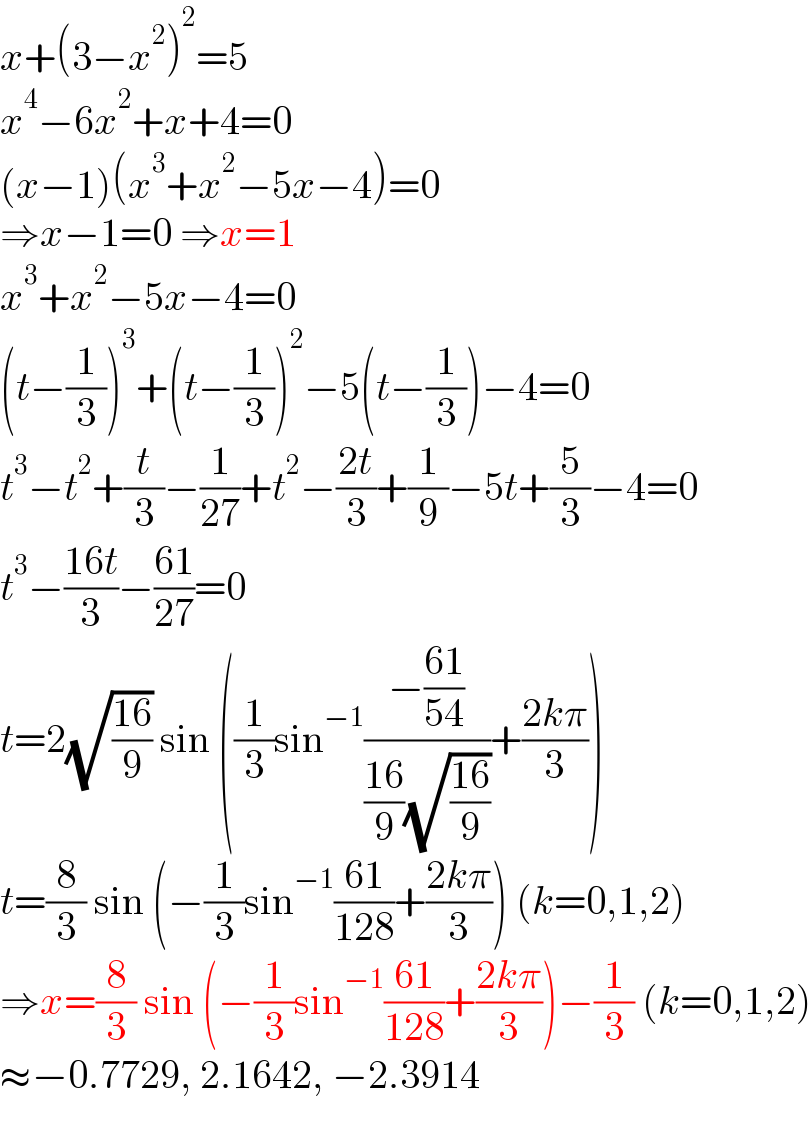
Question Number 126887 by mnjuly1970 last updated on 25/Dec/20

\dots c a l c u l u s \dots

p l e a s e s o l v e : (w i t h e x p l a n a t i o n)

{_{x^{2} + y = 3}^{x + y^{2} = 5}

Commented by Lordose last updated on 25/Dec/20

I {don}^{'} t know a calculus approach though

Commented by bramlexs22 last updated on 25/Dec/20

x = 5 - y^{2}

{(5 - y^{2})}^{2} + y = 3

y^{4} - 10 y^{2} + y + 22 = 0

(y - 2) (y^{3} + 2 y^{2} - 6 y - 11) = 0

y = 2 \land x = 1

y^{3} + 2 y^{2} - 6 y - 11 = 0

C a r d a n o

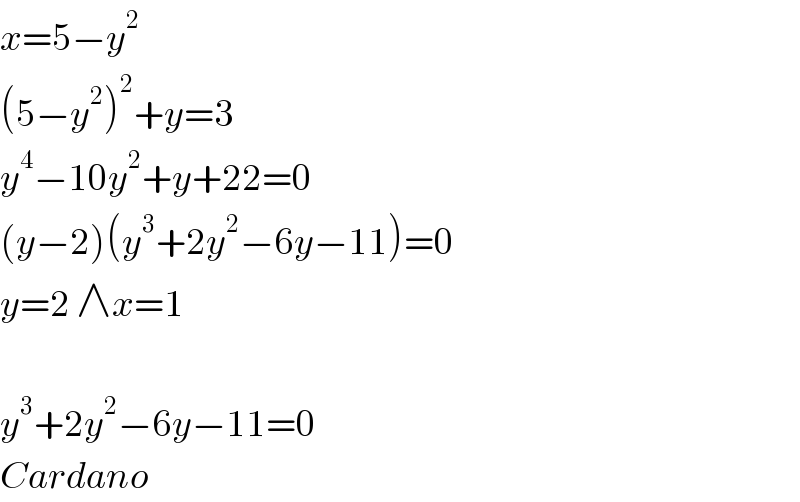
Answered by Lordose last updated on 25/Dec/20

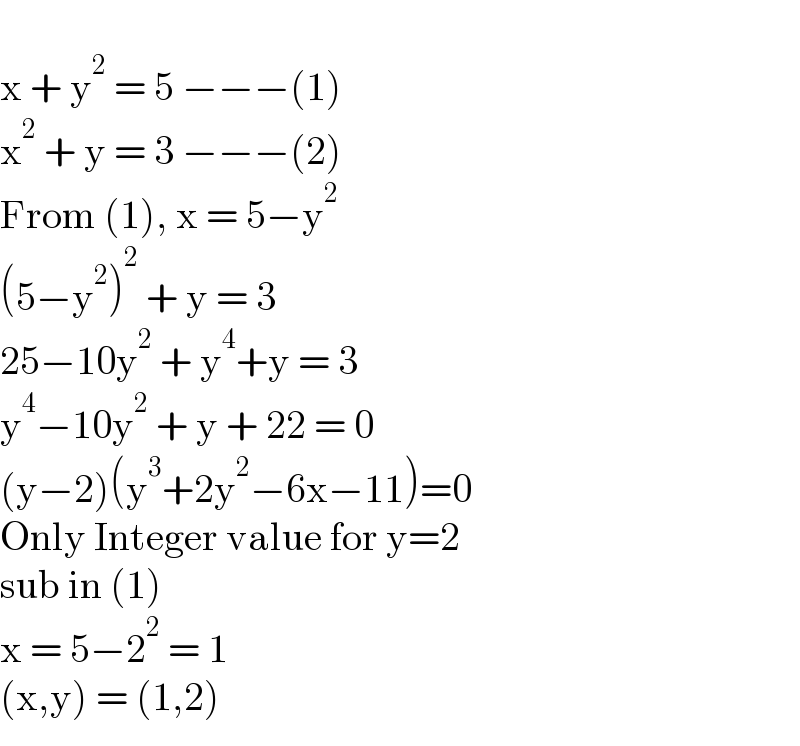
x + y^{2} = 5 - - - (1)

x^{2} + y = 3 - - - (2)

From (1), x = 5 - y^{2}

{(5 - y^{2})}^{2} + y = 3

25 - {10 y}^{2} + y^{4} + y = 3

y^{4} - {10 y}^{2} + y + 22 = 0

(y - 2) (y^{3} + {2 y}^{2} - 6 x - 11) = 0

Only Integer value for y = 2

sub in (1)

x = 5 - 2^{2} = 1

(x, y) = (1, 2)

Answered by mr W last updated on 25/Dec/20

x + {(3 - x^{2})}^{2} = 5

x^{4} - 6 x^{2} + x + 4 = 0

(x - 1) (x^{3} + x^{2} - 5 x - 4) = 0

\Rightarrow x - 1 = 0 \Rightarrow x = 1

x^{3} + x^{2} - 5 x - 4 = 0

{(t - \frac{1}{3})}^{3} + {(t - \frac{1}{3})}^{2} - 5 (t - \frac{1}{3}) - 4 = 0

t^{3} - t^{2} + \frac{t}{3} - \frac{1}{27} + t^{2} - \frac{2 t}{3} + \frac{1}{9} - 5 t + \frac{5}{3} - 4 = 0

t^{3} - \frac{16 t}{3} - \frac{61}{27} = 0

t = 2 \sqrt{\frac{16}{9}} \sin (\frac{1}{3} \sin^{- 1} \frac{- \frac{61}{54}}{\frac{16}{9} \sqrt{\frac{16}{9}}} + \frac{2 k π}{3})

t = \frac{8}{3} \sin (- \frac{1}{3} \sin^{- 1} \frac{61}{128} + \frac{2 k π}{3}) (k = 0, 1, 2)

\Rightarrow x = \frac{8}{3} \sin (- \frac{1}{3} \sin^{- 1} \frac{61}{128} + \frac{2 k π}{3}) - \frac{1}{3} (k = 0, 1, 2)

\approx - 0.7729, 2.1642, - 2.3914

Facebook Tweet Pin