Question Number 159973 by abdullah_ff last updated on 23/Nov/21

Commented by tounghoungko last updated on 23/Nov/21

Commented by abdullah_ff last updated on 23/Nov/21
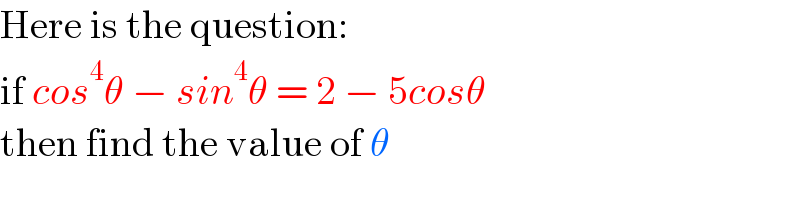
Commented by Rasheed.Sindhi last updated on 23/Nov/21
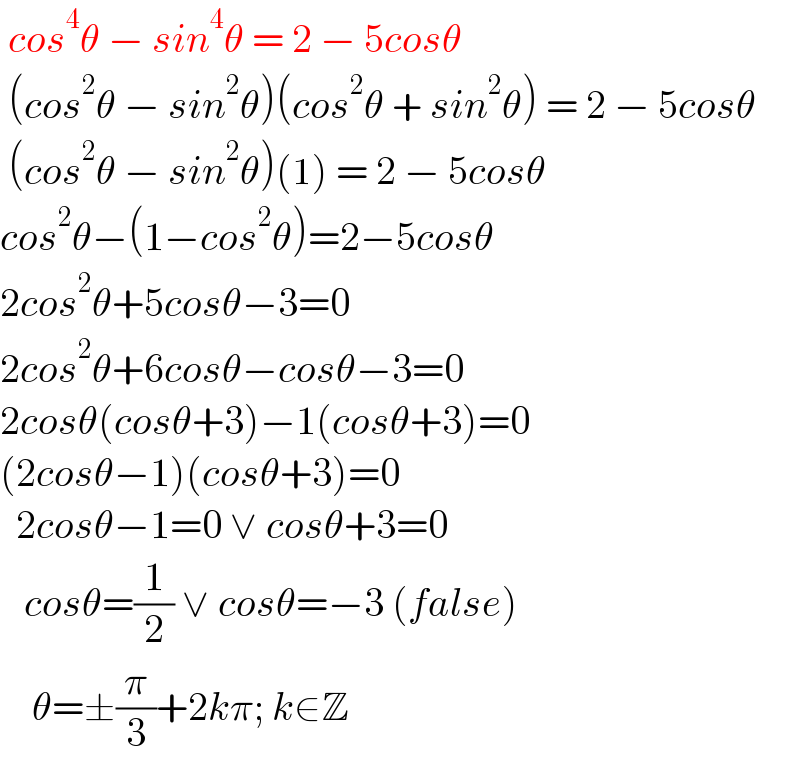
Commented by abdullah_ff last updated on 23/Nov/21

Commented by Rasheed.Sindhi last updated on 23/Nov/21
Commented by abdullah_ff last updated on 23/Nov/21

