Question Number 171260 by udaythool last updated on 11/Jun/22
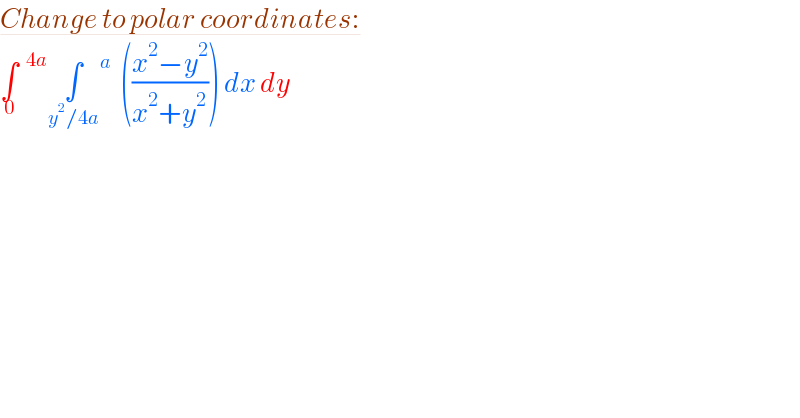
$$\underline{{Change}\:{to}\:{polar}\:{coordinates}:} \\ $$$$\underset{\mathrm{0}} {\int}^{\:\:\mathrm{4}{a}} \underset{{y}^{\mathrm{2}} /\mathrm{4}{a}} {\int}\overset{{a}} {\:}\:\:\left(\frac{{x}^{\mathrm{2}} −{y}^{\mathrm{2}} }{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} }\right)\:{dx}\:{dy} \\ $$
Answered by udaythool last updated on 13/Jun/22

$$\mathrm{Solution}:\:\mathrm{Let}\:{f}\equiv\:\left(\frac{{x}^{\mathrm{2}} −{y}^{\mathrm{2}} }{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} }\right)\Rightarrow{f}\:=\:{r}\mathrm{cos}\:\mathrm{2}\theta \\ $$$$\Rightarrow{I}=\int_{\mathrm{0}} ^{\:\mathrm{4}{a}} \int_{{y}^{\mathrm{2}} /\mathrm{4}{a}} ^{\:\:\:\:{a}} {f}\:{dxdy}\: \\ $$$$=\int_{\mathrm{0}} ^{\:\mathrm{2}{a}} \int_{{y}^{\mathrm{2}} /\mathrm{4}{a}} ^{\:\:\:\:{a}} {f}\:{dxdy}\:−\int_{\mathrm{2}{a}} ^{\:\mathrm{4}{a}} \int_{{a}} ^{\:\:{y}^{\mathrm{2}} /\mathrm{4}{a}} {f}\:{dxdy} \\ $$$$=\underset{{R}_{\mathrm{1}} } {\int\int}\:\:−\:\underset{{R}_{\mathrm{2}} } {\int\int}\:={I}_{\mathrm{1}} −{I}_{\mathrm{2}} \\ $$$$\mathrm{where}\:{R}_{\mathrm{1}} \equiv\mathrm{0}\leq{y}\leq\mathrm{2}{a},\:{y}^{\mathrm{2}} /\mathrm{4}{a}\leq{x}\leq{a} \\ $$$$\mathrm{and}\:{R}_{\mathrm{2}} \equiv\mathrm{2}{a}\leq{y}\leq\mathrm{4}{a},\:\:\:{a}\leq{x}\leq\:{y}^{\mathrm{2}} /\mathrm{4}{a} \\ $$$$\mathrm{For}\:\mathrm{polar}\:\mathrm{coordinates}\:\mathrm{we}\:\mathrm{should}\:\mathrm{have} \\ $$$$\mathrm{to}\:\mathrm{divide}\:\mathrm{both}\:{R}_{\mathrm{1}} \:\mathrm{into}\:{A},\:{B}\:\mathrm{and}\:{R}_{\mathrm{2}} \:\mathrm{into}\:{C},\:{D} \\ $$$$\mathrm{subregions}\:\mathrm{by}\:\mathrm{a}\:\mathrm{line}\:\mathrm{from}\:\mathrm{origin}\:\mathrm{and}\:\left(\mathrm{a},\:\mathrm{2}{a}\right).\: \\ $$$$\mathrm{Thus}\:{I}_{\mathrm{1}} ={I}_{{A}} +{I}_{{B}} \:\mathrm{and}\:{I}_{\mathrm{2}} ={I}_{{C}} +{I}_{{D}} \\ $$$$\mathrm{For}\:{A},\:\mathrm{0}\leq\theta\leq\mathrm{tan}^{−\mathrm{1}} \mathrm{2}\:\mathrm{and}\:\mathrm{0}\leq{r}\leq{a}/\mathrm{cos}\:\theta \\ $$$$\Rightarrow{I}_{{A}} =\int_{\mathrm{0}} ^{\mathrm{tan}^{−\mathrm{1}} \mathrm{2}} \int_{\mathrm{0}} ^{{a}/\mathrm{cos}\:\theta} {r}\:\mathrm{cos2}\theta\:{dr}\:{d}\theta\:={a}^{\mathrm{2}} \left(\mathrm{tan}^{−\mathrm{1}} \mathrm{2}−\mathrm{1}\right). \\ $$$$\mathrm{For}\:{B},\:\mathrm{tan}^{−\mathrm{1}} \mathrm{2}\leq\theta\leq\pi/\mathrm{2}\:\mathrm{and}\:\mathrm{0}\leq{r}\leq\mathrm{4}{a}\mathrm{cos}\:\theta/\mathrm{sin}^{\mathrm{2}} \theta \\ $$$$\Rightarrow{I}_{{B}} =\int_{\mathrm{tan}^{−\mathrm{1}} \mathrm{2}} ^{\pi/\mathrm{2}} \int_{\mathrm{0}} ^{\mathrm{4}{a}\mathrm{cos}\:\theta/\mathrm{sin}^{\mathrm{2}} \theta} {r}\:\mathrm{cos2}\theta\:{dr}\:{d}\theta \\ $$$${I}_{{B}} =\mathrm{8}{a}^{\mathrm{2}} \left(\pi−\mathrm{23}/\mathrm{24}−\mathrm{2tan}^{−\mathrm{1}} \mathrm{2}\right). \\ $$$$\mathrm{For}\:{C},\:\pi/\mathrm{4}\leq\theta\leq\mathrm{tan}^{−\mathrm{1}} \mathrm{2}\:\mathrm{and}\:\mathrm{4}{a}\mathrm{cos}\:\theta/\mathrm{sin}^{\mathrm{2}} \theta\leq{r}\leq\mathrm{4}{a}/\mathrm{sin}\:\theta \\ $$$$\Rightarrow{I}_{{C}} =\int_{\pi/\mathrm{4}} ^{\mathrm{tan}^{−\mathrm{1}} \mathrm{2}} \int_{\mathrm{4}{a}\mathrm{cos}\:\theta/\mathrm{sin}^{\mathrm{2}} \theta} ^{\mathrm{4}{a}/\mathrm{sin}\:\theta} {r}\:\mathrm{cos2}\theta\:{dr}\:{d}\theta \\ $$$${I}_{{C}} =\mathrm{8}{a}^{\mathrm{2}} \left(\pi+\mathrm{29}/\mathrm{24}−\mathrm{4tan}^{−\mathrm{1}} \mathrm{2}\right). \\ $$$$\mathrm{And}\:\mathrm{for}\:{D},\:\mathrm{tan}^{−\mathrm{1}} \mathrm{2}\leq\theta\leq\mathrm{tan}^{−\mathrm{1}} \mathrm{4}\:\mathrm{and}\:{a}/\mathrm{cos}\:\theta\leq{r}\leq\mathrm{4}{a}/\mathrm{sin}\:\theta \\ $$$$\Rightarrow{I}_{{D}} =\int_{\mathrm{tan}^{−\mathrm{1}} \mathrm{2}} ^{\mathrm{tan}^{−\mathrm{1}} \mathrm{4}} \int_{{a}/\mathrm{cos}\:\theta} ^{\mathrm{4}{a}/\mathrm{sin}\:\theta} {r}\:\mathrm{cos2}\theta\:{dr}\:{d}\theta \\ $$$${I}_{{D}} ={a}^{\mathrm{2}} \left(\mathrm{3}+\mathrm{174tan}^{−\mathrm{1}} \mathrm{2}−\mathrm{17tan}^{−\mathrm{1}} \mathrm{4}\right). \\ $$$$\therefore\:{I}=\left(\frac{−\mathrm{64}}{\mathrm{3}}\right){a}^{\mathrm{2}} +\mathrm{17}{a}^{\mathrm{2}} \mathrm{tan}^{−\mathrm{1}} \mathrm{4} \\ $$
