Compare-x-2-3-2-3-and-y-3-2-3-2-

Question Number 150516 by mathdanisur last updated on 13/Aug/21

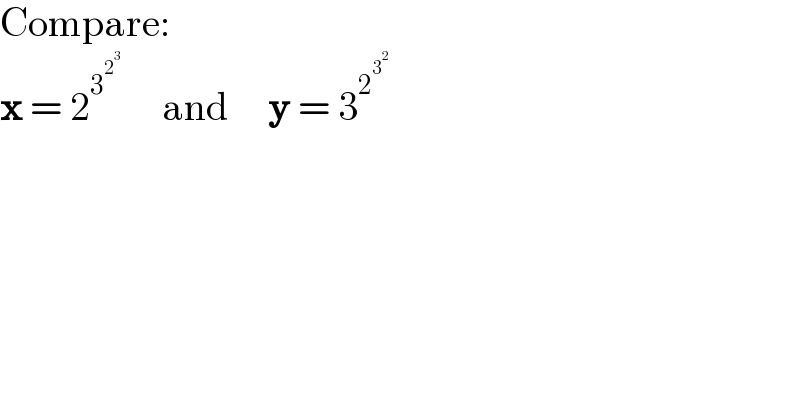
$$\mathrm{Compare}: \\ $$$$\boldsymbol{\mathrm{x}}\:=\:\mathrm{2}^{\mathrm{3}^{\mathrm{2}^{\mathrm{3}} } } \:\:\:\:\:\mathrm{and}\:\:\:\:\:\boldsymbol{\mathrm{y}}\:=\:\mathrm{3}^{\mathrm{2}^{\mathrm{3}^{\mathrm{2}} } } \\ $$

Commented by mr W last updated on 13/Aug/21

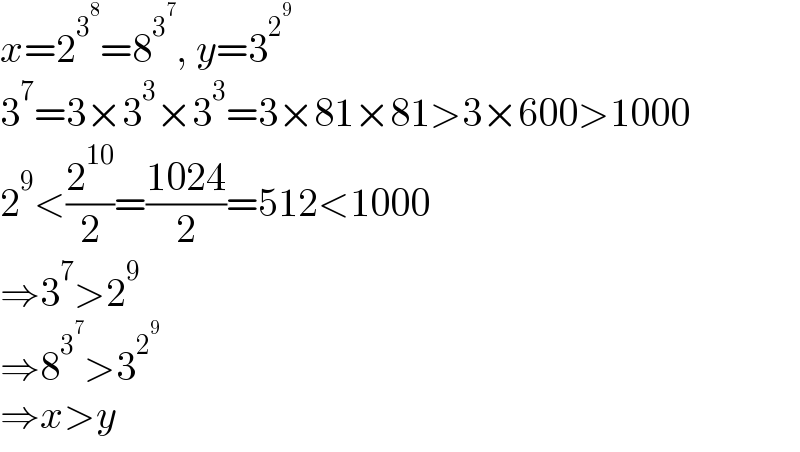
x=2^3^8 =8^3^7 , y=3^2^9 3^7 =3×3^3 ×3^3 =3×81×81>3×600>1000 2^9 <(2^(10) /2)=((1024)/2)=512<1000 ⇒3^7 >2^9 ⇒8^3^7 >3^2^9 ⇒x>y

$${x}=\mathrm{2}^{\mathrm{3}^{\mathrm{8}} } =\mathrm{8}^{\mathrm{3}^{\mathrm{7}} } ,\:{y}=\mathrm{3}^{\mathrm{2}^{\mathrm{9}} } \\ $$$$\mathrm{3}^{\mathrm{7}} =\mathrm{3}×\mathrm{3}^{\mathrm{3}} ×\mathrm{3}^{\mathrm{3}} =\mathrm{3}×\mathrm{81}×\mathrm{81}>\mathrm{3}×\mathrm{600}>\mathrm{1000} \\ $$$$\mathrm{2}^{\mathrm{9}} <\frac{\mathrm{2}^{\mathrm{10}} }{\mathrm{2}}=\frac{\mathrm{1024}}{\mathrm{2}}=\mathrm{512}<\mathrm{1000} \\ $$$$\Rightarrow\mathrm{3}^{\mathrm{7}} >\mathrm{2}^{\mathrm{9}} \\ $$$$\Rightarrow\mathrm{8}^{\mathrm{3}^{\mathrm{7}} } >\mathrm{3}^{\mathrm{2}^{\mathrm{9}} } \\ $$$$\Rightarrow{x}>{y} \\ $$

Commented by mathdanisur last updated on 13/Aug/21