Question Number 100769 by Rio Michael last updated on 28/Jun/20
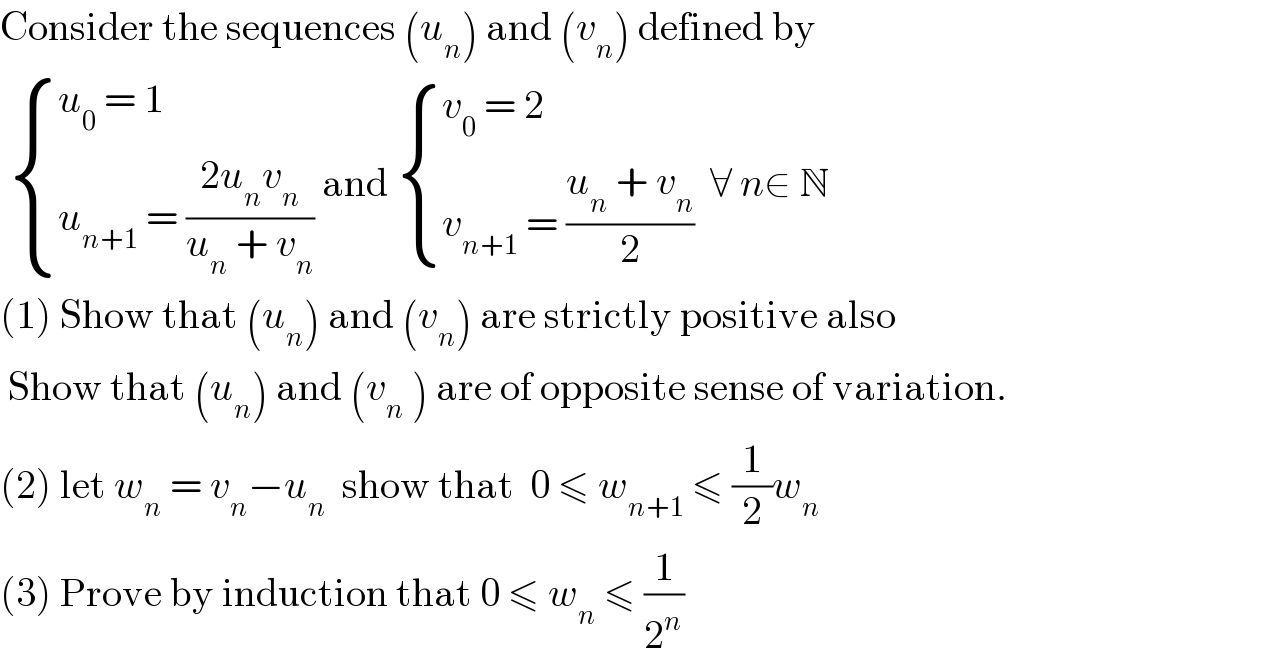
$$\mathrm{Consider}\:\mathrm{the}\:\mathrm{sequences}\:\left({u}_{{n}} \right)\:\mathrm{and}\:\left({v}_{{n}} \right)\:\mathrm{defined}\:\mathrm{by} \\ $$$$\:\begin{cases}{{u}_{\mathrm{0}} \:=\:\mathrm{1}}\\{{u}_{{n}+\mathrm{1}} \:=\:\frac{\mathrm{2}{u}_{{n}} {v}_{{n}} }{{u}_{{n}} \:+\:{v}_{{n}} }}\end{cases}\:\mathrm{and}\:\begin{cases}{{v}_{\mathrm{0}} \:=\:\mathrm{2}}\\{{v}_{{n}+\mathrm{1}} \:=\:\frac{{u}_{{n}} \:+\:{v}_{{n}} }{\mathrm{2}}}\end{cases}\:\:\forall\:{n}\in\:\mathbb{N} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{Show}\:\mathrm{that}\:\left({u}_{{n}} \right)\:\mathrm{and}\:\left({v}_{{n}} \right)\:\mathrm{are}\:\mathrm{strictly}\:\mathrm{positive}\:\mathrm{also} \\ $$$$\:\mathrm{Show}\:\mathrm{that}\:\left({u}_{{n}} \right)\:\mathrm{and}\:\left({v}_{{n}} \:\right)\:\mathrm{are}\:\mathrm{of}\:\mathrm{opposite}\:\mathrm{sense}\:\mathrm{of}\:\mathrm{variation}. \\ $$$$\left(\mathrm{2}\right)\:\mathrm{let}\:{w}_{{n}} \:=\:{v}_{{n}} −{u}_{{n}} \:\:\mathrm{show}\:\mathrm{that}\:\:\mathrm{0}\:\leqslant\:{w}_{{n}+\mathrm{1}} \:\leqslant\:\frac{\mathrm{1}}{\mathrm{2}}{w}_{{n}} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{Prove}\:\mathrm{by}\:\mathrm{induction}\:\mathrm{that}\:\mathrm{0}\:\leqslant\:{w}_{{n}} \:\leqslant\:\frac{\mathrm{1}}{\mathrm{2}^{{n}} } \\ $$
