Question Number 61842 by psyche last updated on 10/Jun/19
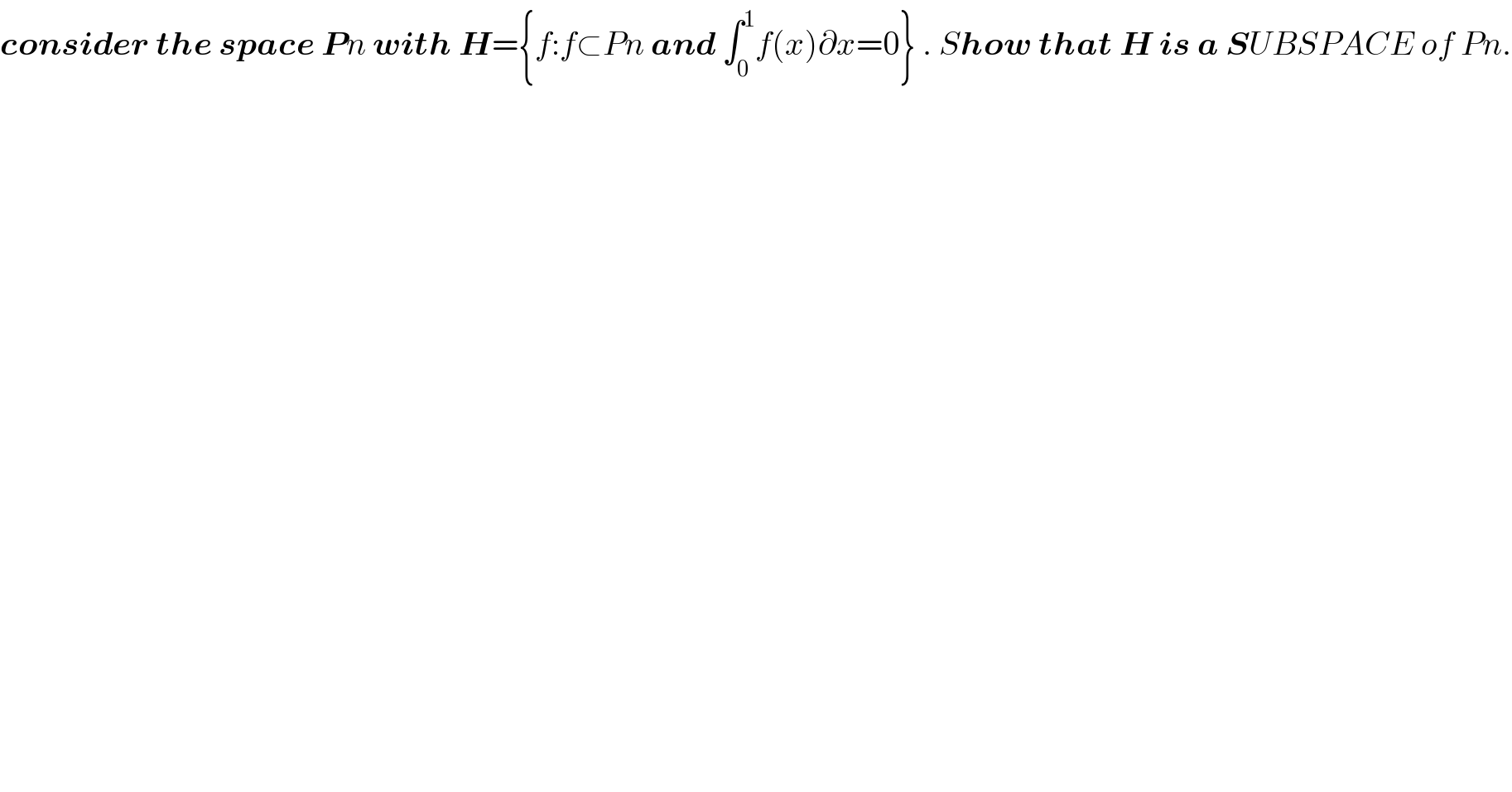
$$\boldsymbol{{consider}}\:\boldsymbol{{the}}\:\boldsymbol{{space}}\:\boldsymbol{{P}}{n}\:\boldsymbol{{with}}\:\boldsymbol{{H}}=\left\{{f}:{f}\subset{Pn}\:\boldsymbol{{and}}\:\int_{\mathrm{0}} ^{\mathrm{1}} {f}\left({x}\right)\partial{x}=\mathrm{0}\right\}\:.\:{S}\boldsymbol{{how}}\:\boldsymbol{{that}}\:\boldsymbol{{H}}\:\boldsymbol{{is}}\:\boldsymbol{{a}}\:\boldsymbol{{S}}{UBSPACE}\:{of}\:{Pn}. \\ $$
Commented by arcana last updated on 10/Jun/19

$$\mathrm{define}\:\mathrm{P}_{{n}} \\ $$
