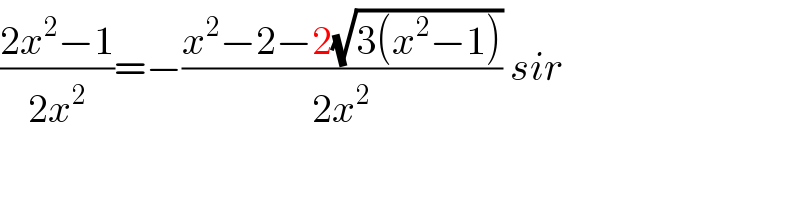Question Number 61511 by Kunal12588 last updated on 03/Jun/19
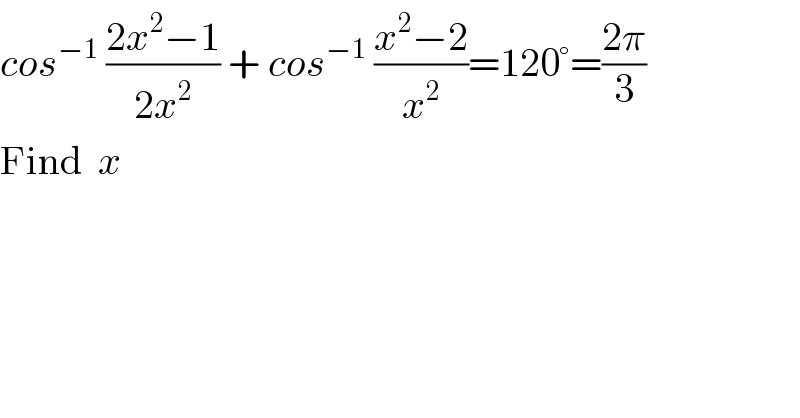
Answered by MJS last updated on 03/Jun/19
![cos^(−1) ((2x^2 −1)/(2x^2 )) +cos^(−1) ((x^2 −2)/x^2 ) =((2π)/3) π−sin^(−1) ((2x^2 −1)/x^2 ) −sin^(−1) ((x^2 −2)/x^2 )=((2π)/3) sin^(−1) ((2x^2 −1)/x^2 ) =(π/3)−sin^(−1) ((x^2 −2)/x^2 ) ((2x^2 −1)/(2x^2 ))=−((x^2 −2−(√(3(x^2 −1))))/(2x^2 )) [beware of false solutions!!!] ⇒ x=±1 [false!] ∨ x=±((√(21))/3)](https://www.tinkutara.com/question/Q61512.png)
Commented by Kunal12588 last updated on 04/Jun/19

Commented by Kunal12588 last updated on 04/Jun/19
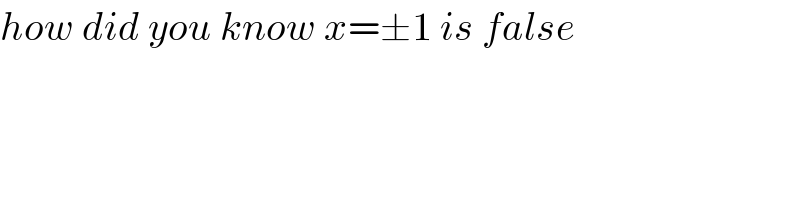
Commented by Kunal12588 last updated on 04/Jun/19