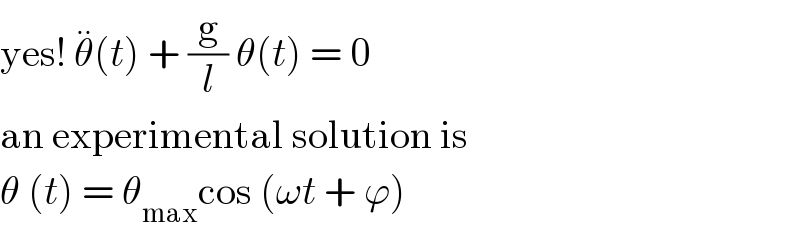d-2-dt-2-g-l-sin-0-Or-t-g-l-t-0-

Question Number 114340 by Dwaipayan Shikari last updated on 18/Sep/20

\frac{d^{2} θ}{{d t}^{2}} + \frac{g}{l} s i n θ = 0

O r \overset{\cdot \cdot}{θ} (t) + \frac{g}{l} θ (t) = 0

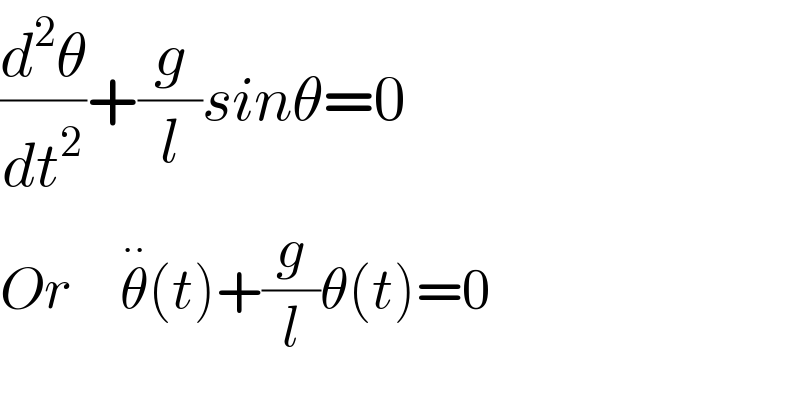
Commented by mr W last updated on 18/Sep/20

Commented by mohammad17 last updated on 18/Sep/20

w h a t s t h e m e a n (\frac{g}{l}) i s i t c o n s t a n t ?

Commented by Dwaipayan Shikari last updated on 18/Sep/20

Commented by Dwaipayan Shikari last updated on 18/Sep/20

G r a v i t a t i o n a l a c c e l a r a t i o n o n s i m p l e p e n d u l a m (- g)

O n h o r i z o n t a l d i r e c t i o n - g s i n θ

S = l θ

\frac{d^{2} s}{{d t}^{2}} = l \frac{d^{2} θ}{{d t}^{2}}

l \frac{d^{2} θ}{{d t}^{2}} = - g s i n θ

\frac{d^{2} θ}{{d t}^{2}} + \frac{g}{l} s i n θ = 0

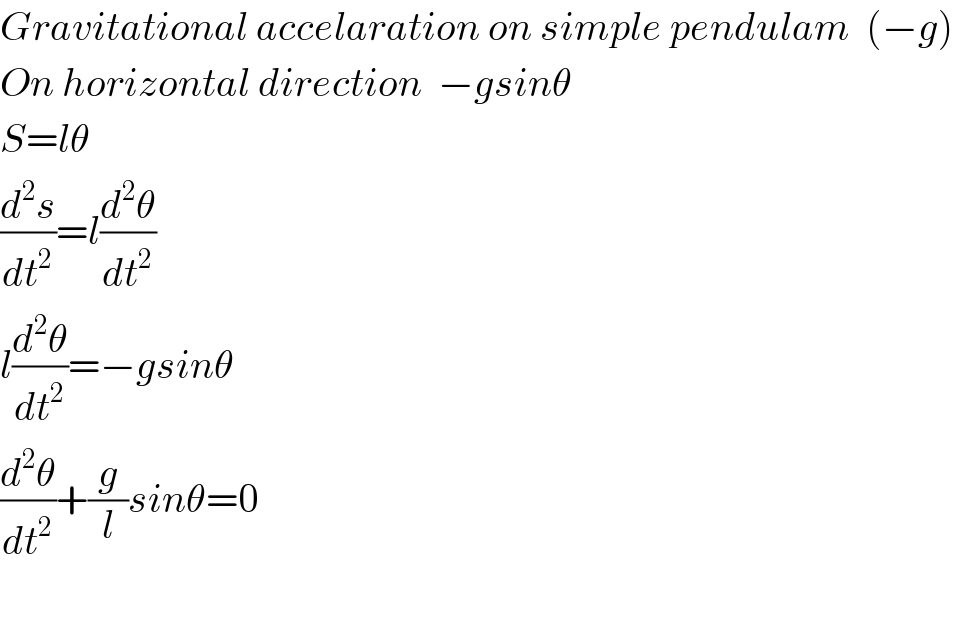
Answered by Rio Michael last updated on 18/Sep/20

yes! \overset{. .}{θ} (t) + \frac{g}{l} θ (t) = 0

an experimental solution is

θ (t) = θ_{\max} \cos (ω t + φ)