Menu Close

d-2-y-dx-2-2-dy-dx-2y-cos-4x-by-UC-method-

Tinku Tara 0 Comments
Pin




Question Number 103468 by bemath last updated on 15/Jul/20
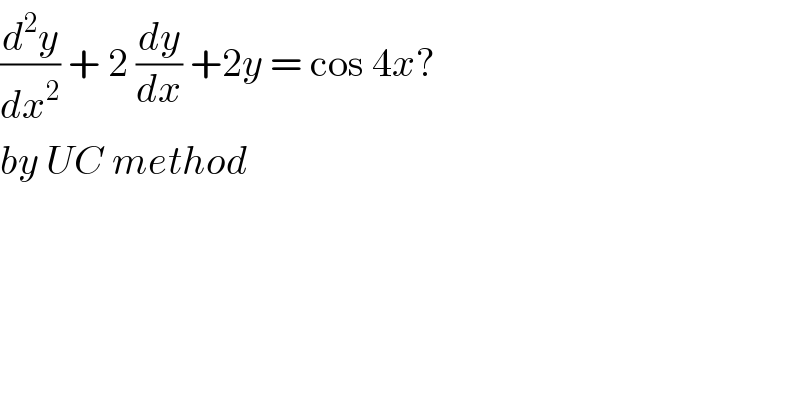
(d^2 y/dx^2 ) + 2 (dy/dx) +2y = cos 4x? by UC method
$$\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }\:+\:\mathrm{2}\:\frac{{dy}}{{dx}}\:+\mathrm{2}{y}\:=\:\mathrm{cos}\:\mathrm{4}{x}? \\ $$$${by}\:{UC}\:{method}\: \\ $$
Answered by bramlex last updated on 15/Jul/20
Homogenous solution ρ^2 +2ρ+2 = 0 (ρ+1)^2 +1 = 0 ρ = −1± i y_h = e^(−x) (C_1 cos x+C_2 sin x) particular solution y_p = Acos 4x+Bsin 4x y′_p =−4Asin 4x+4Bcos 4x y′′_p = −16Acos 4x−16Bsin 4x comparing coefficient −16Acos 4x−16Bsin 4x−8Asin 4x +8Bcos 4x+2Acos 4x+2Bsin 4x = cos 4x (−14A+8B)cos 4x +(−14B−8A)sin 4x = cos 4x ⇒ { ((−14B−8A=0 ⇒A=−(7/4))),((−14A+8B=1 ⇒B=1−14((7/4)))) :} B = 1−((49)/2)= −((47)/2) general solution y=e^(−x) (C_1 cos x+C_2 sin x)−(7/4)cos 4x−((47)/2) sin 4x .(⊕)
$$\mathrm{Homogenous}\:\mathrm{solution} \\ $$$$\rho^{\mathrm{2}} +\mathrm{2}\rho+\mathrm{2}\:=\:\mathrm{0} \\ $$$$\left(\rho+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{1}\:=\:\mathrm{0} \\ $$$$\rho\:=\:−\mathrm{1}\pm\:\mathrm{i}\: \\ $$$$\mathrm{y}_{\mathrm{h}} \:=\:\mathrm{e}^{−\mathrm{x}} \left(\mathrm{C}_{\mathrm{1}} \mathrm{cos}\:\mathrm{x}+\mathrm{C}_{\mathrm{2}} \mathrm{sin}\:\mathrm{x}\right) \\ $$$$\mathrm{particular}\:\mathrm{solution} \\ $$$$\mathrm{y}_{\mathrm{p}} =\:\mathrm{Acos}\:\mathrm{4x}+\mathrm{Bsin}\:\mathrm{4x} \\ $$$$\mathrm{y}'_{\mathrm{p}} =−\mathrm{4Asin}\:\mathrm{4x}+\mathrm{4Bcos}\:\mathrm{4x} \\ $$$$\mathrm{y}''_{\mathrm{p}} =\:−\mathrm{16Acos}\:\mathrm{4x}−\mathrm{16Bsin}\:\mathrm{4x} \\ $$$$\mathrm{comparing}\:\mathrm{coefficient} \\ $$$$−\mathrm{16Acos}\:\mathrm{4x}−\mathrm{16Bsin}\:\mathrm{4x}−\mathrm{8Asin} \\ $$$$\mathrm{4x}\:+\mathrm{8Bcos}\:\mathrm{4x}+\mathrm{2Acos}\:\mathrm{4x}+\mathrm{2Bsin} \\ $$$$\mathrm{4x}\:=\:\mathrm{cos}\:\mathrm{4x} \\ $$$$\left(−\mathrm{14A}+\mathrm{8B}\right)\mathrm{cos}\:\mathrm{4x} \\ $$$$+\left(−\mathrm{14B}−\mathrm{8A}\right)\mathrm{sin}\:\mathrm{4x}\:=\:\mathrm{cos}\:\mathrm{4x} \\ $$$$\Rightarrow\begin{cases}{−\mathrm{14B}−\mathrm{8A}=\mathrm{0}\:\Rightarrow\mathrm{A}=−\frac{\mathrm{7}}{\mathrm{4}}}\\{−\mathrm{14A}+\mathrm{8B}=\mathrm{1}\:\Rightarrow\mathrm{B}=\mathrm{1}−\mathrm{14}\left(\frac{\mathrm{7}}{\mathrm{4}}\right)}\end{cases} \\ $$$$\mathrm{B}\:=\:\mathrm{1}−\frac{\mathrm{49}}{\mathrm{2}}=\:−\frac{\mathrm{47}}{\mathrm{2}} \\ $$$$\mathrm{general}\:\mathrm{solution}\: \\ $$$$\mathrm{y}=\mathrm{e}^{−\mathrm{x}} \left(\mathrm{C}_{\mathrm{1}} \mathrm{cos}\:\mathrm{x}+\mathrm{C}_{\mathrm{2}} \mathrm{sin}\:\mathrm{x}\right)−\frac{\mathrm{7}}{\mathrm{4}}\mathrm{cos} \\ $$$$\mathrm{4x}−\frac{\mathrm{47}}{\mathrm{2}}\:\mathrm{sin}\:\mathrm{4x}\:.\left(\oplus\right) \\ $$
Answered by mathmax by abdo last updated on 16/Jul/20
let use laplace transform y^(′′) +2y^′ +2y =cos(4x) ⇒L(y^(′′) )+2L(y^′ )+2L(y) =L(cos(4x)) ⇒ x^2 L(y)−xy(o)−y^′ (0)+2(xL(y)−y(o))+2L(y) =L(cos(4x)) ⇒ (x^2 +2x+2)L(y)−(x+2)y(o)−y^′ (0) =L(cos(4x)) L(cos(4x)) =∫_0 ^∞ cos(4t)e^(−xt) dt =Re(∫_0 ^∞ e^((−x+4i)t) dt) ∫_0 ^∞ e^((−x+4i)t) dt =[(1/(−x+4i))e^((−x+4i)t) ]_0 ^∞ =((−1)/(−x+4i)) =(1/(x−4i)) =((x+4i)/(x^2 +16)) ⇒ L(cos(4x)) =(x/(x^2 +16)) e ⇒(x^2 +2x+2)L(y) =(x+2)y(o)+y^′ (0)+ ⇒L(y) =((x+2)/(x^2 +2x+2))y(o)+((y^′ (0))/(x^2 +x+2)) +(x/((x^2 +16)(x^2 +2x+2))) ⇒ y(x) =y(o)L^(−1) (((x+2)/(x^2 +2x+2))) +y^′ (0)L^(−1) ((1/(x^2 +x+2))) +L^(−1) ((x/((x^2 +16)(x^2 +2x+2)))) let decompose f(x) =((x+2)/(x^2 +2x+2)) Δ^′ =−1 ⇒x_1 =−1+i and x_2 =−1−i ⇒f(x) =((x+2)/((x−x_1 )(x−x_2 ))) =(1/(2i))(x+2){(1/(x−x_1 ))−(1/(x−x_2 ))} =(1/(2i)){((x+2)/(x−x_1 ))−((x+2)/(x−x_2 ))} =(1/(2i)){((x−x_1 +2+x_1 )/(x−x_1 ))−((x−x_2 +2+x_2 )/(x−x_2 ))} =(1/(2i)){((2+x_1 )/(x−x_1 ))−((2+x_2 )/(x−x_2 ))} ⇒L^(−1) (f) =((2+x_1 )/(2i))e^(x_1 x) −((2+x_2 )/(2i))e^(x_2 x) L^(−1) ((1/(x^2 +x+2))) =L^(−1) ((1/(2i))((1/(x−x_1 ))−(1/(x−x_2 )))) =(1/(2i)) e^(x_1 x) −(1/(2i))e^(x_2 x) =(1/(2i)){ e^(−x) e^(ix) −e^(−x) e^(−ix) } =e^(−x) ×((e^(ix) −e^(−ix) )/(2i)) =e^(−x) sinx....be continued...
$$\mathrm{let}\:\mathrm{use}\:\mathrm{laplace}\:\mathrm{transform} \\ $$$$\mathrm{y}^{''} \:+\mathrm{2y}^{'} \:+\mathrm{2y}\:=\mathrm{cos}\left(\mathrm{4x}\right)\:\Rightarrow\mathrm{L}\left(\mathrm{y}^{''} \right)+\mathrm{2L}\left(\mathrm{y}^{'} \right)+\mathrm{2L}\left(\mathrm{y}\right)\:=\mathrm{L}\left(\mathrm{cos}\left(\mathrm{4x}\right)\right)\:\Rightarrow \\ $$$$\mathrm{x}^{\mathrm{2}} \:\mathrm{L}\left(\mathrm{y}\right)−\mathrm{xy}\left(\mathrm{o}\right)−\mathrm{y}^{'} \left(\mathrm{0}\right)+\mathrm{2}\left(\mathrm{xL}\left(\mathrm{y}\right)−\mathrm{y}\left(\mathrm{o}\right)\right)+\mathrm{2L}\left(\mathrm{y}\right)\:=\mathrm{L}\left(\mathrm{cos}\left(\mathrm{4x}\right)\right)\:\Rightarrow \\ $$$$\left(\mathrm{x}^{\mathrm{2}} +\mathrm{2x}+\mathrm{2}\right)\mathrm{L}\left(\mathrm{y}\right)−\left(\mathrm{x}+\mathrm{2}\right)\mathrm{y}\left(\mathrm{o}\right)−\mathrm{y}^{'} \left(\mathrm{0}\right)\:=\mathrm{L}\left(\mathrm{cos}\left(\mathrm{4x}\right)\right) \\ $$$$\mathrm{L}\left(\mathrm{cos}\left(\mathrm{4x}\right)\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\mathrm{cos}\left(\mathrm{4t}\right)\mathrm{e}^{−\mathrm{xt}} \:\mathrm{dt}\:=\mathrm{Re}\left(\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{\left(−\mathrm{x}+\mathrm{4i}\right)\mathrm{t}} \mathrm{dt}\right) \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\:\mathrm{e}^{\left(−\mathrm{x}+\mathrm{4i}\right)\mathrm{t}} \mathrm{dt}\:=\left[\frac{\mathrm{1}}{−\mathrm{x}+\mathrm{4i}}\mathrm{e}^{\left(−\mathrm{x}+\mathrm{4i}\right)\mathrm{t}} \right]_{\mathrm{0}} ^{\infty} \:=\frac{−\mathrm{1}}{−\mathrm{x}+\mathrm{4i}}\:=\frac{\mathrm{1}}{\mathrm{x}−\mathrm{4i}}\:=\frac{\mathrm{x}+\mathrm{4i}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{16}}\:\Rightarrow \\ $$$$\mathrm{L}\left(\mathrm{cos}\left(\mathrm{4x}\right)\right)\:=\frac{\mathrm{x}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{16}} \\ $$$$\mathrm{e}\:\Rightarrow\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{2x}+\mathrm{2}\right)\mathrm{L}\left(\mathrm{y}\right)\:=\left(\mathrm{x}+\mathrm{2}\right)\mathrm{y}\left(\mathrm{o}\right)+\mathrm{y}^{'} \left(\mathrm{0}\right)+ \\ $$$$\Rightarrow\mathrm{L}\left(\mathrm{y}\right)\:=\frac{\mathrm{x}+\mathrm{2}}{\mathrm{x}^{\mathrm{2}} +\mathrm{2x}+\mathrm{2}}\mathrm{y}\left(\mathrm{o}\right)+\frac{\mathrm{y}^{'} \left(\mathrm{0}\right)}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{x}+\mathrm{2}}\:+\frac{\mathrm{x}}{\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{16}\right)\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{2x}+\mathrm{2}\right)}\:\Rightarrow \\ $$$$\mathrm{y}\left(\mathrm{x}\right)\:=\mathrm{y}\left(\mathrm{o}\right)\mathrm{L}^{−\mathrm{1}} \left(\frac{\mathrm{x}+\mathrm{2}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{2x}+\mathrm{2}}\right)\:+\mathrm{y}^{'} \left(\mathrm{0}\right)\mathrm{L}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{x}+\mathrm{2}}\right)\:+\mathrm{L}^{−\mathrm{1}} \left(\frac{\mathrm{x}}{\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{16}\right)\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{2x}+\mathrm{2}\right)}\right) \\ $$$$\mathrm{let}\:\mathrm{decompose}\:\mathrm{f}\left(\mathrm{x}\right)\:=\frac{\mathrm{x}+\mathrm{2}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{2x}+\mathrm{2}} \\ $$$$\Delta^{'} \:=−\mathrm{1}\:\Rightarrow\mathrm{x}_{\mathrm{1}} =−\mathrm{1}+\mathrm{i}\:\mathrm{and}\:\mathrm{x}_{\mathrm{2}} =−\mathrm{1}−\mathrm{i}\:\Rightarrow\mathrm{f}\left(\mathrm{x}\right)\:=\frac{\mathrm{x}+\mathrm{2}}{\left(\mathrm{x}−\mathrm{x}_{\mathrm{1}} \right)\left(\mathrm{x}−\mathrm{x}_{\mathrm{2}} \right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2i}}\left(\mathrm{x}+\mathrm{2}\right)\left\{\frac{\mathrm{1}}{\mathrm{x}−\mathrm{x}_{\mathrm{1}} }−\frac{\mathrm{1}}{\mathrm{x}−\mathrm{x}_{\mathrm{2}} }\right\}\:=\frac{\mathrm{1}}{\mathrm{2i}}\left\{\frac{\mathrm{x}+\mathrm{2}}{\mathrm{x}−\mathrm{x}_{\mathrm{1}} }−\frac{\mathrm{x}+\mathrm{2}}{\mathrm{x}−\mathrm{x}_{\mathrm{2}} }\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2i}}\left\{\frac{\mathrm{x}−\mathrm{x}_{\mathrm{1}} \:+\mathrm{2}+\mathrm{x}_{\mathrm{1}} }{\mathrm{x}−\mathrm{x}_{\mathrm{1}} }−\frac{\mathrm{x}−\mathrm{x}_{\mathrm{2}} +\mathrm{2}+\mathrm{x}_{\mathrm{2}} }{\mathrm{x}−\mathrm{x}_{\mathrm{2}} }\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2i}}\left\{\frac{\mathrm{2}+\mathrm{x}_{\mathrm{1}} }{\mathrm{x}−\mathrm{x}_{\mathrm{1}} }−\frac{\mathrm{2}+\mathrm{x}_{\mathrm{2}} }{\mathrm{x}−\mathrm{x}_{\mathrm{2}} }\right\}\:\Rightarrow\mathrm{L}^{−\mathrm{1}} \left(\mathrm{f}\right)\:=\frac{\mathrm{2}+\mathrm{x}_{\mathrm{1}} }{\mathrm{2i}}\mathrm{e}^{\mathrm{x}_{\mathrm{1}} \mathrm{x}} −\frac{\mathrm{2}+\mathrm{x}_{\mathrm{2}} }{\mathrm{2i}}\mathrm{e}^{\mathrm{x}_{\mathrm{2}} \mathrm{x}} \\ $$$$\mathrm{L}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{x}+\mathrm{2}}\right)\:=\mathrm{L}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2i}}\left(\frac{\mathrm{1}}{\mathrm{x}−\mathrm{x}_{\mathrm{1}} }−\frac{\mathrm{1}}{\mathrm{x}−\mathrm{x}_{\mathrm{2}} }\right)\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2i}}\:\mathrm{e}^{\mathrm{x}_{\mathrm{1}} \mathrm{x}} −\frac{\mathrm{1}}{\mathrm{2i}}\mathrm{e}^{\mathrm{x}_{\mathrm{2}} \mathrm{x}} \:=\frac{\mathrm{1}}{\mathrm{2i}}\left\{\:\mathrm{e}^{−\mathrm{x}} \:\mathrm{e}^{\mathrm{ix}} −\mathrm{e}^{−\mathrm{x}} \mathrm{e}^{−\mathrm{ix}} \right\}\:=\mathrm{e}^{−\mathrm{x}} ×\frac{\mathrm{e}^{\mathrm{ix}} −\mathrm{e}^{−\mathrm{ix}} }{\mathrm{2i}} \\ $$$$=\mathrm{e}^{−\mathrm{x}} \:\mathrm{sinx}….\mathrm{be}\:\mathrm{continued}… \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *