Question Number 157141 by cortano last updated on 20/Oct/21
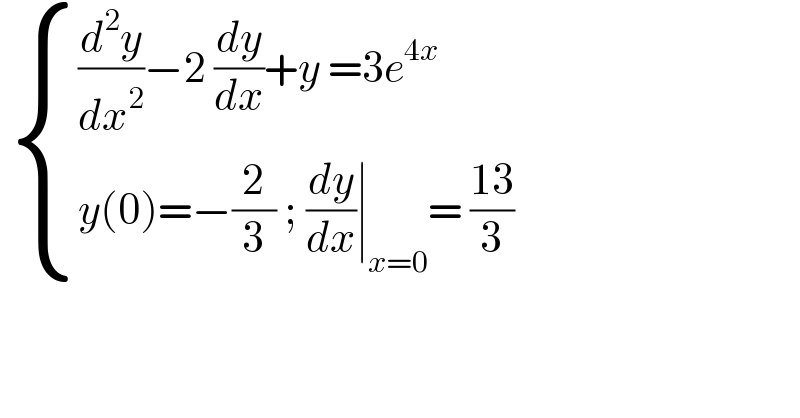
$$\:\begin{cases}{\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }−\mathrm{2}\:\frac{{dy}}{{dx}}+{y}\:=\mathrm{3}{e}^{\mathrm{4}{x}} }\\{{y}\left(\mathrm{0}\right)=−\frac{\mathrm{2}}{\mathrm{3}}\:;\:\frac{{dy}}{{dx}}\mid_{{x}=\mathrm{0}} =\:\frac{\mathrm{13}}{\mathrm{3}}}\end{cases}\: \\ $$
Commented by john_santu last updated on 21/Oct/21
![{ ((y′′−2y′+y=3e^(4x) )),((y(0)=−(2/3) ; y′(0)=((13)/3))) :} By wronskian method ⇒λ^2 −2λ+1=0⇒λ_(1,2) =1 ⇒y_h = Ae^x +Bxe^x Particular solution W(u_1 ,u_2 )= determinant (((e^x xe^x )),((e^x e^x +xe^x )))=e^(2x) W_1 = determinant (((0 xe^x )),((3e^(4x) e^x +xe^x )))=−3xe^(5x) W_2 = determinant (((e^x 0)),((e^x 3e^(4x) )))=3e^(5x) ⇒v_1 =∫ (w_1 /w) dx=∫ ((−3xe^(5x) )/e^(2x) ) dx =−3∫xe^(3x) dx =−3[ (1/3)xe^(3x) −(1/9)e^(3x) ]=−xe^(3x) +(1/3)e^(3x) ⇒v_2 =∫ (w_2 /w) dx=∫ ((3e^(5x) )/e^(2x) ) dx=∫3e^(3x) dx=e^(3x) ⇒y_p =u_1 v_1 +u_2 v_2 =e^x (−xe^(3x) +(1/3)e^(3x) )+xe^x (e^(3x) ) ⇒y_p =(1/3)e^(4x) y_g =Ae^x +Bxe^x +(1/3)e^(4x) { ((x=0⇒A+(1/3)=−(2/3) ; A=−1)),((y′=Ae^x +Be^x +Bxe^x +(4/3)e^(4x) )),((y′(0)=−1+B+(4/3)=((13)/3)⇒B=4 )) :} ⇔ ∴ y=−e^x +4xe^x +(1/3)e^(4x)](https://www.tinkutara.com/question/Q157239.png)
$$\:\begin{cases}{{y}''−\mathrm{2}{y}'+{y}=\mathrm{3}{e}^{\mathrm{4}{x}} }\\{{y}\left(\mathrm{0}\right)=−\frac{\mathrm{2}}{\mathrm{3}}\:;\:{y}'\left(\mathrm{0}\right)=\frac{\mathrm{13}}{\mathrm{3}}}\end{cases} \\ $$$$\:{By}\:{wronskian}\:{method} \\ $$$$\:\Rightarrow\lambda^{\mathrm{2}} −\mathrm{2}\lambda+\mathrm{1}=\mathrm{0}\Rightarrow\lambda_{\mathrm{1},\mathrm{2}} =\mathrm{1} \\ $$$$\Rightarrow{y}_{{h}} =\:{Ae}^{{x}} +{Bxe}^{{x}} \\ $$$${Particular}\:{solution}\: \\ $$$${W}\left({u}_{\mathrm{1}} ,{u}_{\mathrm{2}} \right)=\begin{vmatrix}{{e}^{{x}} \:\:\:\:\:\:\:\:\:{xe}^{{x}} }\\{{e}^{{x}} \:\:\:\:\:{e}^{{x}} +{xe}^{{x}} }\end{vmatrix}={e}^{\mathrm{2}{x}} \\ $$$${W}_{\mathrm{1}} =\begin{vmatrix}{\mathrm{0}\:\:\:\:\:\:\:\:\:\:\:\:\:\:{xe}^{{x}} }\\{\mathrm{3}{e}^{\mathrm{4}{x}} \:\:\:\:\:{e}^{{x}} +{xe}^{{x}} }\end{vmatrix}=−\mathrm{3}{xe}^{\mathrm{5}{x}} \\ $$$${W}_{\mathrm{2}} =\begin{vmatrix}{{e}^{{x}} \:\:\:\:\:\:\:\:\mathrm{0}}\\{{e}^{{x}} \:\:\:\:\:\:\mathrm{3}{e}^{\mathrm{4}{x}} }\end{vmatrix}=\mathrm{3}{e}^{\mathrm{5}{x}} \\ $$$$\Rightarrow{v}_{\mathrm{1}} =\int\:\frac{{w}_{\mathrm{1}} }{{w}}\:{dx}=\int\:\frac{−\mathrm{3}{xe}^{\mathrm{5}{x}} }{{e}^{\mathrm{2}{x}} }\:{dx} \\ $$$$\:\:\:\:\:\:\:\:\:\:=−\mathrm{3}\int{xe}^{\mathrm{3}{x}} \:{dx}\:=−\mathrm{3}\left[\:\frac{\mathrm{1}}{\mathrm{3}}{xe}^{\mathrm{3}{x}} −\frac{\mathrm{1}}{\mathrm{9}}{e}^{\mathrm{3}{x}} \right]=−{xe}^{\mathrm{3}{x}} +\frac{\mathrm{1}}{\mathrm{3}}{e}^{\mathrm{3}{x}} \\ $$$$\Rightarrow{v}_{\mathrm{2}} =\int\:\frac{{w}_{\mathrm{2}} }{{w}}\:{dx}=\int\:\frac{\mathrm{3}{e}^{\mathrm{5}{x}} }{{e}^{\mathrm{2}{x}} }\:{dx}=\int\mathrm{3}{e}^{\mathrm{3}{x}} {dx}={e}^{\mathrm{3}{x}} \\ $$$$\Rightarrow{y}_{{p}} ={u}_{\mathrm{1}} {v}_{\mathrm{1}} +{u}_{\mathrm{2}} {v}_{\mathrm{2}} ={e}^{{x}} \left(−{xe}^{\mathrm{3}{x}} +\frac{\mathrm{1}}{\mathrm{3}}{e}^{\mathrm{3}{x}} \right)+{xe}^{{x}} \left({e}^{\mathrm{3}{x}} \right) \\ $$$$\Rightarrow{y}_{{p}} =\frac{\mathrm{1}}{\mathrm{3}}{e}^{\mathrm{4}{x}} \\ $$$${y}_{{g}} ={Ae}^{{x}} +{Bxe}^{{x}} +\frac{\mathrm{1}}{\mathrm{3}}{e}^{\mathrm{4}{x}} \\ $$$$\:\begin{cases}{{x}=\mathrm{0}\Rightarrow{A}+\frac{\mathrm{1}}{\mathrm{3}}=−\frac{\mathrm{2}}{\mathrm{3}}\:;\:{A}=−\mathrm{1}}\\{{y}'={Ae}^{{x}} +{Be}^{{x}} +{Bxe}^{{x}} +\frac{\mathrm{4}}{\mathrm{3}}{e}^{\mathrm{4}{x}} \:}\\{{y}'\left(\mathrm{0}\right)=−\mathrm{1}+{B}+\frac{\mathrm{4}}{\mathrm{3}}=\frac{\mathrm{13}}{\mathrm{3}}\Rightarrow{B}=\mathrm{4}\:}\end{cases} \\ $$$$\Leftrightarrow\:\therefore\:{y}=−{e}^{{x}} +\mathrm{4}{xe}^{{x}} +\frac{\mathrm{1}}{\mathrm{3}}{e}^{\mathrm{4}{x}} \\ $$$$\:\:\: \\ $$
Answered by qaz last updated on 21/Oct/21
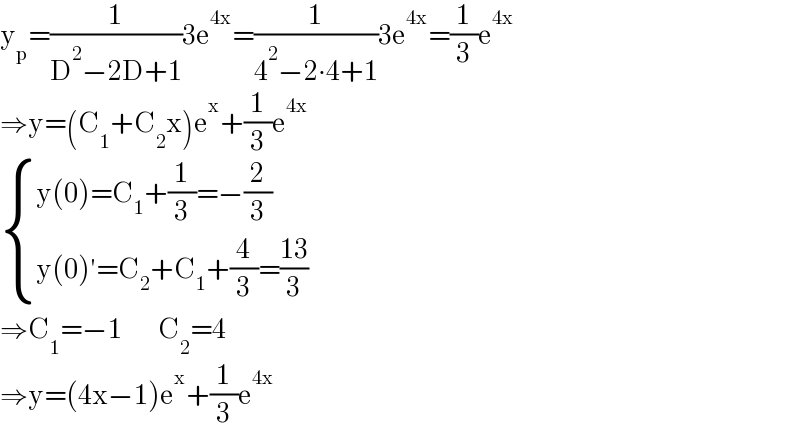
$$\mathrm{y}_{\mathrm{p}} =\frac{\mathrm{1}}{\mathrm{D}^{\mathrm{2}} −\mathrm{2D}+\mathrm{1}}\mathrm{3e}^{\mathrm{4x}} =\frac{\mathrm{1}}{\mathrm{4}^{\mathrm{2}} −\mathrm{2}\centerdot\mathrm{4}+\mathrm{1}}\mathrm{3e}^{\mathrm{4x}} =\frac{\mathrm{1}}{\mathrm{3}}\mathrm{e}^{\mathrm{4x}} \\ $$$$\Rightarrow\mathrm{y}=\left(\mathrm{C}_{\mathrm{1}} +\mathrm{C}_{\mathrm{2}} \mathrm{x}\right)\mathrm{e}^{\mathrm{x}} +\frac{\mathrm{1}}{\mathrm{3}}\mathrm{e}^{\mathrm{4x}} \\ $$$$\begin{cases}{\mathrm{y}\left(\mathrm{0}\right)=\mathrm{C}_{\mathrm{1}} +\frac{\mathrm{1}}{\mathrm{3}}=−\frac{\mathrm{2}}{\mathrm{3}}}\\{\mathrm{y}\left(\mathrm{0}\right)'=\mathrm{C}_{\mathrm{2}} +\mathrm{C}_{\mathrm{1}} +\frac{\mathrm{4}}{\mathrm{3}}=\frac{\mathrm{13}}{\mathrm{3}}}\end{cases} \\ $$$$\Rightarrow\mathrm{C}_{\mathrm{1}} =−\mathrm{1}\:\:\:\:\:\:\:\:\:\mathrm{C}_{\mathrm{2}} =\mathrm{4} \\ $$$$\Rightarrow\mathrm{y}=\left(\mathrm{4x}−\mathrm{1}\right)\mathrm{e}^{\mathrm{x}} +\frac{\mathrm{1}}{\mathrm{3}}\mathrm{e}^{\mathrm{4x}} \\ $$
