Menu Close

d-2-y-dx-2-a-2-y-cosax-

Tinku Tara 0 Comments
Pin




Question Number 117602 by TANMAY PANACEA last updated on 12/Oct/20
(d^2 y/dx^2 )+a^2 y=cosax
$$\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }+{a}^{\mathrm{2}} {y}={cosax} \\ $$
Answered by TANMAY PANACEA last updated on 12/Oct/20
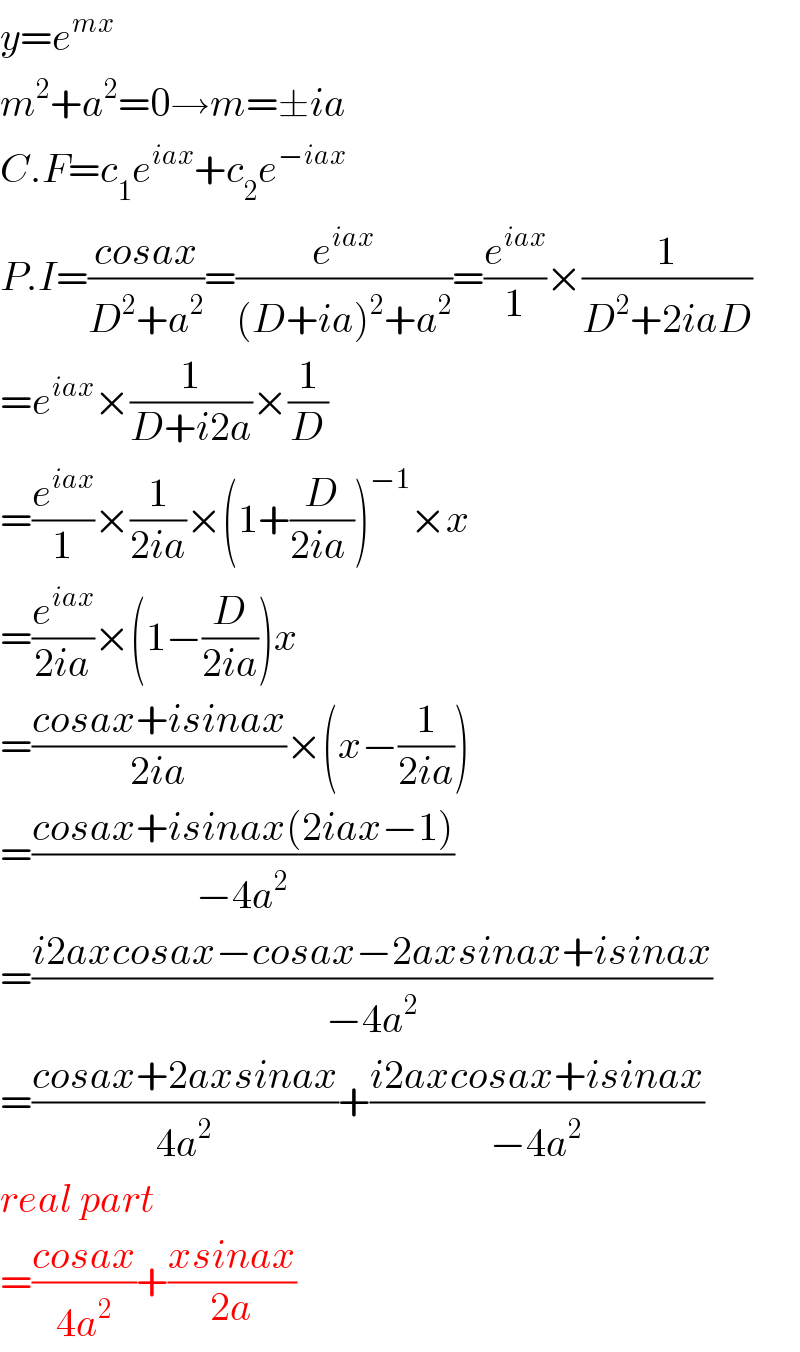
y=e^(mx) m^2 +a^2 =0→m=±ia C.F=c_1 e^(iax) +c_2 e^(−iax) P.I=((cosax)/(D^2 +a^2 ))=(e^(iax) /((D+ia)^2 +a^2 ))=(e^(iax) /1)×(1/(D^2 +2iaD)) =e^(iax) ×(1/(D+i2a))×(1/D) =(e^(iax) /1)×(1/(2ia))×(1+(D/(2ia )))^(−1) ×x =(e^(iax) /(2ia))×(1−(D/(2ia)))x =((cosax+isinax)/(2ia))×(x−(1/(2ia))) =((cosax+isinax(2iax−1))/(−4a^2 )) =((i2axcosax−cosax−2axsinax+isinax)/(−4a^2 )) =((cosax+2axsinax)/(4a^2 ))+((i2axcosax+isinax)/(−4a^2 )) real part =((cosax)/(4a^2 ))+((xsinax)/(2a))
$${y}={e}^{{mx}} \\ $$$${m}^{\mathrm{2}} +{a}^{\mathrm{2}} =\mathrm{0}\rightarrow{m}=\pm{ia} \\ $$$${C}.{F}={c}_{\mathrm{1}} {e}^{{iax}} +{c}_{\mathrm{2}} {e}^{−{iax}} \\ $$$${P}.{I}=\frac{{cosax}}{{D}^{\mathrm{2}} +{a}^{\mathrm{2}} }=\frac{{e}^{{iax}} }{\left({D}+{ia}\right)^{\mathrm{2}} +{a}^{\mathrm{2}} }=\frac{{e}^{{iax}} }{\mathrm{1}}×\frac{\mathrm{1}}{{D}^{\mathrm{2}} +\mathrm{2}{iaD}} \\ $$$$={e}^{{iax}} ×\frac{\mathrm{1}}{{D}+{i}\mathrm{2}{a}}×\frac{\mathrm{1}}{{D}} \\ $$$$=\frac{{e}^{{iax}} }{\mathrm{1}}×\frac{\mathrm{1}}{\mathrm{2}{ia}}×\left(\mathrm{1}+\frac{{D}}{\mathrm{2}{ia}\:}\right)^{−\mathrm{1}} ×{x} \\ $$$$=\frac{{e}^{{iax}} }{\mathrm{2}{ia}}×\left(\mathrm{1}−\frac{{D}}{\mathrm{2}{ia}}\right){x} \\ $$$$=\frac{{cosax}+{isinax}}{\mathrm{2}{ia}}×\left({x}−\frac{\mathrm{1}}{\mathrm{2}{ia}}\right) \\ $$$$=\frac{{cosax}+{isinax}\left(\mathrm{2}{iax}−\mathrm{1}\right)}{−\mathrm{4}{a}^{\mathrm{2}} } \\ $$$$=\frac{{i}\mathrm{2}{axcosax}−{cosax}−\mathrm{2}{axsinax}+{isinax}}{−\mathrm{4}{a}^{\mathrm{2}} } \\ $$$$=\frac{{cosax}+\mathrm{2}{axsinax}}{\mathrm{4}{a}^{\mathrm{2}} }+\frac{{i}\mathrm{2}{axcosax}+{isinax}}{−\mathrm{4}{a}^{\mathrm{2}} } \\ $$$${real}\:{part} \\ $$$$=\frac{{cosax}}{\mathrm{4}{a}^{\mathrm{2}} }+\frac{{xsinax}}{\mathrm{2}{a}} \\ $$
Answered by mathmax by abdo last updated on 12/Oct/20
y^(′′) +a^2 y =cos(ax) h→r^2 +a^2 =0 ⇒r =+^− ia ⇒y_h =me^(iax) +n e^(−iax) =αcos(ax) +βsin(ax) =αu_1 (x)+β u_2 (x) W(u_1 ,u_2 ) = determinant (((cos(ax) sin(ax))),((−asin(ax) acos(ax))))=a we supoose a≠0 W_1 = determinant (((o sin(ax))),((cos(ax) acos(ax))))=−cos(ax)sin(ax) W_2 = determinant (((cos(ax) 0)),((−asin(ax) cos(ax))))=cos^2 (ax) v_1 =∫ (W_1 /W)dx =−∫ ((cos(ax)sin(ax))/a) =−(1/(2a))∫ sin(2ax)dx =(1/(4a^2 ))cos(2ax) v_2 =∫ (W_2 /W)dx =∫ ((cos^2 (ax))/a)dx =(1/(2a))∫(1+cos(2ax))dx =(x/(2a)) +(1/(4a^2 ))sin(2ax) ⇒ y_p =u_1 v_1 +u_2 v_2 =cos(ax)((1/(4a^2 ))cos(2ax)) +sin(ax)((x/(2a))+(1/(4a^2 ))sin(2ax) =((cos(ax)cos(2ax))/(4a^2 )) +((xsin(ax))/(2a)) +((sin(ax)sin(2ax))/(4a^2 )) the general solution is y =y_h +y_p
$$\mathrm{y}^{''} \:+\mathrm{a}^{\mathrm{2}} \mathrm{y}\:=\mathrm{cos}\left(\mathrm{ax}\right) \\ $$$$\mathrm{h}\rightarrow\mathrm{r}^{\mathrm{2}} +\mathrm{a}^{\mathrm{2}} \:=\mathrm{0}\:\Rightarrow\mathrm{r}\:=\overset{−} {+}\mathrm{ia}\:\Rightarrow\mathrm{y}_{\mathrm{h}} =\mathrm{me}^{\mathrm{iax}} \:+\mathrm{n}\:\mathrm{e}^{−\mathrm{iax}} \\ $$$$=\alpha\mathrm{cos}\left(\mathrm{ax}\right)\:+\beta\mathrm{sin}\left(\mathrm{ax}\right)\:=\alpha\mathrm{u}_{\mathrm{1}} \left(\mathrm{x}\right)+\beta\:\mathrm{u}_{\mathrm{2}} \left(\mathrm{x}\right) \\ $$$$\mathrm{W}\left(\mathrm{u}_{\mathrm{1}} ,\mathrm{u}_{\mathrm{2}} \right)\:=\begin{vmatrix}{\mathrm{cos}\left(\mathrm{ax}\right)\:\:\:\:\:\:\:\:\:\mathrm{sin}\left(\mathrm{ax}\right)}\\{−\mathrm{asin}\left(\mathrm{ax}\right)\:\:\:\:\:\:\mathrm{acos}\left(\mathrm{ax}\right)}\end{vmatrix}=\mathrm{a}\:\:\mathrm{we}\:\mathrm{supoose}\:\mathrm{a}\neq\mathrm{0} \\ $$$$\mathrm{W}_{\mathrm{1}} =\begin{vmatrix}{\mathrm{o}\:\:\:\:\:\:\:\:\:\:\mathrm{sin}\left(\mathrm{ax}\right)}\\{\mathrm{cos}\left(\mathrm{ax}\right)\:\:\:\mathrm{acos}\left(\mathrm{ax}\right)}\end{vmatrix}=−\mathrm{cos}\left(\mathrm{ax}\right)\mathrm{sin}\left(\mathrm{ax}\right) \\ $$$$\mathrm{W}_{\mathrm{2}} =\begin{vmatrix}{\mathrm{cos}\left(\mathrm{ax}\right)\:\:\:\:\:\:\mathrm{0}}\\{−\mathrm{asin}\left(\mathrm{ax}\right)\:\:\mathrm{cos}\left(\mathrm{ax}\right)}\end{vmatrix}=\mathrm{cos}^{\mathrm{2}} \left(\mathrm{ax}\right) \\ $$$$\mathrm{v}_{\mathrm{1}} =\int\:\frac{\mathrm{W}_{\mathrm{1}} }{\mathrm{W}}\mathrm{dx}\:=−\int\:\:\frac{\mathrm{cos}\left(\mathrm{ax}\right)\mathrm{sin}\left(\mathrm{ax}\right)}{\mathrm{a}}\:=−\frac{\mathrm{1}}{\mathrm{2a}}\int\:\mathrm{sin}\left(\mathrm{2ax}\right)\mathrm{dx} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4a}^{\mathrm{2}} }\mathrm{cos}\left(\mathrm{2ax}\right) \\ $$$$\mathrm{v}_{\mathrm{2}} =\int\:\frac{\mathrm{W}_{\mathrm{2}} }{\mathrm{W}}\mathrm{dx}\:=\int\:\frac{\mathrm{cos}^{\mathrm{2}} \left(\mathrm{ax}\right)}{\mathrm{a}}\mathrm{dx}\:=\frac{\mathrm{1}}{\mathrm{2a}}\int\left(\mathrm{1}+\mathrm{cos}\left(\mathrm{2ax}\right)\right)\mathrm{dx} \\ $$$$=\frac{\mathrm{x}}{\mathrm{2a}}\:+\frac{\mathrm{1}}{\mathrm{4a}^{\mathrm{2}} }\mathrm{sin}\left(\mathrm{2ax}\right)\:\Rightarrow \\ $$$$\mathrm{y}_{\mathrm{p}} =\mathrm{u}_{\mathrm{1}} \mathrm{v}_{\mathrm{1}} +\mathrm{u}_{\mathrm{2}} \mathrm{v}_{\mathrm{2}} =\mathrm{cos}\left(\mathrm{ax}\right)\left(\frac{\mathrm{1}}{\mathrm{4a}^{\mathrm{2}} }\mathrm{cos}\left(\mathrm{2ax}\right)\right)\:+\mathrm{sin}\left(\mathrm{ax}\right)\left(\frac{\mathrm{x}}{\mathrm{2a}}+\frac{\mathrm{1}}{\mathrm{4a}^{\mathrm{2}} }\mathrm{sin}\left(\mathrm{2ax}\right)\right. \\ $$$$=\frac{\mathrm{cos}\left(\mathrm{ax}\right)\mathrm{cos}\left(\mathrm{2ax}\right)}{\mathrm{4a}^{\mathrm{2}} }\:+\frac{\mathrm{xsin}\left(\mathrm{ax}\right)}{\mathrm{2a}}\:+\frac{\mathrm{sin}\left(\mathrm{ax}\right)\mathrm{sin}\left(\mathrm{2ax}\right)}{\mathrm{4a}^{\mathrm{2}} } \\ $$$$\mathrm{the}\:\mathrm{general}\:\mathrm{solution}\:\mathrm{is}\:\mathrm{y}\:=\mathrm{y}_{\mathrm{h}} \:+\mathrm{y}_{\mathrm{p}} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *