Question Number 104893 by bramlex last updated on 24/Jul/20
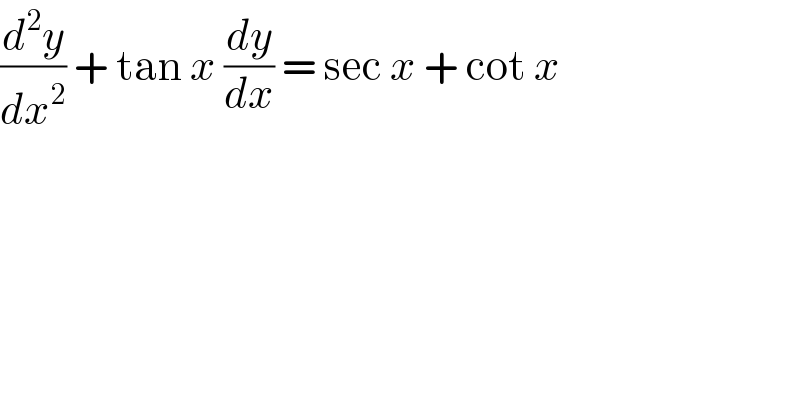
$$\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }\:+\:\mathrm{tan}\:{x}\:\frac{{dy}}{{dx}}\:=\:\mathrm{sec}\:{x}\:+\:\mathrm{cot}\:{x} \\ $$
Answered by mathmax by abdo last updated on 24/Jul/20
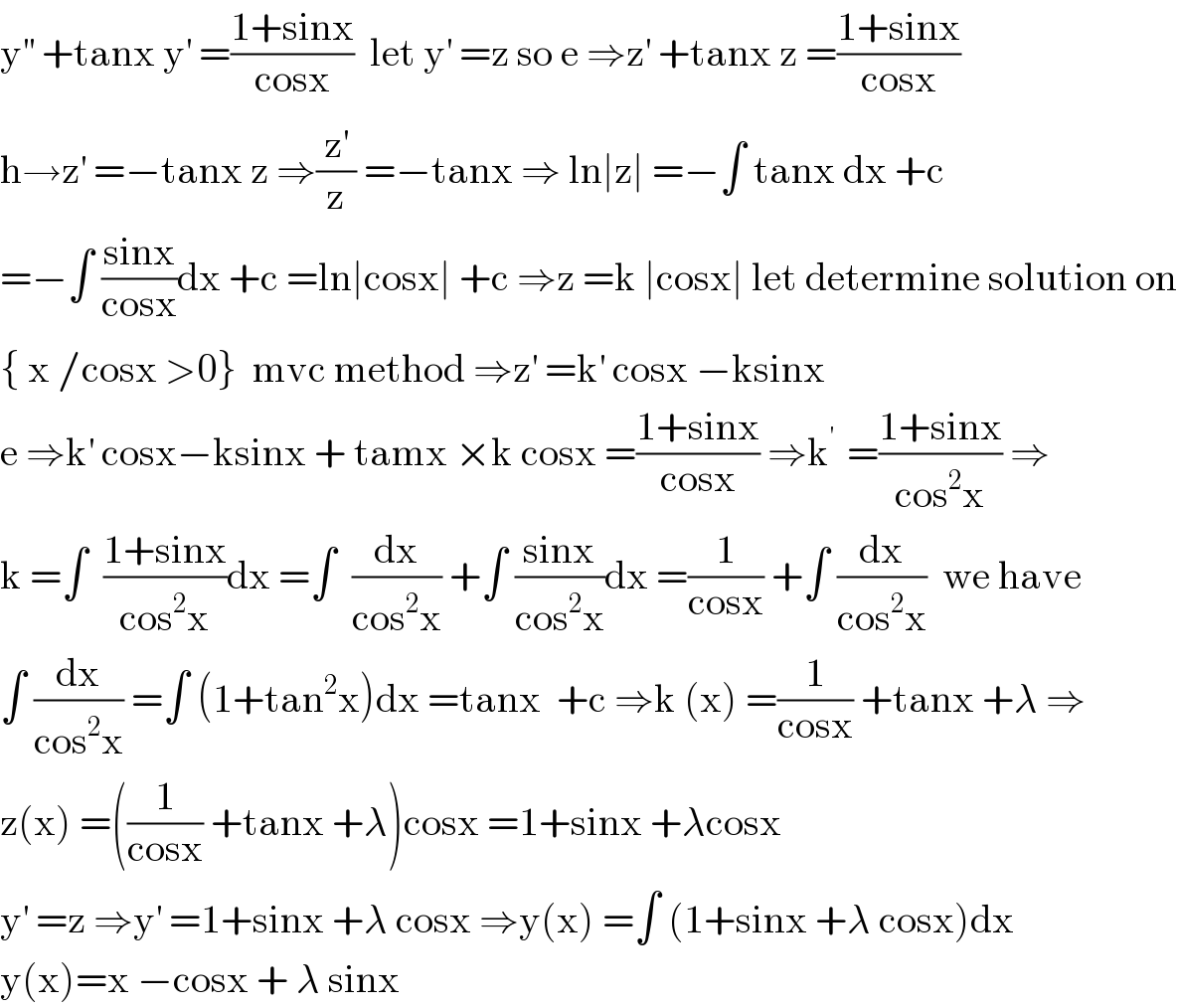
$$\mathrm{y}^{''} \:+\mathrm{tanx}\:\mathrm{y}^{'} \:=\frac{\mathrm{1}+\mathrm{sinx}}{\mathrm{cosx}}\:\:\mathrm{let}\:\mathrm{y}^{'} \:=\mathrm{z}\:\mathrm{so}\:\mathrm{e}\:\Rightarrow\mathrm{z}^{'} \:+\mathrm{tanx}\:\mathrm{z}\:=\frac{\mathrm{1}+\mathrm{sinx}}{\mathrm{cosx}} \\ $$$$\mathrm{h}\rightarrow\mathrm{z}^{'} \:=−\mathrm{tanx}\:\mathrm{z}\:\Rightarrow\frac{\mathrm{z}^{'} }{\mathrm{z}}\:=−\mathrm{tanx}\:\Rightarrow\:\mathrm{ln}\mid\mathrm{z}\mid\:=−\int\:\mathrm{tanx}\:\mathrm{dx}\:+\mathrm{c} \\ $$$$=−\int\:\frac{\mathrm{sinx}}{\mathrm{cosx}}\mathrm{dx}\:+\mathrm{c}\:=\mathrm{ln}\mid\mathrm{cosx}\mid\:+\mathrm{c}\:\Rightarrow\mathrm{z}\:=\mathrm{k}\:\mid\mathrm{cosx}\mid\:\mathrm{let}\:\mathrm{determine}\:\mathrm{solution}\:\mathrm{on} \\ $$$$\left\{\:\mathrm{x}\:/\mathrm{cosx}\:>\mathrm{0}\right\}\:\:\mathrm{mvc}\:\mathrm{method}\:\Rightarrow\mathrm{z}^{'} \:=\mathrm{k}^{'} \:\mathrm{cosx}\:−\mathrm{ksinx} \\ $$$$\mathrm{e}\:\Rightarrow\mathrm{k}^{'} \:\mathrm{cosx}−\mathrm{ksinx}\:+\:\mathrm{tamx}\:×\mathrm{k}\:\mathrm{cosx}\:=\frac{\mathrm{1}+\mathrm{sinx}}{\mathrm{cosx}}\:\Rightarrow\mathrm{k}^{'\:} \:=\frac{\mathrm{1}+\mathrm{sinx}}{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\:\Rightarrow \\ $$$$\mathrm{k}\:=\int\:\:\frac{\mathrm{1}+\mathrm{sinx}}{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\mathrm{dx}\:=\int\:\:\frac{\mathrm{dx}}{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\:+\int\:\frac{\mathrm{sinx}}{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\mathrm{dx}\:=\frac{\mathrm{1}}{\mathrm{cosx}}\:+\int\:\frac{\mathrm{dx}}{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\:\:\mathrm{we}\:\mathrm{have} \\ $$$$\int\:\frac{\mathrm{dx}}{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\:=\int\:\left(\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \mathrm{x}\right)\mathrm{dx}\:=\mathrm{tanx}\:\:+\mathrm{c}\:\Rightarrow\mathrm{k}\:\left(\mathrm{x}\right)\:=\frac{\mathrm{1}}{\mathrm{cosx}}\:+\mathrm{tanx}\:+\lambda\:\Rightarrow \\ $$$$\mathrm{z}\left(\mathrm{x}\right)\:=\left(\frac{\mathrm{1}}{\mathrm{cosx}}\:+\mathrm{tanx}\:+\lambda\right)\mathrm{cosx}\:=\mathrm{1}+\mathrm{sinx}\:+\lambda\mathrm{cosx} \\ $$$$\mathrm{y}^{'} \:=\mathrm{z}\:\Rightarrow\mathrm{y}^{'} \:=\mathrm{1}+\mathrm{sinx}\:+\lambda\:\mathrm{cosx}\:\Rightarrow\mathrm{y}\left(\mathrm{x}\right)\:=\int\:\left(\mathrm{1}+\mathrm{sinx}\:+\lambda\:\mathrm{cosx}\right)\mathrm{dx} \\ $$$$\mathrm{y}\left(\mathrm{x}\right)=\mathrm{x}\:−\mathrm{cosx}\:+\:\lambda\:\mathrm{sinx} \\ $$
Commented by mathmax by abdo last updated on 24/Jul/20
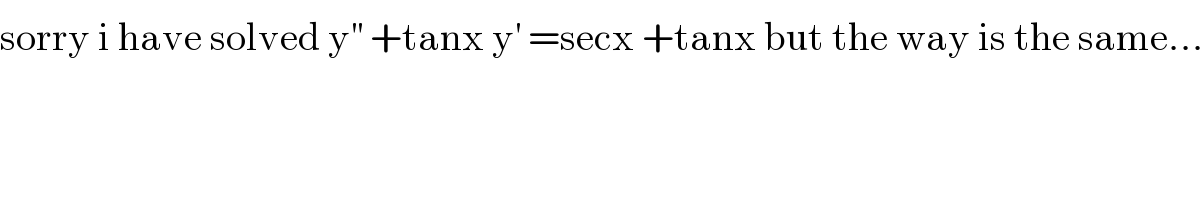
$$\mathrm{sorry}\:\mathrm{i}\:\mathrm{have}\:\mathrm{solved}\:\mathrm{y}^{''} \:+\mathrm{tanx}\:\mathrm{y}^{'} \:=\mathrm{secx}\:+\mathrm{tanx}\:\mathrm{but}\:\mathrm{the}\:\mathrm{way}\:\mathrm{is}\:\mathrm{the}\:\mathrm{same}… \\ $$
Commented by bramlex last updated on 24/Jul/20

$${ok}\:{sir}\:.\:{thank}\:{you} \\ $$
Commented by mathmax by abdo last updated on 24/Jul/20

$$\mathrm{you}\:\mathrm{are}\:\mathrm{welcome} \\ $$
