Question Number 100017 by bemath last updated on 24/Jun/20

$$\frac{\mathrm{d}^{\mathrm{2}} \mathrm{y}}{\mathrm{dx}^{\mathrm{2}} }\:+\:\mathrm{y}\:=\:\mathrm{sec}\:\mathrm{3x}\: \\ $$
Answered by mathmax by abdo last updated on 24/Jun/20
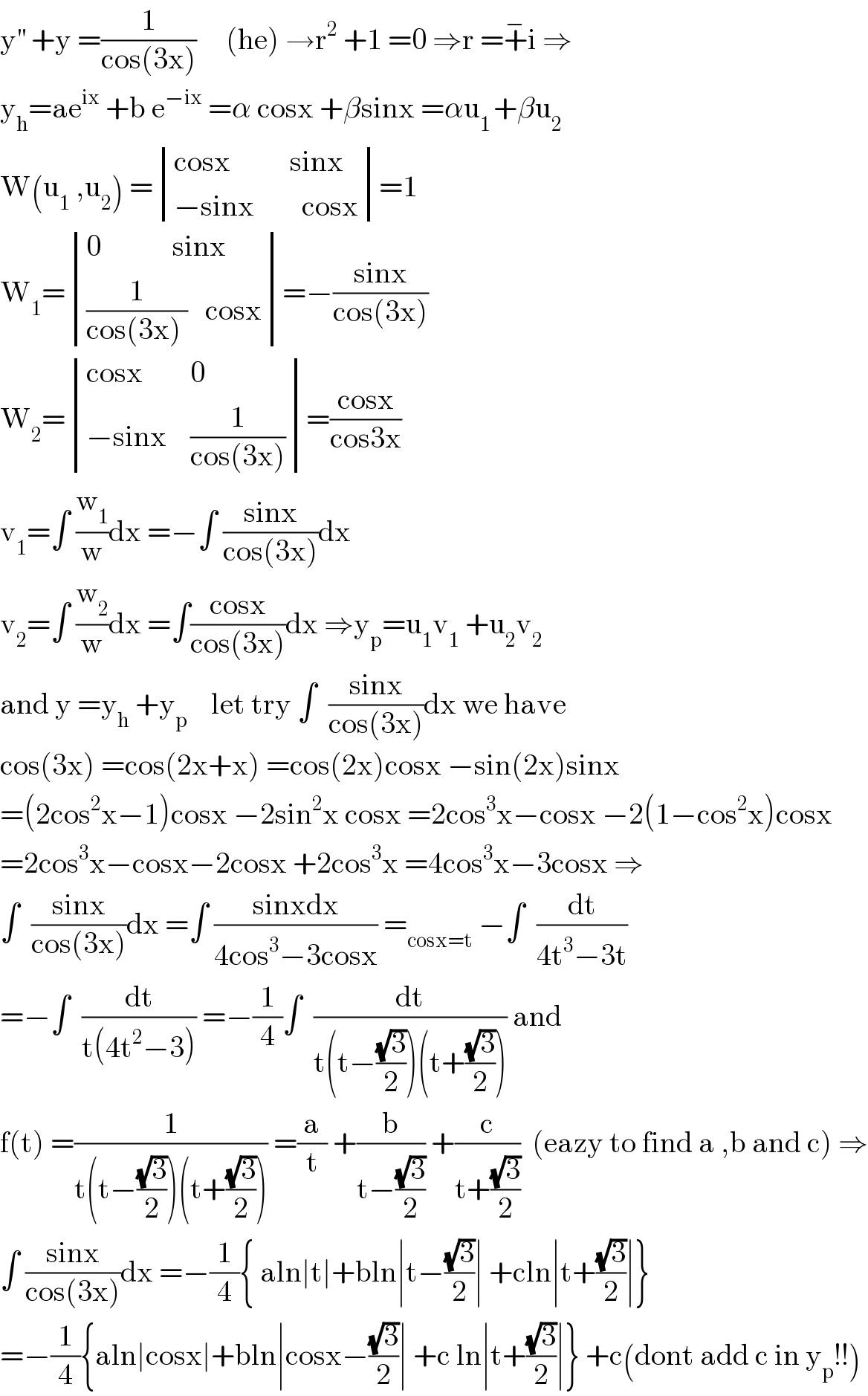
$$\mathrm{y}^{''} \:+\mathrm{y}\:=\frac{\mathrm{1}}{\mathrm{cos}\left(\mathrm{3x}\right)}\:\:\:\:\:\left(\mathrm{he}\right)\:\rightarrow\mathrm{r}^{\mathrm{2}} \:+\mathrm{1}\:=\mathrm{0}\:\Rightarrow\mathrm{r}\:=\overset{−} {+}\mathrm{i}\:\Rightarrow \\ $$$$\mathrm{y}_{\mathrm{h}} =\mathrm{ae}^{\mathrm{ix}} \:+\mathrm{b}\:\mathrm{e}^{−\mathrm{ix}} \:=\alpha\:\mathrm{cosx}\:+\beta\mathrm{sinx}\:=\alpha\mathrm{u}_{\mathrm{1}\:} +\beta\mathrm{u}_{\mathrm{2}} \\ $$$$\mathrm{W}\left(\mathrm{u}_{\mathrm{1}} \:,\mathrm{u}_{\mathrm{2}} \right)\:=\begin{vmatrix}{\mathrm{cosx}\:\:\:\:\:\:\:\:\:\:\mathrm{sinx}}\\{−\mathrm{sinx}\:\:\:\:\:\:\:\:\mathrm{cosx}}\end{vmatrix}=\mathrm{1} \\ $$$$\mathrm{W}_{\mathrm{1}} =\begin{vmatrix}{\mathrm{0}\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{sinx}}\\{\frac{\mathrm{1}}{\mathrm{cos}\left(\mathrm{3x}\right)\:}\:\:\:\mathrm{cosx}}\end{vmatrix}=−\frac{\mathrm{sinx}}{\mathrm{cos}\left(\mathrm{3x}\right)} \\ $$$$\mathrm{W}_{\mathrm{2}} =\begin{vmatrix}{\mathrm{cosx}\:\:\:\:\:\:\:\:\mathrm{0}}\\{−\mathrm{sinx}\:\:\:\:\frac{\mathrm{1}}{\mathrm{cos}\left(\mathrm{3x}\right)}}\end{vmatrix}=\frac{\mathrm{cosx}}{\mathrm{cos3x}} \\ $$$$\mathrm{v}_{\mathrm{1}} =\int\:\frac{\mathrm{w}_{\mathrm{1}} }{\mathrm{w}}\mathrm{dx}\:=−\int\:\frac{\mathrm{sinx}}{\mathrm{cos}\left(\mathrm{3x}\right)}\mathrm{dx} \\ $$$$\mathrm{v}_{\mathrm{2}} =\int\:\frac{\mathrm{w}_{\mathrm{2}} }{\mathrm{w}}\mathrm{dx}\:=\int\frac{\mathrm{cosx}}{\mathrm{cos}\left(\mathrm{3x}\right)}\mathrm{dx}\:\Rightarrow\mathrm{y}_{\mathrm{p}} =\mathrm{u}_{\mathrm{1}} \mathrm{v}_{\mathrm{1}} \:+\mathrm{u}_{\mathrm{2}} \mathrm{v}_{\mathrm{2}} \\ $$$$\mathrm{and}\:\mathrm{y}\:=\mathrm{y}_{\mathrm{h}} \:+\mathrm{y}_{\mathrm{p}} \:\:\:\:\mathrm{let}\:\mathrm{try}\:\int\:\:\frac{\mathrm{sinx}}{\mathrm{cos}\left(\mathrm{3x}\right)}\mathrm{dx}\:\mathrm{we}\:\mathrm{have} \\ $$$$\mathrm{cos}\left(\mathrm{3x}\right)\:=\mathrm{cos}\left(\mathrm{2x}+\mathrm{x}\right)\:=\mathrm{cos}\left(\mathrm{2x}\right)\mathrm{cosx}\:−\mathrm{sin}\left(\mathrm{2x}\right)\mathrm{sinx} \\ $$$$=\left(\mathrm{2cos}^{\mathrm{2}} \mathrm{x}−\mathrm{1}\right)\mathrm{cosx}\:−\mathrm{2sin}^{\mathrm{2}} \mathrm{x}\:\mathrm{cosx}\:=\mathrm{2cos}^{\mathrm{3}} \mathrm{x}−\mathrm{cosx}\:−\mathrm{2}\left(\mathrm{1}−\mathrm{cos}^{\mathrm{2}} \mathrm{x}\right)\mathrm{cosx} \\ $$$$=\mathrm{2cos}^{\mathrm{3}} \mathrm{x}−\mathrm{cosx}−\mathrm{2cosx}\:+\mathrm{2cos}^{\mathrm{3}} \mathrm{x}\:=\mathrm{4cos}^{\mathrm{3}} \mathrm{x}−\mathrm{3cosx}\:\Rightarrow \\ $$$$\int\:\:\frac{\mathrm{sinx}}{\mathrm{cos}\left(\mathrm{3x}\right)}\mathrm{dx}\:=\int\:\frac{\mathrm{sinxdx}}{\mathrm{4cos}^{\mathrm{3}} −\mathrm{3cosx}}\:=_{\mathrm{cosx}=\mathrm{t}} \:−\int\:\:\frac{\mathrm{dt}}{\mathrm{4t}^{\mathrm{3}} −\mathrm{3t}} \\ $$$$=−\int\:\:\frac{\mathrm{dt}}{\mathrm{t}\left(\mathrm{4t}^{\mathrm{2}} −\mathrm{3}\right)}\:=−\frac{\mathrm{1}}{\mathrm{4}}\int\:\:\frac{\mathrm{dt}}{\mathrm{t}\left(\mathrm{t}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)\left(\mathrm{t}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)}\:\mathrm{and} \\ $$$$\mathrm{f}\left(\mathrm{t}\right)\:=\frac{\mathrm{1}}{\mathrm{t}\left(\mathrm{t}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)\left(\mathrm{t}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)}\:=\frac{\mathrm{a}}{\mathrm{t}}\:+\frac{\mathrm{b}}{\mathrm{t}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}}\:+\frac{\mathrm{c}}{\mathrm{t}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}}\:\:\left(\mathrm{eazy}\:\mathrm{to}\:\mathrm{find}\:\mathrm{a}\:,\mathrm{b}\:\mathrm{and}\:\mathrm{c}\right)\:\Rightarrow \\ $$$$\int\:\frac{\mathrm{sinx}}{\mathrm{cos}\left(\mathrm{3x}\right)}\mathrm{dx}\:=−\frac{\mathrm{1}}{\mathrm{4}}\left\{\:\mathrm{aln}\mid\mathrm{t}\mid+\mathrm{bln}\mid\mathrm{t}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mid\:+\mathrm{cln}\mid\mathrm{t}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mid\right\} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}\left\{\mathrm{aln}\mid\mathrm{cosx}\mid+\mathrm{bln}\mid\mathrm{cosx}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mid\:+\mathrm{c}\:\mathrm{ln}\mid\mathrm{t}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\mid\right\}\:+\mathrm{c}\left(\mathrm{dont}\:\mathrm{add}\:\mathrm{c}\:\mathrm{in}\:\mathrm{y}_{\mathrm{p}} !!\right) \\ $$
