Question Number 158906 by SANOGO last updated on 10/Nov/21

$${determiner}\:{le}\:{reste}\:{de}\:{la}\:{division}\:{eucludienne}\:{de}: \\ $$$$\mathrm{10}^{\mathrm{100}} \:{par}\:\mathrm{105} \\ $$
Answered by Rasheed.Sindhi last updated on 11/Nov/21
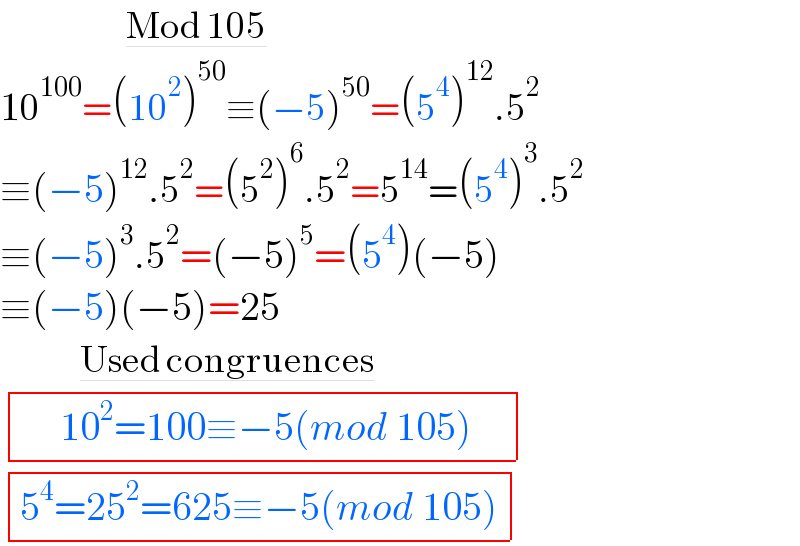
$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\underline{\mathrm{Mod}\:\mathrm{105}} \\ $$$$\mathrm{10}^{\mathrm{100}} =\left(\mathrm{10}^{\mathrm{2}} \right)^{\mathrm{50}} \equiv\left(−\mathrm{5}\right)^{\mathrm{50}} =\left(\mathrm{5}^{\mathrm{4}} \right)^{\mathrm{12}} .\mathrm{5}^{\mathrm{2}} \\ $$$$\equiv\left(−\mathrm{5}\right)^{\mathrm{12}} .\mathrm{5}^{\mathrm{2}} =\left(\mathrm{5}^{\mathrm{2}} \right)^{\mathrm{6}} .\mathrm{5}^{\mathrm{2}} =\mathrm{5}^{\mathrm{14}} =\left(\mathrm{5}^{\mathrm{4}} \right)^{\mathrm{3}} .\mathrm{5}^{\mathrm{2}} \\ $$$$\equiv\left(−\mathrm{5}\right)^{\mathrm{3}} .\mathrm{5}^{\mathrm{2}} =\left(−\mathrm{5}\right)^{\mathrm{5}} =\left(\mathrm{5}^{\mathrm{4}} \right)\left(−\mathrm{5}\right) \\ $$$$\equiv\left(−\mathrm{5}\right)\left(−\mathrm{5}\right)=\mathrm{25} \\ $$$$\:\:\:\:\:\:\:\:\:\:\underline{\mathrm{Used}\:\mathrm{congruences}}\:\:\:\:\:\: \\ $$$$\begin{array}{|c|}{\:\:\:\:\:\mathrm{10}^{\mathrm{2}} =\mathrm{100}\equiv−\mathrm{5}\left({mod}\:\mathrm{105}\right)\:\:\:\:}\\\hline\end{array}\: \\ $$$$\begin{array}{|c|}{\mathrm{5}^{\mathrm{4}} =\mathrm{25}^{\mathrm{2}} =\mathrm{625}\equiv−\mathrm{5}\left({mod}\:\mathrm{105}\right)}\\\hline\end{array}\: \\ $$
Commented by Rasheed.Sindhi last updated on 12/Nov/21
$${You}'{re}\:{welcome}\:{sir}! \\ $$
Commented by Rasheed.Sindhi last updated on 12/Nov/21

$${Why}\:{did}\:{you}\:{delete}\:{your}\:{answer}\:{sir}? \\ $$$${It}\:{would}\:{really}\:{require}\:{some} \\ $$$$\:\boldsymbol{{minor}}\:{changes}.\mathcal{T}{he}\:{logic}\:{was} \\ $$$${very}\:{correct}! \\ $$
Commented by SANOGO last updated on 11/Nov/21

$${merci}\:{bien} \\ $$
Commented by puissant last updated on 12/Nov/21

$${Yes}\:{sir}\:{my}\:{logic}\:{was}\:{correct}\:{but}\:{i}\:{did} \\ $$$${not}\:{achieve}\:{the}\:{result}\:{is}\:{proved}\:{that} \\ $$$${your}\:{logic}\:{is}\:{clearer}\:{really}\:{thank}\:{you}\: \\ $$$${very}\:{much}\:{sir}.. \\ $$
Commented by puissant last updated on 12/Nov/21

$${Thanks}\:{sir}… \\ $$
Commented by Rasheed.Sindhi last updated on 12/Nov/21
������
