Differentiate-the-following-wrt-x-1-y-x-x-2-y-sin-1-2x-1-Mastermind-

Question Number 169305 by Mastermind last updated on 28/Apr/22

D i f f e r e n t i a t e t h e f o l l o w i n g w r t x

1) y = x^{x}

2) y = {s i n}^{- 1} (2 x + 1)

M a s t e r m i n d

Commented by infinityaction last updated on 28/Apr/22

\log y = x \log x

\frac{1}{y} \frac{d y}{d x} = 1 + \log x

\frac{d y}{d x} = x^{x} {1 + \log ∣ x ∣}

Commented by Mastermind last updated on 29/Apr/22

w h a t o f n o . 2 ?

Answered by rexford last updated on 28/Apr/22

(1) l n y = x l n x

\frac{y^{'}}{y} = l n x + 1

y^{'} = y [l n x + 1]

y^{'} = x^{x} [l n x + 1]

Answered by rexford last updated on 28/Apr/22

y^{'} = \frac{1}{\sqrt{1 - {(2 x + 1)}^{2}}} \times \frac{d}{d x} (2 x + 1)

Missing \left or extra \right

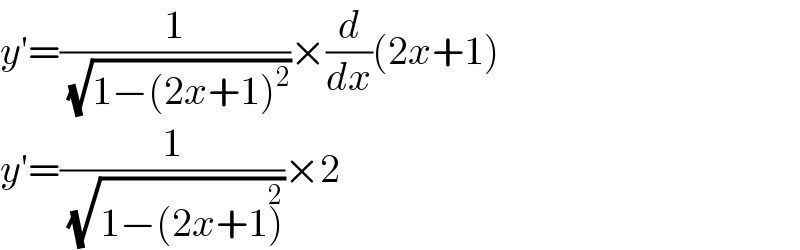
Commented by infinityaction last updated on 29/Apr/22

x = (- 1, 0)

Answered by thfchristopher last updated on 30/Apr/22

1) y = x^{x}

\Rightarrow \ln y = x \ln x

\Rightarrow \frac{1}{y} \frac{d y}{d x} = \frac{x}{x} + \ln x

\Rightarrow \frac{d y}{d x} = y (1 + \ln x)

= x^{x} (1 + \ln x)

2) y = \sin^{- 1} (2 x + 1)

\Rightarrow \sin y = 2 x + 1

\Rightarrow \cos y \frac{d y}{d x} = 2

\Rightarrow \sqrt{1 - {(2 x + 1)}^{2}} \frac{d y}{d x} = 2

\Rightarrow \frac{d y}{d x} = \frac{2}{\sqrt{1 - {(2 x + 1)}^{2}}}

Answered by MikeH last updated on 01/May/22

\ln y = x \ln x

\Rightarrow \frac{1}{y} \frac{d y}{d x} = \ln x + x (\frac{1}{x})

\Rightarrow \frac{d y}{d x} = y (\ln x + 1)

\Rightarrow \frac{d y}{d x} = x^{x} (\ln x + 1)

Facebook Tweet Pin