Menu Close

dx-a-cosx-2-a-gt-1-

Tinku Tara 0 Comments
Pin




Question Number 162410 by mahdipoor last updated on 29/Dec/21
∫(dx/((a−cosx)^2 )) a>1
$$\int\frac{{dx}}{\left({a}−{cosx}\right)^{\mathrm{2}} }\:\:\:{a}>\mathrm{1} \\ $$
Answered by mathmax by abdo last updated on 29/Dec/21
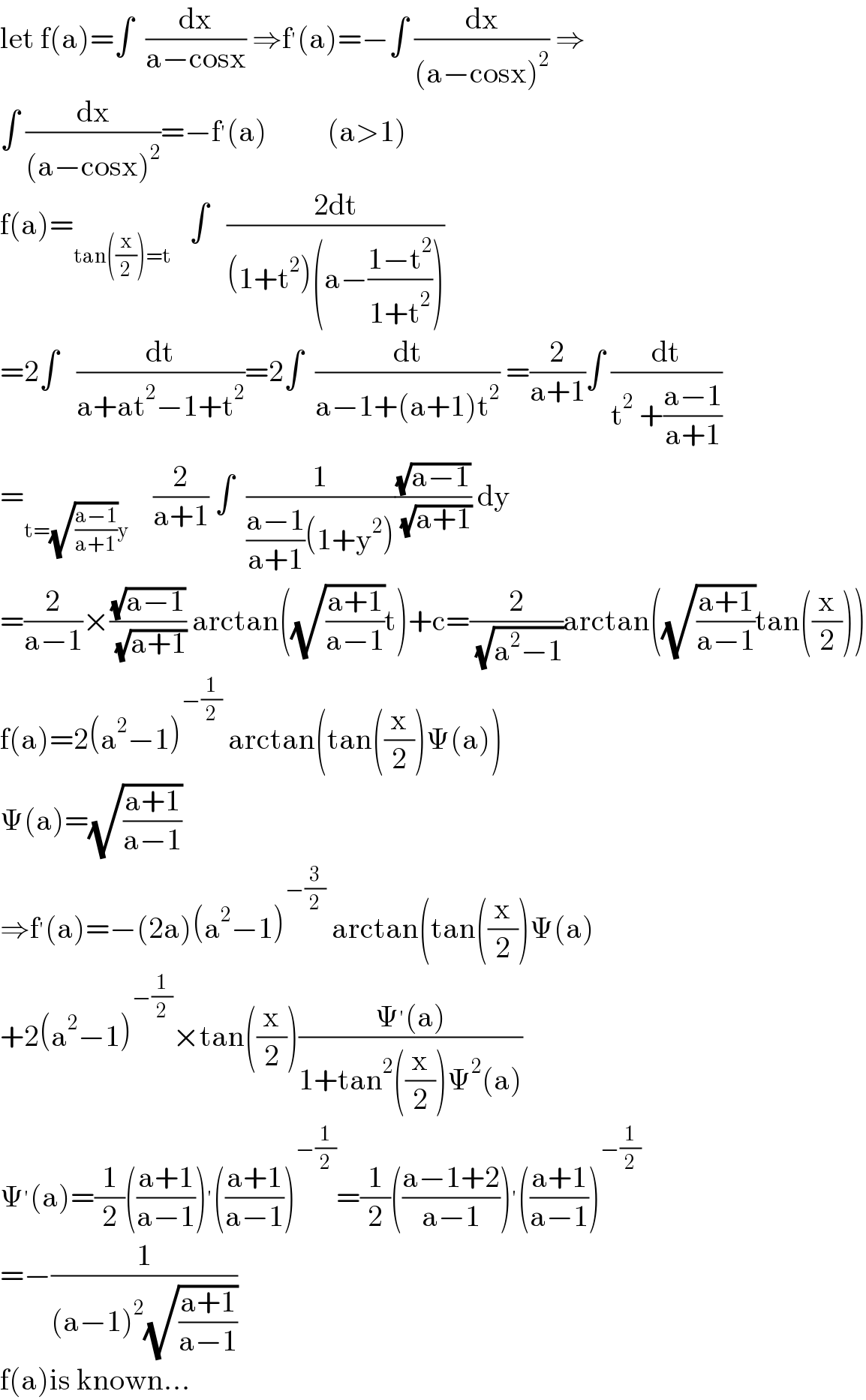
let f(a)=∫ (dx/(a−cosx)) ⇒f^′ (a)=−∫ (dx/((a−cosx)^2 )) ⇒ ∫ (dx/((a−cosx)^2 ))=−f^′ (a) (a>1) f(a)=_(tan((x/2))=t) ∫ ((2dt)/((1+t^2 )(a−((1−t^2 )/(1+t^2 ))))) =2∫ (dt/(a+at^2 −1+t^2 ))=2∫ (dt/(a−1+(a+1)t^2 )) =(2/(a+1))∫ (dt/(t^2 +((a−1)/(a+1)))) =_(t=(√((a−1)/(a+1)))y) (2/(a+1)) ∫ (1/(((a−1)/(a+1))(1+y^2 )))((√(a−1))/( (√(a+1)))) dy =(2/(a−1))×((√(a−1))/( (√(a+1)))) arctan((√((a+1)/(a−1)))t)+c=(2/( (√(a^2 −1))))arctan((√((a+1)/(a−1)))tan((x/2))) f(a)=2(a^2 −1)^(−(1/2)) arctan(tan((x/2))Ψ(a)) Ψ(a)=(√((a+1)/(a−1))) ⇒f^′ (a)=−(2a)(a^2 −1)^(−(3/2)) arctan(tan((x/2))Ψ(a) +2(a^2 −1)^(−(1/2)) ×tan((x/2))((Ψ^′ (a))/(1+tan^2 ((x/2))Ψ^2 (a))) Ψ^′ (a)=(1/2)(((a+1)/(a−1)))^′ (((a+1)/(a−1)))^(−(1/2)) =(1/2)(((a−1+2)/(a−1)))^′ (((a+1)/(a−1)))^(−(1/2)) =−(1/((a−1)^2 (√((a+1)/(a−1))))) f(a)is known...
$$\mathrm{let}\:\mathrm{f}\left(\mathrm{a}\right)=\int\:\:\frac{\mathrm{dx}}{\mathrm{a}−\mathrm{cosx}}\:\Rightarrow\mathrm{f}^{'} \left(\mathrm{a}\right)=−\int\:\frac{\mathrm{dx}}{\left(\mathrm{a}−\mathrm{cosx}\right)^{\mathrm{2}} }\:\Rightarrow \\ $$$$\int\:\frac{\mathrm{dx}}{\left(\mathrm{a}−\mathrm{cosx}\right)^{\mathrm{2}} }=−\mathrm{f}^{'} \left(\mathrm{a}\right)\:\:\:\:\:\:\:\:\:\:\left(\mathrm{a}>\mathrm{1}\right) \\ $$$$\mathrm{f}\left(\mathrm{a}\right)=_{\mathrm{tan}\left(\frac{\mathrm{x}}{\mathrm{2}}\right)=\mathrm{t}} \:\:\:\int\:\:\:\frac{\mathrm{2dt}}{\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\left(\mathrm{a}−\frac{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\right)} \\ $$$$=\mathrm{2}\int\:\:\:\frac{\mathrm{dt}}{\mathrm{a}+\mathrm{at}^{\mathrm{2}} −\mathrm{1}+\mathrm{t}^{\mathrm{2}} }=\mathrm{2}\int\:\:\frac{\mathrm{dt}}{\mathrm{a}−\mathrm{1}+\left(\mathrm{a}+\mathrm{1}\right)\mathrm{t}^{\mathrm{2}} }\:=\frac{\mathrm{2}}{\mathrm{a}+\mathrm{1}}\int\:\frac{\mathrm{dt}}{\mathrm{t}^{\mathrm{2}} \:+\frac{\mathrm{a}−\mathrm{1}}{\mathrm{a}+\mathrm{1}}} \\ $$$$=_{\mathrm{t}=\sqrt{\frac{\mathrm{a}−\mathrm{1}}{\mathrm{a}+\mathrm{1}}}\mathrm{y}} \:\:\:\:\frac{\mathrm{2}}{\mathrm{a}+\mathrm{1}}\:\int\:\:\frac{\mathrm{1}}{\frac{\mathrm{a}−\mathrm{1}}{\mathrm{a}+\mathrm{1}}\left(\mathrm{1}+\mathrm{y}^{\mathrm{2}} \right)}\frac{\sqrt{\mathrm{a}−\mathrm{1}}}{\:\sqrt{\mathrm{a}+\mathrm{1}}}\:\mathrm{dy} \\ $$$$=\frac{\mathrm{2}}{\mathrm{a}−\mathrm{1}}×\frac{\sqrt{\mathrm{a}−\mathrm{1}}}{\:\sqrt{\mathrm{a}+\mathrm{1}}}\:\mathrm{arctan}\left(\sqrt{\frac{\mathrm{a}+\mathrm{1}}{\mathrm{a}−\mathrm{1}}}\mathrm{t}\right)+\mathrm{c}=\frac{\mathrm{2}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} −\mathrm{1}}}\mathrm{arctan}\left(\sqrt{\frac{\mathrm{a}+\mathrm{1}}{\mathrm{a}−\mathrm{1}}}\mathrm{tan}\left(\frac{\mathrm{x}}{\mathrm{2}}\right)\right) \\ $$$$\mathrm{f}\left(\mathrm{a}\right)=\mathrm{2}\left(\mathrm{a}^{\mathrm{2}} −\mathrm{1}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \:\mathrm{arctan}\left(\mathrm{tan}\left(\frac{\mathrm{x}}{\mathrm{2}}\right)\Psi\left(\mathrm{a}\right)\right) \\ $$$$\Psi\left(\mathrm{a}\right)=\sqrt{\frac{\mathrm{a}+\mathrm{1}}{\mathrm{a}−\mathrm{1}}} \\ $$$$\Rightarrow\mathrm{f}^{'} \left(\mathrm{a}\right)=−\left(\mathrm{2a}\right)\left(\mathrm{a}^{\mathrm{2}} −\mathrm{1}\right)^{−\frac{\mathrm{3}}{\mathrm{2}}} \:\mathrm{arctan}\left(\mathrm{tan}\left(\frac{\mathrm{x}}{\mathrm{2}}\right)\Psi\left(\mathrm{a}\right)\right. \\ $$$$+\mathrm{2}\left(\mathrm{a}^{\mathrm{2}} −\mathrm{1}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} ×\mathrm{tan}\left(\frac{\mathrm{x}}{\mathrm{2}}\right)\frac{\Psi^{'} \left(\mathrm{a}\right)}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \left(\frac{\mathrm{x}}{\mathrm{2}}\right)\Psi^{\mathrm{2}} \left(\mathrm{a}\right)} \\ $$$$\Psi^{'} \left(\mathrm{a}\right)=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{a}+\mathrm{1}}{\mathrm{a}−\mathrm{1}}\right)^{'} \left(\frac{\mathrm{a}+\mathrm{1}}{\mathrm{a}−\mathrm{1}}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} =\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{a}−\mathrm{1}+\mathrm{2}}{\mathrm{a}−\mathrm{1}}\right)^{'} \left(\frac{\mathrm{a}+\mathrm{1}}{\mathrm{a}−\mathrm{1}}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$$=−\frac{\mathrm{1}}{\left(\mathrm{a}−\mathrm{1}\right)^{\mathrm{2}} \sqrt{\frac{\mathrm{a}+\mathrm{1}}{\mathrm{a}−\mathrm{1}}}} \\ $$$$\mathrm{f}\left(\mathrm{a}\right)\mathrm{is}\:\mathrm{known}… \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *