Question Number 163414 by AbdullahIbrahim last updated on 06/Jan/22
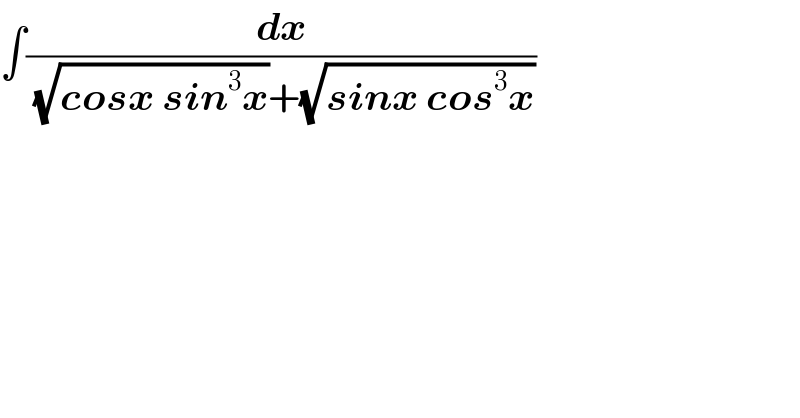
$$\int\frac{\boldsymbol{{dx}}}{\:\sqrt{\boldsymbol{{cosx}}\:\boldsymbol{{sin}}^{\mathrm{3}} \boldsymbol{{x}}}+\sqrt{\boldsymbol{{sinx}}\:\boldsymbol{{cos}}^{\mathrm{3}} \boldsymbol{{x}}}} \\ $$
Answered by Ar Brandon last updated on 06/Jan/22
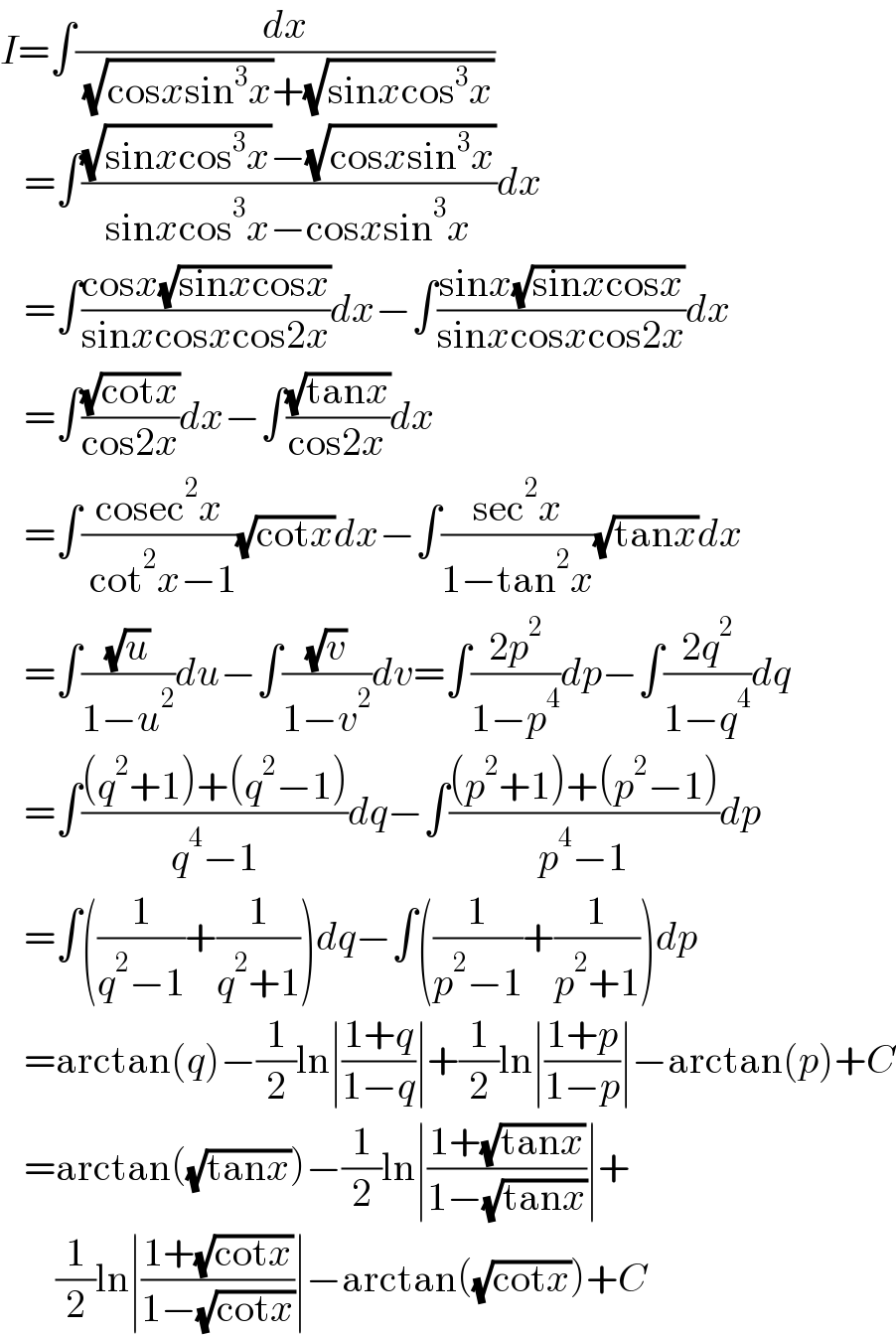
$${I}=\int\frac{{dx}}{\:\sqrt{\mathrm{cos}{x}\mathrm{sin}^{\mathrm{3}} {x}}+\sqrt{\mathrm{sin}{x}\mathrm{cos}^{\mathrm{3}} {x}}} \\ $$$$\:\:\:=\int\frac{\sqrt{\mathrm{sin}{x}\mathrm{cos}^{\mathrm{3}} {x}}−\sqrt{\mathrm{cos}{x}\mathrm{sin}^{\mathrm{3}} {x}}}{\mathrm{sin}{x}\mathrm{cos}^{\mathrm{3}} {x}−\mathrm{cos}{x}\mathrm{sin}^{\mathrm{3}} {x}}{dx} \\ $$$$\:\:\:=\int\frac{\mathrm{cos}{x}\sqrt{\mathrm{sin}{x}\mathrm{cos}{x}}}{\mathrm{sin}{x}\mathrm{cos}{x}\mathrm{cos2}{x}}{dx}−\int\frac{\mathrm{sin}{x}\sqrt{\mathrm{sin}{x}\mathrm{cos}{x}}}{\mathrm{sin}{x}\mathrm{cos}{x}\mathrm{cos2}{x}}{dx} \\ $$$$\:\:\:=\int\frac{\sqrt{\mathrm{cot}{x}}}{\mathrm{cos2}{x}}{dx}−\int\frac{\sqrt{\mathrm{tan}{x}}}{\mathrm{cos2}{x}}{dx} \\ $$$$\:\:\:=\int\frac{\mathrm{cosec}^{\mathrm{2}} {x}}{\:\mathrm{cot}^{\mathrm{2}} {x}−\mathrm{1}}\sqrt{\mathrm{cot}{x}}{dx}−\int\frac{\mathrm{sec}^{\mathrm{2}} {x}}{\mathrm{1}−\mathrm{tan}^{\mathrm{2}} {x}}\sqrt{\mathrm{tan}{x}}{dx} \\ $$$$\:\:\:=\int\frac{\sqrt{{u}}}{\mathrm{1}−{u}^{\mathrm{2}} }{du}−\int\frac{\sqrt{{v}}}{\mathrm{1}−{v}^{\mathrm{2}} }{dv}=\int\frac{\mathrm{2}{p}^{\mathrm{2}} }{\mathrm{1}−{p}^{\mathrm{4}} }{dp}−\int\frac{\mathrm{2}{q}^{\mathrm{2}} }{\mathrm{1}−{q}^{\mathrm{4}} }{dq} \\ $$$$\:\:\:=\int\frac{\left({q}^{\mathrm{2}} +\mathrm{1}\right)+\left({q}^{\mathrm{2}} −\mathrm{1}\right)}{{q}^{\mathrm{4}} −\mathrm{1}}{dq}−\int\frac{\left({p}^{\mathrm{2}} +\mathrm{1}\right)+\left({p}^{\mathrm{2}} −\mathrm{1}\right)}{{p}^{\mathrm{4}} −\mathrm{1}}{dp} \\ $$$$\:\:\:=\int\left(\frac{\mathrm{1}}{{q}^{\mathrm{2}} −\mathrm{1}}+\frac{\mathrm{1}}{{q}^{\mathrm{2}} +\mathrm{1}}\right){dq}−\int\left(\frac{\mathrm{1}}{{p}^{\mathrm{2}} −\mathrm{1}}+\frac{\mathrm{1}}{{p}^{\mathrm{2}} +\mathrm{1}}\right){dp} \\ $$$$\:\:\:=\mathrm{arctan}\left({q}\right)−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\frac{\mathrm{1}+{q}}{\mathrm{1}−{q}}\mid+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\frac{\mathrm{1}+{p}}{\mathrm{1}−{p}}\mid−\mathrm{arctan}\left({p}\right)+{C} \\ $$$$\:\:\:=\mathrm{arctan}\left(\sqrt{\mathrm{tan}{x}}\right)−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\frac{\mathrm{1}+\sqrt{\mathrm{tan}{x}}}{\mathrm{1}−\sqrt{\mathrm{tan}{x}}}\mid+ \\ $$$$\:\:\:\:\:\:\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\frac{\mathrm{1}+\sqrt{\mathrm{cot}{x}}}{\mathrm{1}−\sqrt{\mathrm{cot}{x}}}\mid−\mathrm{arctan}\left(\sqrt{\mathrm{cot}{x}}\right)+{C} \\ $$
Commented by peter frank last updated on 07/Jan/22

$$\mathrm{great} \\ $$
