Menu Close

dx-x-3-3x-5-

Tinku Tara 0 Comments
Pin




Question Number 111206 by mohammad17 last updated on 02/Sep/20
∫ (dx/(x^3 +3x−5))
$$\int\:\frac{{dx}}{{x}^{\mathrm{3}} +\mathrm{3}{x}−\mathrm{5}} \\ $$
Answered by Sarah85 last updated on 02/Sep/20
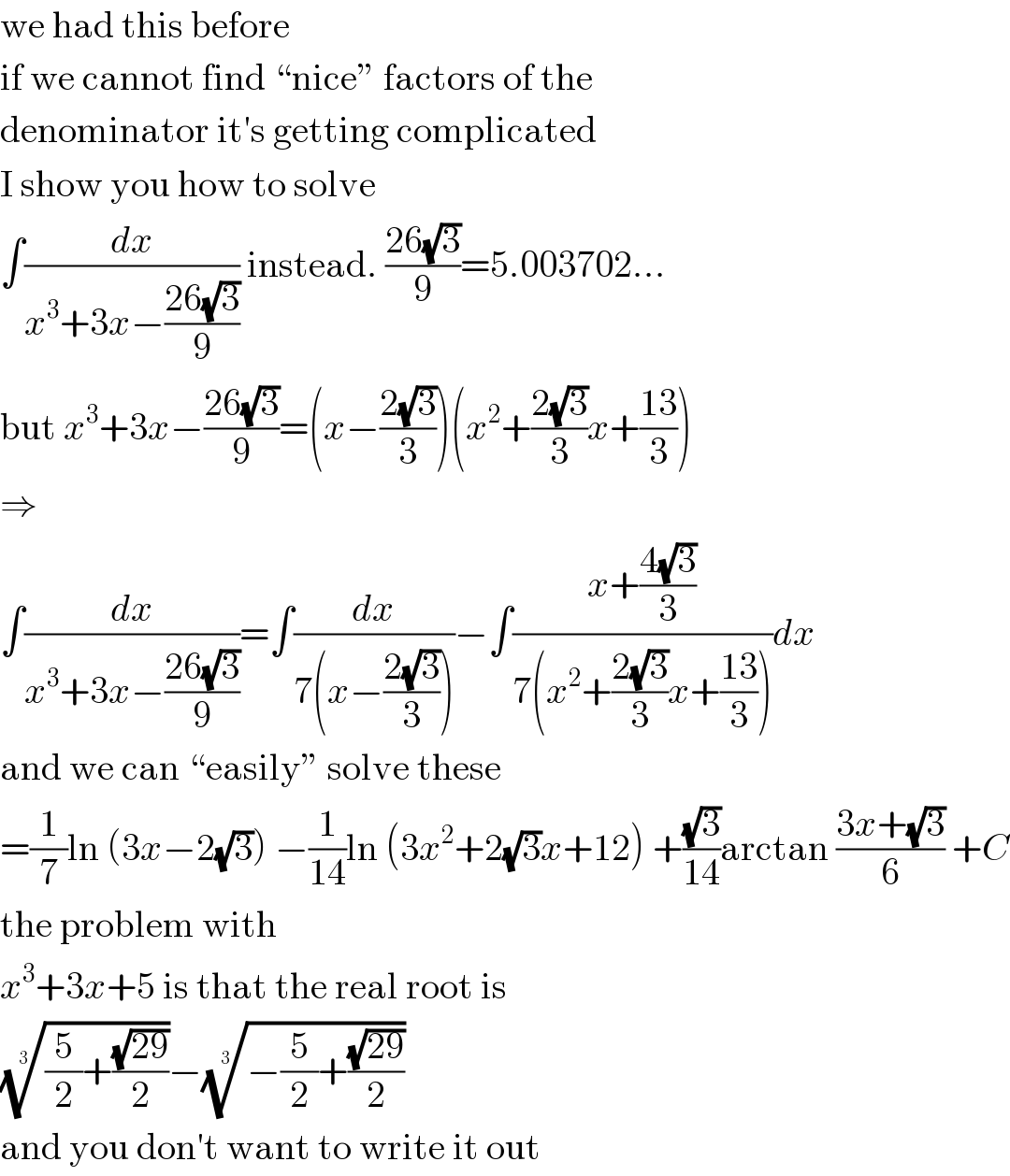
we had this before if we cannot find “nice” factors of the denominator it′s getting complicated I show you how to solve ∫(dx/(x^3 +3x−((26(√3))/9))) instead. ((26(√3))/9)=5.003702... but x^3 +3x−((26(√3))/9)=(x−((2(√3))/3))(x^2 +((2(√3))/3)x+((13)/3)) ⇒ ∫(dx/(x^3 +3x−((26(√3))/9)))=∫(dx/(7(x−((2(√3))/3))))−∫((x+((4(√3))/3))/(7(x^2 +((2(√3))/3)x+((13)/3))))dx and we can “easily” solve these =(1/7)ln (3x−2(√3)) −(1/(14))ln (3x^2 +2(√3)x+12) +((√3)/(14))arctan ((3x+(√3))/6) +C the problem with x^3 +3x+5 is that the real root is (((5/2)+((√(29))/2)))^(1/3) −((−(5/2)+((√(29))/2)))^(1/3) and you don′t want to write it out
$$\mathrm{we}\:\mathrm{had}\:\mathrm{this}\:\mathrm{before} \\ $$$$\mathrm{if}\:\mathrm{we}\:\mathrm{cannot}\:\mathrm{find}\:“\mathrm{nice}''\:\mathrm{factors}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{denominator}\:\mathrm{it}'\mathrm{s}\:\mathrm{getting}\:\mathrm{complicated} \\ $$$$\mathrm{I}\:\mathrm{show}\:\mathrm{you}\:\mathrm{how}\:\mathrm{to}\:\mathrm{solve} \\ $$$$\int\frac{{dx}}{{x}^{\mathrm{3}} +\mathrm{3}{x}−\frac{\mathrm{26}\sqrt{\mathrm{3}}}{\mathrm{9}}}\:\mathrm{instead}.\:\frac{\mathrm{26}\sqrt{\mathrm{3}}}{\mathrm{9}}=\mathrm{5}.\mathrm{003702}… \\ $$$$\mathrm{but}\:{x}^{\mathrm{3}} +\mathrm{3}{x}−\frac{\mathrm{26}\sqrt{\mathrm{3}}}{\mathrm{9}}=\left({x}−\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{3}}\right)\left({x}^{\mathrm{2}} +\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{3}}{x}+\frac{\mathrm{13}}{\mathrm{3}}\right) \\ $$$$\Rightarrow \\ $$$$\int\frac{{dx}}{{x}^{\mathrm{3}} +\mathrm{3}{x}−\frac{\mathrm{26}\sqrt{\mathrm{3}}}{\mathrm{9}}}=\int\frac{{dx}}{\mathrm{7}\left({x}−\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{3}}\right)}−\int\frac{{x}+\frac{\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{3}}}{\mathrm{7}\left({x}^{\mathrm{2}} +\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{3}}{x}+\frac{\mathrm{13}}{\mathrm{3}}\right)}{dx} \\ $$$$\mathrm{and}\:\mathrm{we}\:\mathrm{can}\:“\mathrm{easily}''\:\mathrm{solve}\:\mathrm{these} \\ $$$$=\frac{\mathrm{1}}{\mathrm{7}}\mathrm{ln}\:\left(\mathrm{3}{x}−\mathrm{2}\sqrt{\mathrm{3}}\right)\:−\frac{\mathrm{1}}{\mathrm{14}}\mathrm{ln}\:\left(\mathrm{3}{x}^{\mathrm{2}} +\mathrm{2}\sqrt{\mathrm{3}}{x}+\mathrm{12}\right)\:+\frac{\sqrt{\mathrm{3}}}{\mathrm{14}}\mathrm{arctan}\:\frac{\mathrm{3}{x}+\sqrt{\mathrm{3}}}{\mathrm{6}}\:+{C} \\ $$$$\mathrm{the}\:\mathrm{problem}\:\mathrm{with} \\ $$$${x}^{\mathrm{3}} +\mathrm{3}{x}+\mathrm{5}\:\mathrm{is}\:\mathrm{that}\:\mathrm{the}\:\mathrm{real}\:\mathrm{root}\:\mathrm{is} \\ $$$$\sqrt[{\mathrm{3}}]{\frac{\mathrm{5}}{\mathrm{2}}+\frac{\sqrt{\mathrm{29}}}{\mathrm{2}}}−\sqrt[{\mathrm{3}}]{−\frac{\mathrm{5}}{\mathrm{2}}+\frac{\sqrt{\mathrm{29}}}{\mathrm{2}}} \\ $$$$\mathrm{and}\:\mathrm{you}\:\mathrm{don}'\mathrm{t}\:\mathrm{want}\:\mathrm{to}\:\mathrm{write}\:\mathrm{it}\:\mathrm{out} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *