Question Number 20243 by tammi last updated on 24/Aug/17

$$\int\frac{{dx}}{{x}+\sqrt{{x}}} \\ $$
Answered by $@ty@m last updated on 25/Aug/17
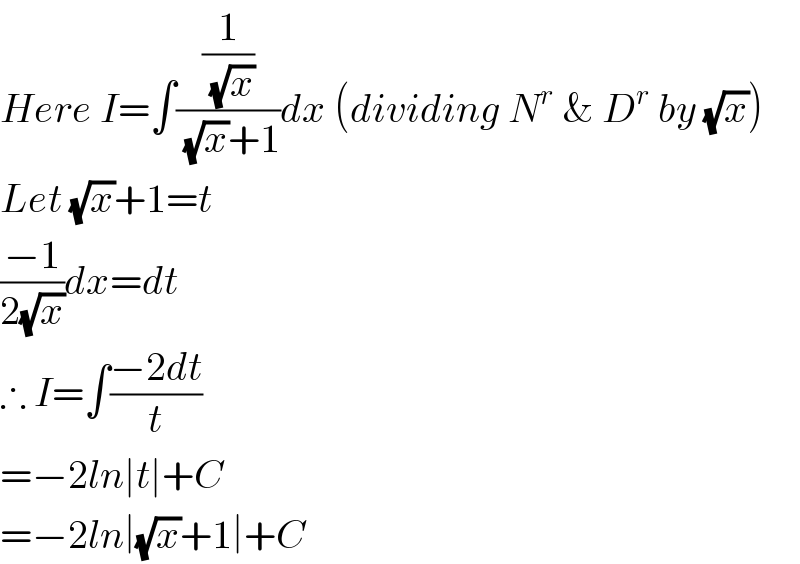
$${Here}\:{I}=\int\frac{\frac{\mathrm{1}}{\:\sqrt{{x}}}}{\:\sqrt{{x}}+\mathrm{1}}{dx}\:\left({dividing}\:{N}^{{r}} \:\&\:{D}^{{r}} \:{by}\:\sqrt{{x}}\right) \\ $$$${Let}\:\sqrt{{x}}+\mathrm{1}={t} \\ $$$$\frac{−\mathrm{1}}{\mathrm{2}\sqrt{{x}}}{dx}={dt} \\ $$$$\therefore\:{I}=\int\frac{−\mathrm{2}{dt}}{{t}} \\ $$$$=−\mathrm{2}{ln}\mid{t}\mid+{C} \\ $$$$=−\mathrm{2}{ln}\mid\sqrt{{x}}+\mathrm{1}\mid+{C} \\ $$
