dx-x-x-2-1-

Question Number 85637 by jagoll last updated on 23/Mar/20

\int \frac{dx}{x + \sqrt{x^{2} + 1}}

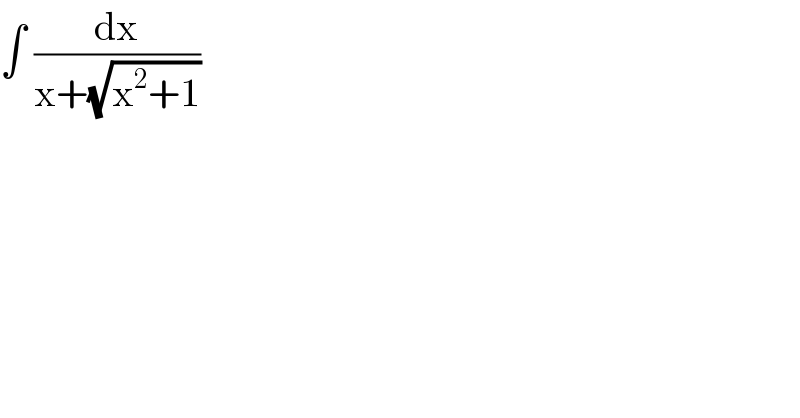
Commented by abdomathmax last updated on 23/Mar/20

I = \int \frac{d x}{x + \sqrt{x^{2} + 1}} w e d o t h e c h a n g e m e n t x = s h (t) \Rightarrow

I = \int \frac{c h t}{s h t + c h t} d t = \int \frac{e^{t} + e^{- t}}{e^{t} - e^{- t} + e^{t} + e^{- t}} d t

= \int \frac{e^{t} + e^{- t}}{2 e^{t}} d t ? = \int \frac{e^{- t}}{2} (e^{t} + e^{- t}) d t

= \frac{1}{2} \int (1 + e^{- 2 t}) d t = \frac{t}{2} - \frac{1}{4} e^{- 2 t} + c

t = l n (x + \sqrt{1 + x^{2}}) \Rightarrow e^{- 2 t} = \frac{1}{{(x + \sqrt{1 + x^{2}})}^{2}} \Rightarrow

I = \frac{1}{2} l n (x + \sqrt{1 + x^{2}}) - \frac{1}{4 {(x + \sqrt{1 + x^{2}})}^{2}} + c

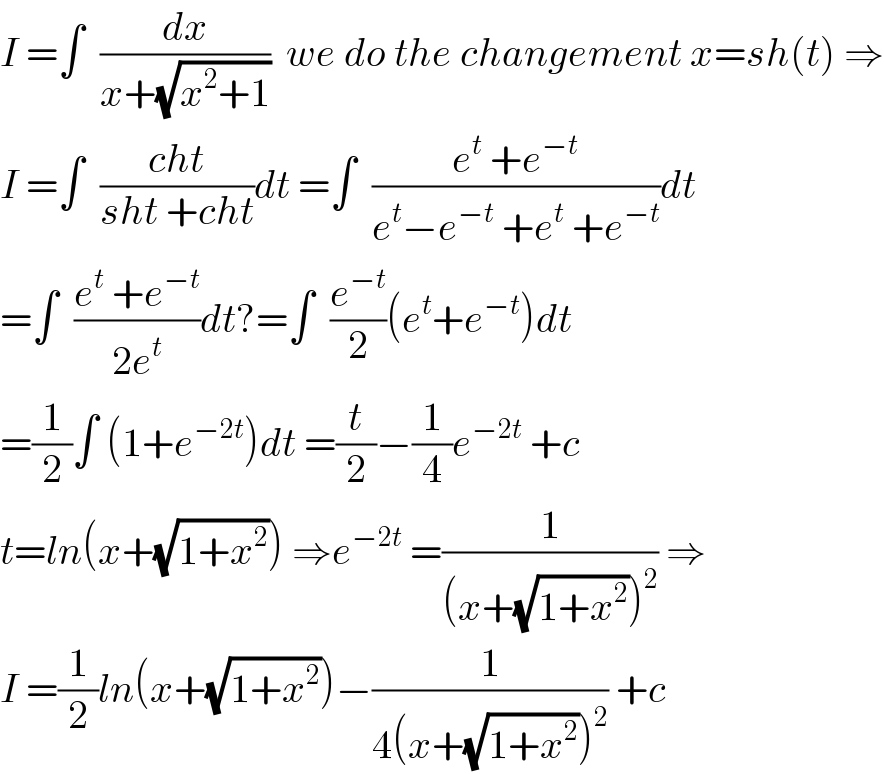
Commented by john santu last updated on 23/Mar/20

l e t x = \tan t

\int \frac{\sec^{2} t d t}{\tan t + \sec t} = \int \frac{d t}{\cos t (\sin t + 1)}

= \int \frac{\sin t - 1}{- \cos^{3} t} d t = \int \frac{1 - \sin t}{\cos^{3} t}

= \int \sec^{3} t d t + \int \frac{d (\cos t)}{\cos^{3} t}

= \frac{1}{2} \sec t \tan t + \frac{1}{2} \ln ∣ \sec t + \tan t ∣

- \frac{1}{2} \sec^{2} t + c

= \frac{1}{2} x \sqrt{1 + x^{2}} + \frac{1}{2} \ln ∣ x + \sqrt{1 + x^{2}} ∣ -

\frac{1}{2} (1 + x^{2}) + c

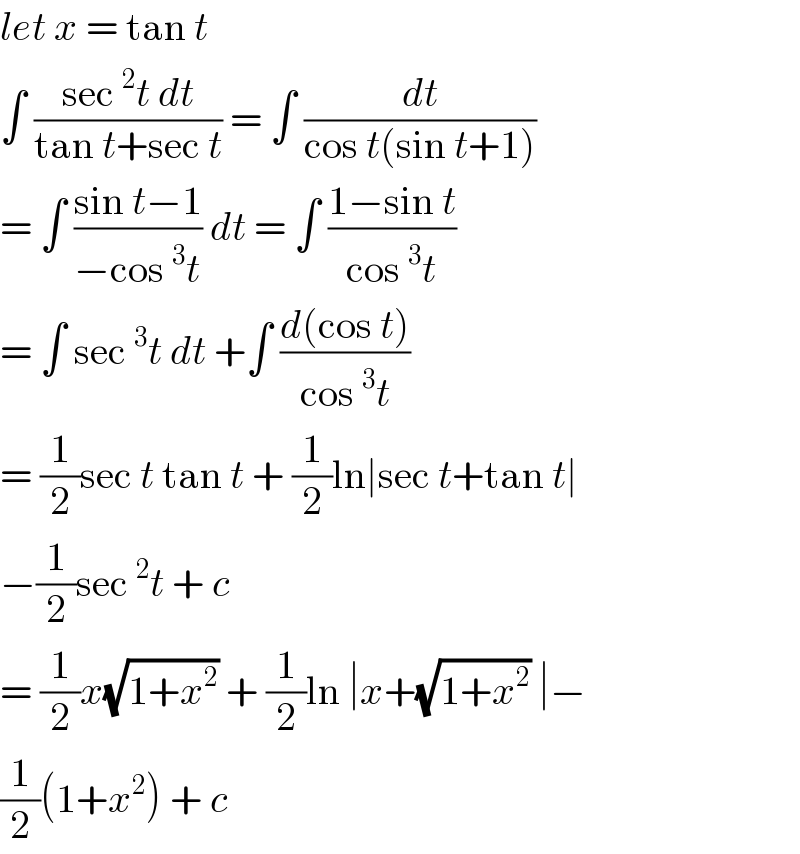
Answered by MJS last updated on 23/Mar/20

\int \frac{d x}{x + \sqrt{x^{2} + 1}} = - \int (x - \sqrt{x^{2} + 1}) d x =

= \int \sqrt{x^{2} + 1} d x - \int x d x and these are standard

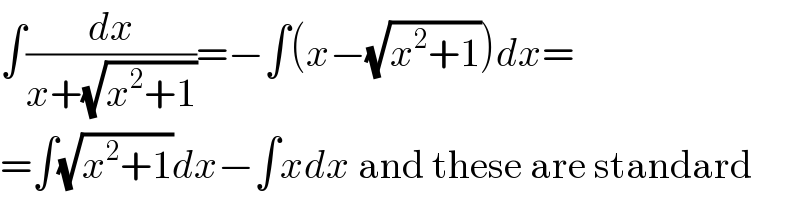
Commented by john santu last updated on 24/Mar/20

h a h a h a . . s t a n d a r d s i r