Question Number 121960 by bemath last updated on 13/Nov/20
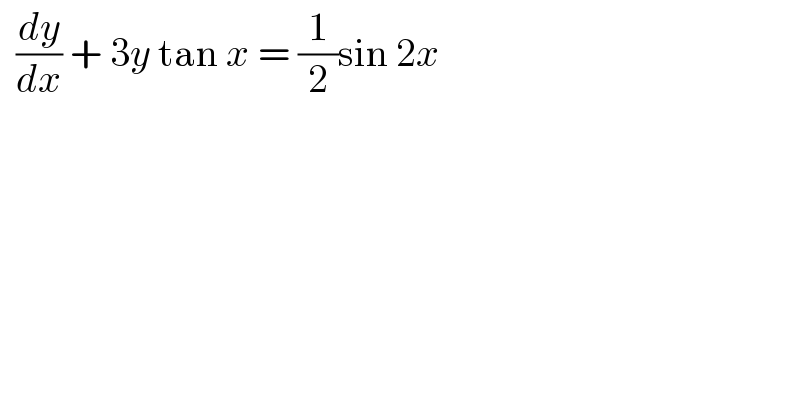
$$\:\:\frac{{dy}}{{dx}}\:+\:\mathrm{3}{y}\:\mathrm{tan}\:{x}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}\:\mathrm{2}{x}\: \\ $$
Answered by liberty last updated on 13/Nov/20
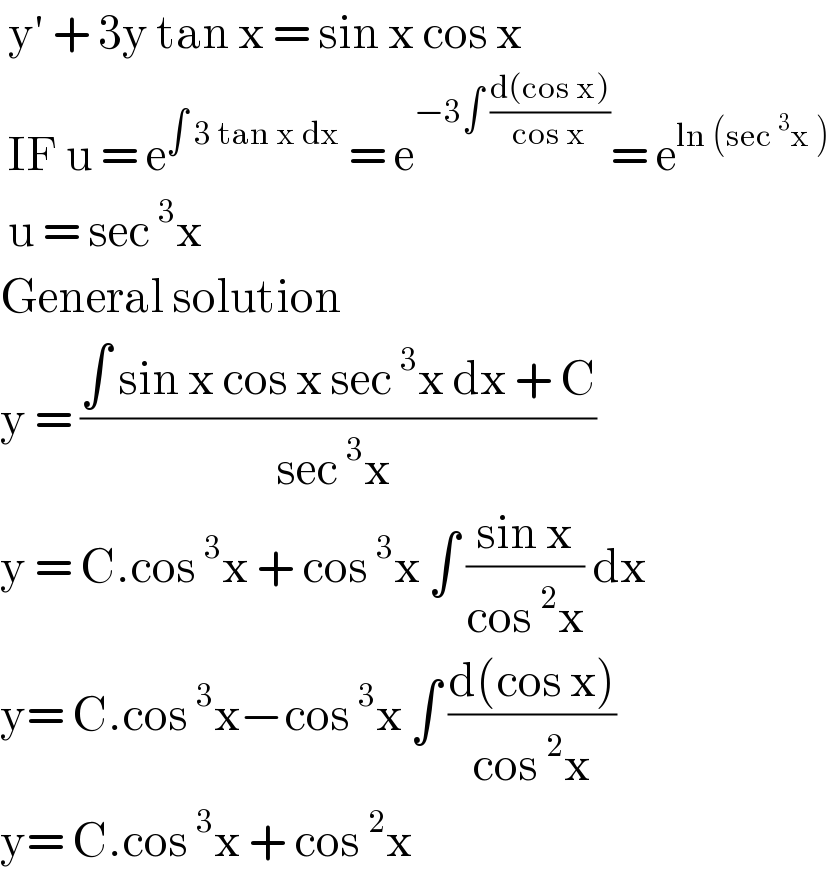
$$\:\mathrm{y}'\:+\:\mathrm{3y}\:\mathrm{tan}\:\mathrm{x}\:=\:\mathrm{sin}\:\mathrm{x}\:\mathrm{cos}\:\mathrm{x} \\ $$$$\:\mathrm{IF}\:\mathrm{u}\:=\:\mathrm{e}^{\int\:\mathrm{3}\:\mathrm{tan}\:\mathrm{x}\:\mathrm{dx}} \:=\:\mathrm{e}^{−\mathrm{3}\int\:\frac{\mathrm{d}\left(\mathrm{cos}\:\mathrm{x}\right)}{\mathrm{cos}\:\mathrm{x}}} =\:\mathrm{e}^{\mathrm{ln}\:\left(\mathrm{sec}\:^{\mathrm{3}} \mathrm{x}\:\right)} \\ $$$$\:\mathrm{u}\:=\:\mathrm{sec}\:^{\mathrm{3}} \mathrm{x}\: \\ $$$$\mathrm{General}\:\mathrm{solution}\: \\ $$$$\mathrm{y}\:=\:\frac{\int\:\mathrm{sin}\:\mathrm{x}\:\mathrm{cos}\:\mathrm{x}\:\mathrm{sec}\:^{\mathrm{3}} \mathrm{x}\:\mathrm{dx}\:+\:\mathrm{C}}{\mathrm{sec}\:^{\mathrm{3}} \mathrm{x}\:} \\ $$$$\mathrm{y}\:=\:\mathrm{C}.\mathrm{cos}\:^{\mathrm{3}} \mathrm{x}\:+\:\mathrm{cos}\:^{\mathrm{3}} \mathrm{x}\:\int\:\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{cos}\:^{\mathrm{2}} \mathrm{x}}\:\mathrm{dx} \\ $$$$\mathrm{y}=\:\mathrm{C}.\mathrm{cos}\:^{\mathrm{3}} \mathrm{x}−\mathrm{cos}\:^{\mathrm{3}} \mathrm{x}\:\int\:\frac{\mathrm{d}\left(\mathrm{cos}\:\mathrm{x}\right)}{\mathrm{cos}\:^{\mathrm{2}} \mathrm{x}} \\ $$$$\mathrm{y}=\:\mathrm{C}.\mathrm{cos}\:^{\mathrm{3}} \mathrm{x}\:+\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{x}\: \\ $$
