e-2x-1-x-1-2x-2-dx-e-2x-1-sin-2x-1-cos-2x-dx-Solve-above-Questions-by-using-the-formulae-e-kx-f-kx-f-kx-dx-e-kx-f-kx-c-

Question Number 54699 by rahul 19 last updated on 09/Feb/19

\int e^{2 x} (\frac{1}{x} - \frac{1}{2 x^{2}}) d x

\int e^{2 x} (\frac{1 + \sin 2 x}{1 + \cos 2 x}) d x .

S o l v e a b o v e Q u e s t i o n s b y u s i n g t h e

f o r m u l a e :

\int e^{k x} {f (k x) + f^{'} (k x)} d x = e^{k x} f (k x) + c .

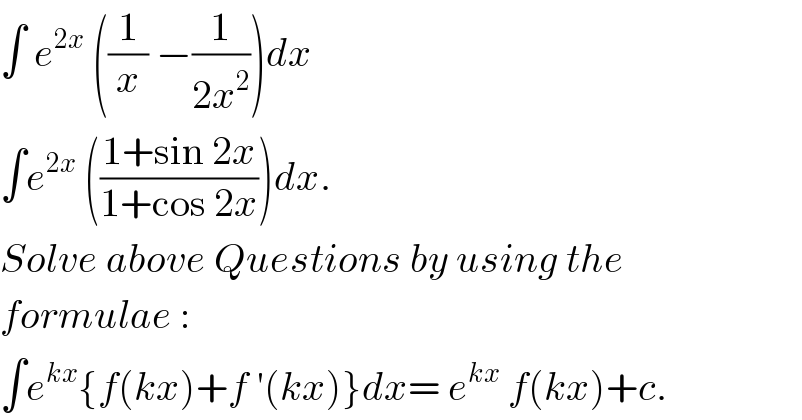
Commented by maxmathsup by imad last updated on 10/Feb/19

l e t I = \int (\frac{1}{x} - \frac{1}{2 x^{2}}) e^{2 x} d x \Rightarrow I = \int \frac{e^{2 x}}{x} d x - \int \frac{e^{2 x}}{2 x^{2}} b y p s r t s

\int \frac{e^{2 x}}{x} d x = \frac{1}{2 x} e^{2 x} - \int - \frac{1}{2 x^{2}} e^{2 x} = \frac{1}{2 x} e^{2 x} + \int \frac{e^{2 x}}{2 x^{2}} \Rightarrow

I = \frac{e^{2 x}}{2 x} + \int \frac{e^{2 x}}{2 x^{2}} - \int \frac{e^{2 x}}{2 x^{2}} d x + c \Rightarrow I = \frac{e^{2 x}}{2 x} + c .

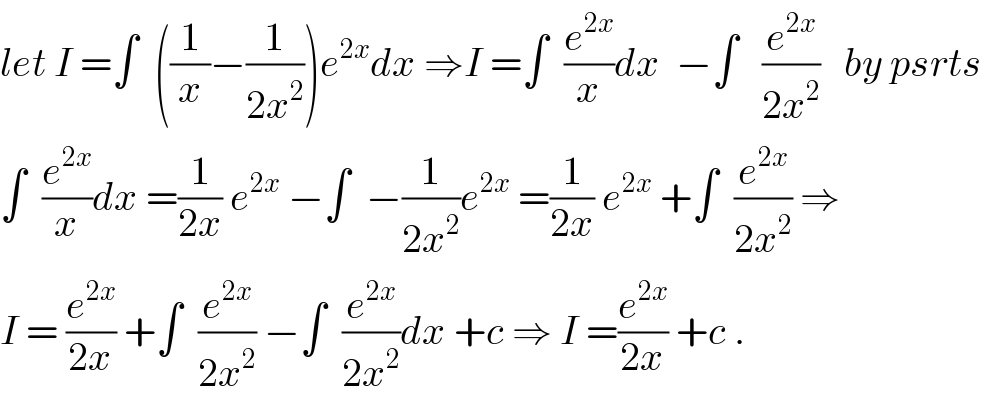
Commented by rahul 19 last updated on 10/Feb/19

thank you profAbdo .

Commented by maxmathsup by imad last updated on 11/Feb/19

y o u a r e w e l c o m e s i r .

Answered by kaivan.ahmadi last updated on 09/Feb/19

f (x) = \frac{2}{x} \Rightarrow f (2 x) = \frac{1}{x} \Rightarrow f^{'} (x) = \frac{- 2}{x^{2}} \Rightarrow

f^{'} (2 x) = \frac{- 1}{{2 x}^{2}}

\int e^{2 x} (\frac{1}{x} - \frac{1}{{2 x}^{2}}) dx = e^{2 x} f (2 x) + c = \frac{e^{2 x}}{x} + c

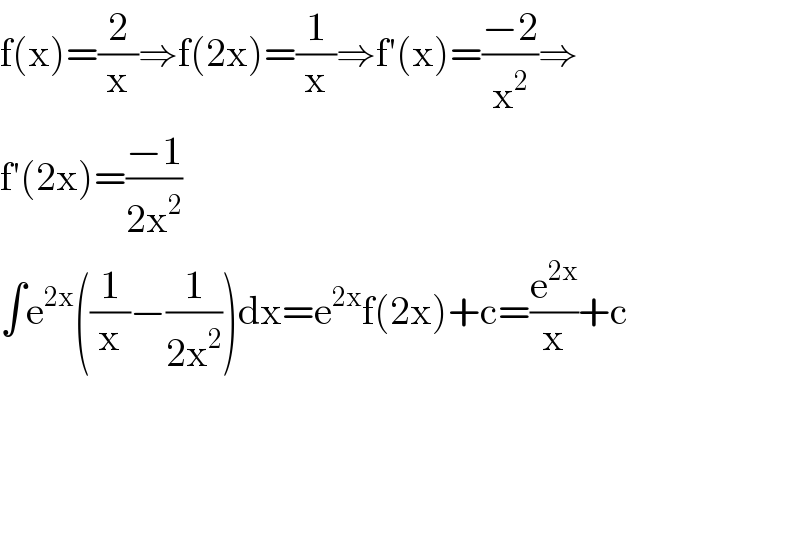
Commented by rahul 19 last updated on 09/Feb/19

S i r, i f f (2 x) = \frac{1}{x} t h e n f^{'} (2 x) s h o u l d b e

\frac{- 1}{x^{2}} ? {I s n}^{'} t i t ?

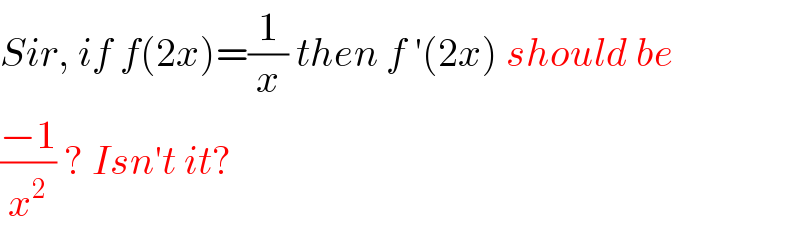
Commented by kaivan.ahmadi last updated on 09/Feb/19

yes it is, thank you . the answer of your integral is not true, because

{(e^{kx} f (kx))}^{^{'}} = {ke}^{2 x} (f (kx) + f^{'} (kx))

Answered by tanmay.chaudhury50@gmail.com last updated on 09/Feb/19

1) \frac{1}{2} \int [\frac{1}{x} \times \frac{d}{d x} (e^{2 x}) + e^{2 x} \frac{d}{d x} (\frac{1}{x})] d x

\frac{1}{2} \int \frac{d}{d x} (\frac{1}{x} e^{2 x}) d x

\frac{1}{2} (\frac{e^{2 x}}{x}) + c

2) \int e^{2 x} (\frac{1 + 2 s i n x c o s x}{2 {c o s}^{2} x})

\int e^{2 x} (\frac{1}{2} {s e c}^{2} x + t a n x) d x

\frac{1}{2} \int [e^{2 x} \times \frac{d}{d x} (t a n x) + t a n x \times \frac{d}{d x} (e^{2 x})] d x

= \frac{1}{2} \int \frac{d}{d x} (e^{2 x} t a n x) d x

\frac{e^{2 x} t a n x}{2} + c

Commented by rahul 19 last updated on 10/Feb/19

thank you sir �� but how to do by using given formulae ?