e-pis-s-2-s-2-1-find-inverse-laplace-

Question Number 129241 by BHOOPENDRA last updated on 14/Jan/21

(e^(−πs) /(s^2 (s^2 +1))) find inverse laplace

$$\frac{{e}^{−\pi{s}} }{{s}^{\mathrm{2}} \left({s}^{\mathrm{2}} +\mathrm{1}\right)}\:{find}\:{inverse}\:{laplace} \\ $$

Answered by Olaf last updated on 14/Jan/21

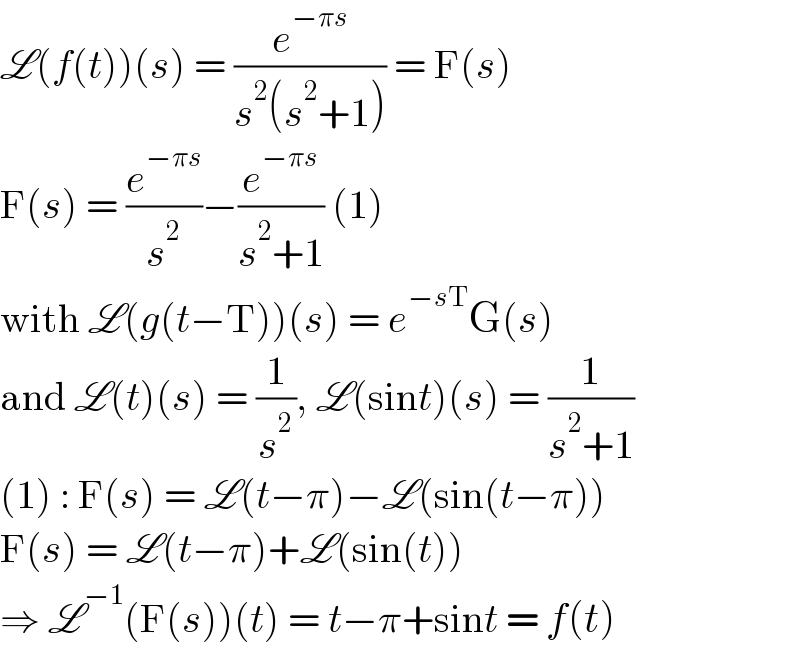
L(f(t))(s) = (e^(−πs) /(s^2 (s^2 +1))) = F(s) F(s) = (e^(−πs) /s^2 )−(e^(−πs) /(s^2 +1)) (1) with L(g(t−T))(s) = e^(−sT) G(s) and L(t)(s) = (1/s^2 ), L(sint)(s) = (1/(s^2 +1)) (1) : F(s) = L(t−π)−L(sin(t−π)) F(s) = L(t−π)+L(sin(t)) ⇒ L^(−1) (F(s))(t) = t−π+sint = f(t)

$$\mathscr{L}\left({f}\left({t}\right)\right)\left({s}\right)\:=\:\frac{{e}^{−\pi{s}} }{{s}^{\mathrm{2}} \left({s}^{\mathrm{2}} +\mathrm{1}\right)}\:=\:\mathrm{F}\left({s}\right) \\ $$$$\mathrm{F}\left({s}\right)\:=\:\frac{{e}^{−\pi{s}} }{{s}^{\mathrm{2}} }−\frac{{e}^{−\pi{s}} }{{s}^{\mathrm{2}} +\mathrm{1}}\:\left(\mathrm{1}\right) \\ $$$$\mathrm{with}\:\mathscr{L}\left({g}\left({t}−\mathrm{T}\right)\right)\left({s}\right)\:=\:{e}^{−{s}\mathrm{T}} \mathrm{G}\left({s}\right) \\ $$$$\mathrm{and}\:\mathscr{L}\left({t}\right)\left({s}\right)\:=\:\frac{\mathrm{1}}{{s}^{\mathrm{2}} },\:\mathscr{L}\left(\mathrm{sin}{t}\right)\left({s}\right)\:=\:\frac{\mathrm{1}}{{s}^{\mathrm{2}} +\mathrm{1}} \\ $$$$\left(\mathrm{1}\right)\::\:\mathrm{F}\left({s}\right)\:=\:\mathscr{L}\left({t}−\pi\right)−\mathscr{L}\left(\mathrm{sin}\left({t}−\pi\right)\right) \\ $$$$\mathrm{F}\left({s}\right)\:=\:\mathscr{L}\left({t}−\pi\right)+\mathscr{L}\left(\mathrm{sin}\left({t}\right)\right) \\ $$$$\Rightarrow\:\mathscr{L}^{−\mathrm{1}} \left(\mathrm{F}\left({s}\right)\right)\left({t}\right)\:=\:{t}−\pi+\mathrm{sin}{t}\:=\:{f}\left({t}\right) \\ $$

Commented by BHOOPENDRA last updated on 14/Jan/21

Ans is not crct can you please recheck Ans is [(t−π)+sin(t−π)]×4(t−π)

$${Ans}\:{is}\:{not}\:{crct}\:{can}\:{you}\:{please}\:{recheck} \\ $$$${Ans}\:{is}\:\left[\left({t}−\pi\right)+{sin}\left({t}−\pi\right)\right]×\mathrm{4}\left({t}−\pi\right) \\ $$

Facebook Tweet Pin

e-pis-s-2-s-2-1-find-inverse-laplace-

Leave a Reply Cancel reply