Question Number 144900 by ArielVyny last updated on 30/Jun/21
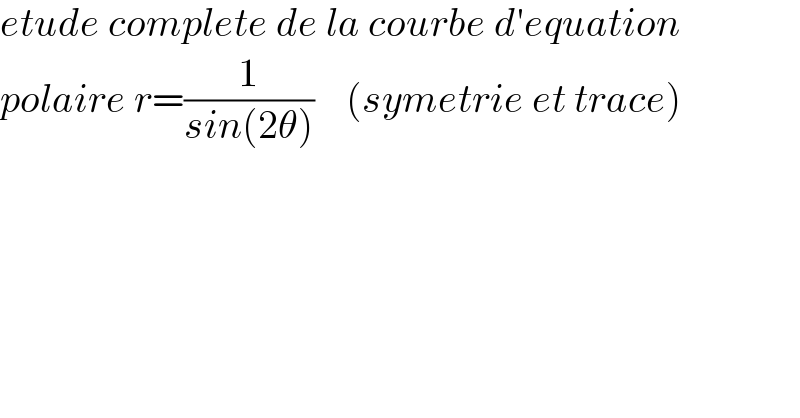
$${etude}\:{complete}\:{de}\:{la}\:{courbe}\:{d}'{equation} \\ $$$${polaire}\:{r}=\frac{\mathrm{1}}{{sin}\left(\mathrm{2}\theta\right)}\:\:\:\:\left({symetrie}\:{et}\:{trace}\right) \\ $$$$ \\ $$
Answered by qaz last updated on 30/Jun/21

$$\mathrm{2rsin}\:\theta\mathrm{cos}\:\theta=\mathrm{1} \\ $$$$\Rightarrow\mathrm{2r}^{\mathrm{2}} \mathrm{sin}\:\theta\mathrm{cos}\:\theta=\mathrm{r} \\ $$$$\Rightarrow\mathrm{2xy}=\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} } \\ $$$$\Rightarrow\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} −\mathrm{4x}^{\mathrm{2}} \mathrm{y}^{\mathrm{2}} =\mathrm{0} \\ $$
