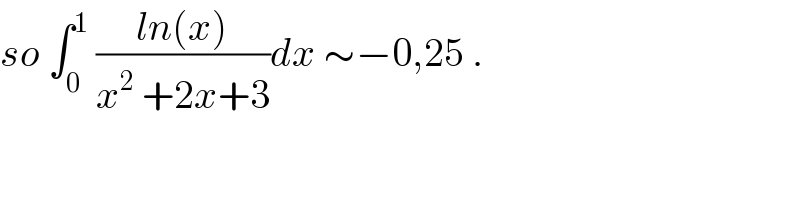Evaluate-0-1-Log-x-x-2-2x-3-dx-

Question Number 48289 by Abdulhafeez Abu qatada last updated on 21/Nov/18

E v a l u a t e \underset{0}{\int^{1}} \frac{L o g (x)}{x^{2} + 2 x + 3} d x

Commented by tanmay.chaudhury50@gmail.com last updated on 22/Nov/18

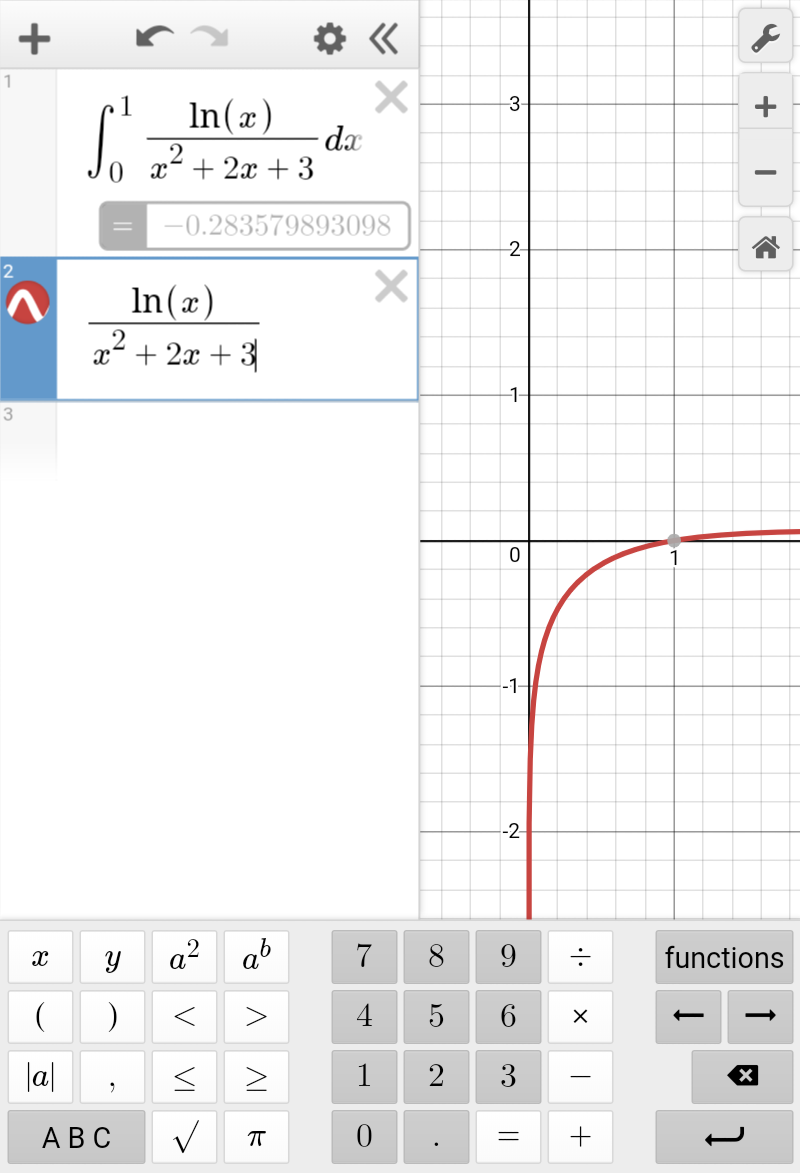
Commented by maxmathsup by imad last updated on 22/Nov/18

l e t A = \int_{0}^{1} \frac{l n (x)}{x^{2} + 2 x + 3} d x \Rightarrow A = \int_{0}^{1} \frac{l n (x)}{{(x + 1)}^{2} + 2}

A =_{x + 1 = \sqrt{2} t} \int_{\frac{1}{\sqrt{2}}}^{\sqrt{2}} \frac{l n (t \sqrt{2} - 1)}{2 (1 + t^{2})} \sqrt{2} d t = \frac{1}{\sqrt{2}} \int_{\frac{1}{\sqrt{2}}}^{\sqrt{2}} \frac{l n (t \sqrt{2} - 1)}{1 + t^{2}} d t l e t c o n s i d e r t h e

p a r a m e t r i c f u n c t i o n φ (x) = \int_{\frac{1}{\sqrt{2}}}^{\sqrt{2}} \frac{l n (x t - 1)}{1 + t^{2}} d t (x t > 1)

φ^{^{'}} (x) = \int_{\frac{1}{\sqrt{2}}}^{\sqrt{2}} \frac{t}{(x t - 1) (1 + t^{2})} d t l e t d e c o m p o s e F (t) = \frac{t}{(x t - 1) (1 + t^{2})}

F (t) = \frac{a}{x t - 1} + \frac{b t + c}{t^{2} + 1}

a = {l i m}_{t \to \frac{1}{x}} (x t - 1) F (t) = \frac{1}{x (1 + \frac{1}{x^{2}})} = \frac{1}{x + \frac{1}{x}} = \frac{x}{x^{2} + 1}

{l i m}_{t \to + \infty} t F (t) = \frac{a}{x} + b \Rightarrow b = - \frac{1}{x^{2} + 1} \Rightarrow F (t) = \frac{x}{(x^{2} + 1) (x t - 1)} + \frac{- \frac{t}{x^{2} + 1} + c}{t^{2} + 1}

F (0) = 0 = - \frac{x}{(x^{2} + 1)} + c \Rightarrow c = \frac{x}{x^{2} + 1} \Rightarrow

F (t) = \frac{x}{(x^{2} + 1) (x t - 1)} - \frac{1}{x^{2} + 1} \frac{t - x}{t^{2} + 1} \Rightarrow

φ^{^{'}} (x) = \int_{\frac{1}{\sqrt{2}}}^{\sqrt{2}} (\frac{x}{(x^{2} + 1) (x t - 1)} - \frac{1}{x^{2} + 1} \frac{t - x}{t^{2} + 1}) d t

= \frac{x}{x^{2} + 1} \int_{\frac{1}{\sqrt{2}}}^{\sqrt{2}} \frac{d t}{x t - 1} - \frac{1}{2 (x^{2} + 1)} \int_{\frac{1}{\sqrt{2}}}^{\sqrt{2}} \frac{2 t}{t^{2} + 1} + \frac{x}{x^{2} + 1} \int_{\frac{1}{\sqrt{2}}}^{\sqrt{2}} \frac{d t}{t^{2} + 1}

{= \frac{1}{x^{2} + 1} l n ∣ x t - 1 ∣]}_{\frac{1}{\sqrt{2}}}^{\sqrt{2}} - \frac{1}{2 (x^{2} + 1)} {[l n (t^{2} + 1)]}_{\frac{1}{\sqrt{2}}}^{\sqrt{2}} + \frac{x}{x^{2} + 1} {[a r c t a n (t)]}_{\frac{1}{\sqrt{2}}}^{\sqrt{2}}

= \frac{1}{x^{2} + 1} {l n ∣ x \sqrt{2} - 1 ∣ - l n ∣ \frac{x}{\sqrt{2}} - 1 ∣} - \frac{l n (2)}{2 (x^{2} + 1)}

+ \frac{x}{x^{2} + 1} {a r c t a n (\sqrt{2}) - a r c t a n (\frac{1}{\sqrt{2}})} \Rightarrow

φ (x) = \int \frac{l n (x \sqrt{2} - 1)}{x^{2} + 1} d x - \int \frac{l n (\frac{x}{\sqrt{2}} - 1)}{x^{2} + 1} d x - \frac{l n (2)}{2} \int \frac{d x}{1 + x^{2}}

+ (a r c t a n (\sqrt{2}) - a r c t a n (\frac{1}{\sqrt{2}}) \int \frac{x d x}{x^{2} + 1} + c \Rightarrow

φ (x) = \int \frac{l n (x \sqrt{2} - 1)}{x^{2} + 1} d x - \int \frac{l n (x - \sqrt{2})}{x^{2} + 1} d x + l n (\sqrt{2}) a r c t a n x - \frac{l n (2)}{2} a r c t a n x

+ \frac{1}{2} (a r c t a n (\sqrt{2}) - a r c t a n (\frac{1}{\sqrt{2}})) l n (1 + x^{2}) + c

= \int \frac{l n (x \sqrt{2} - 1)}{x^{2} + 1} d x - \int \frac{l n (x - \sqrt{2})}{x^{2} + 1} d x + \frac{1}{2} (a r c t a n (\sqrt{2}) - a r c t a n (\frac{1}{\sqrt{2}})) l n (1 + x^{2}) + c

\dots . b e c o n t i n u e d \dots

Commented by maxmathsup by imad last updated on 22/Nov/18

w e h a v e 0 < x^{2} < 1, 0 < 2 x < 2 \Rightarrow 3 < x^{2} + 2 x + 3 < 5 \Rightarrow \frac{1}{5} < \frac{1}{x^{2} + 2 x + 3} < \frac{1}{3} \Rightarrow

\Rightarrow - \frac{1}{5} l n (x) < - \frac{l n x}{x^{2} + 2 x + 3} < - \frac{1}{3} l n (x) (s e e t h a t l n (x) < 0 o n] 0, 1 [) \Rightarrow

\frac{1}{3} l n (x) < \frac{l n (x)}{x^{2} + 2 x + 3} < \frac{1}{5} l n (x) \Rightarrow \frac{1}{3} \int_{0}^{1} l n (x) d x < \int_{0}^{1} \frac{l n (x)}{x^{2} + 2 x + 3} d x < \frac{1}{5} \int_{0}^{1} l n (x) \Rightarrow

\frac{1}{3} {[x l n (x) - x]}_{0}^{1} < \int_{0}^{1} \frac{l n (x)}{x^{2} + 2 x + 3} < \frac{1}{5} {[x l n (x) - x]}_{0}^{1} \Rightarrow

- \frac{1}{3} < \int_{0}^{1} \frac{l n (x)}{x^{2} + 2 x + 3} d x < - \frac{1}{5} w e c a n t a k e \int_{0}^{1} \frac{l n (x)}{x^{2} + 2 x + 3} \sim - \frac{8}{30}

w i t h p r e c i s i o n σ = \frac{\frac{1}{3} - \frac{1}{5}}{2} = \frac{2}{30} .

Commented by maxmathsup by imad last updated on 22/Nov/18

s o \int_{0}^{1} \frac{l n (x)}{x^{2} + 2 x + 3} d x \sim - 0, 25 .