Evaluate-0-a-b-1-x-2-a-2-dx-

Question Number 118073 by bemath last updated on 15/Oct/20

Evaluate \underset{0}{\int^{a}} b \sqrt{1 - \frac{x^{2}}{a^{2}}} d x

Answered by john santu last updated on 15/Oct/20

I = \underset{0}{\int^{a}} \frac{b}{a} \sqrt{a^{2} - x^{2}} d x; t h e i n t e g r a l r e p r e s e n t s t h e a r e a

o f a q u a r t e r c i r c l e h a v i n g r a d i u s a

I = \frac{b}{a} \times \frac{1}{4} π a^{2} = \frac{π a b}{4}

Commented by bemath last updated on 15/Oct/20

gave kudos

Commented by MJS_new last updated on 15/Oct/20

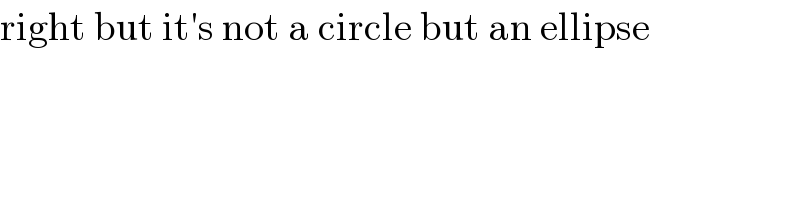
right but {it}^{'} s not a circle but an ellipse

Commented by john santu last updated on 16/Oct/20

n o s i r . i t s a c i r c l e . I f y = \sqrt{a^{2} - x^{2}}

\Rightarrow y^{2} + x^{2} = a^{2}

Commented by MJS_new last updated on 16/Oct/20

yes . but y = \frac{b}{a} \sqrt{a^{2} - x^{2}} is an ellipse

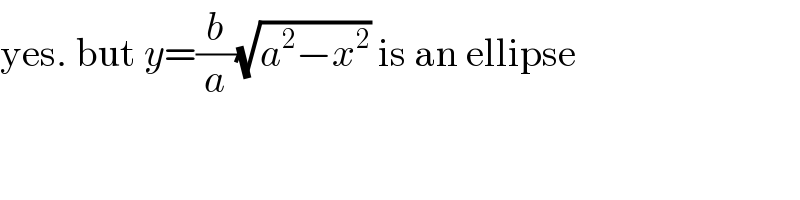
Answered by 1549442205PVT last updated on 15/Oct/20

Evaluate \underset{0}{\int^{a}} b \sqrt{1 - \frac{x^{2}}{a^{2}}} d x

Put x = acos φ (φ \in [0, π]) \Rightarrow dx = - asin φ d φ

I = - \int_{π / 2}^{0} {absin}^{2} φ d φ = \int_{0}^{π / 2} ab \frac{1 - \cos 2 φ}{2} d φ

= (\frac{ab φ}{2} - \frac{ab}{4} \sin 2 φ) ∣_{0}^{π / 2} = \frac{ab π}{4}