Evaluate-1-1-x-2-1-2-x-dx-Please-help-me-

Question Number 170519 by nimnim last updated on 25/May/22

E v a l u a t e : \int_{- 1}^{+ 1} \frac{x^{2}}{1 + 2^{x}} d x

P l e a s e h e l p m e . .

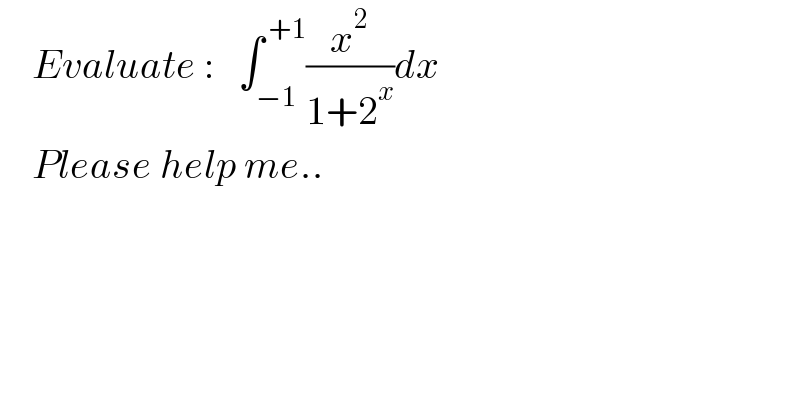
Answered by Mathspace last updated on 26/May/22

I = \int_{- 1}^{1} \frac{x^{2}}{1 + 2^{x}} d x \Rightarrow I =_{x = - t}

\int_{1}^{- 1} \frac{t^{2}}{1 + 2^{- t}} (- d t) = \int_{- 1}^{1} \frac{x^{2}}{1 + 2^{- x}} d x \Rightarrow

2 I = \int_{- 1}^{1} (\frac{1}{1 + 2^{x}} + \frac{1}{1 + 2^{- x}}) x^{2} d x

= \int_{- 1}^{1} \frac{1 + 2^{- x} + 1 + 2^{x}}{1 + 2^{- x} + 2^{x} + 1} x^{2} d x

= \int_{- 1}^{1} x^{2} d x = {[\frac{x^{3}}{3}]}_{- 1}^{1} = \frac{1}{3} + \frac{1}{3}

= \frac{2}{3}

Commented by Mathspace last updated on 26/May/22

\Rightarrow I = \frac{1}{3}

Commented by SLVR last updated on 27/May/22

n i c e s o l u t i o n

Commented by nimnim last updated on 27/May/22

T h a n k y o u S i r .

Facebook Tweet Pin